Skip to main content
PMC home page
Proceedings of the National Academy of Sciences of the United States of America logoLink to Proceedings of the National Academy of Sciences of the United States of America
. 1982 Nov;79(22):6917–6921. doi: 10.1073/pnas.79.22.6917

Birhythmicity, chaos, and other patterns of temporal self-organization in a multiply regulated biochemical system.

O Decroly, A Goldbeter
PMCID: PMC347245  PMID: 6960354

Abstract

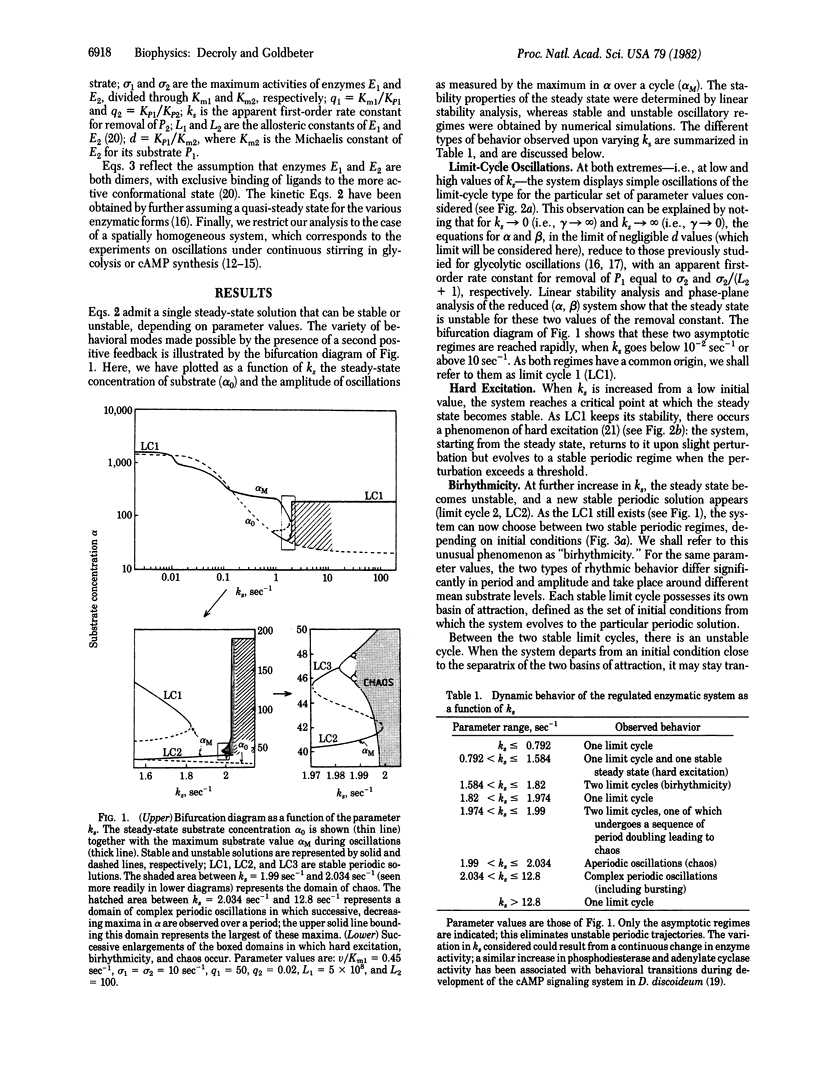
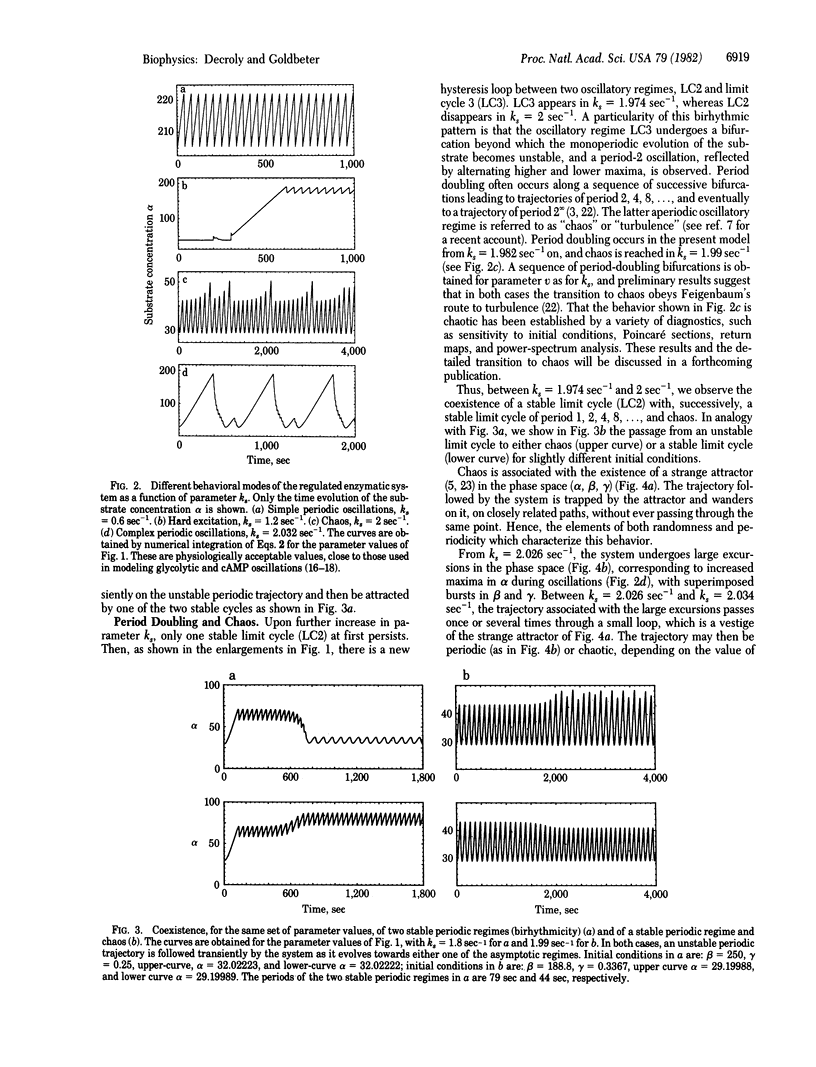
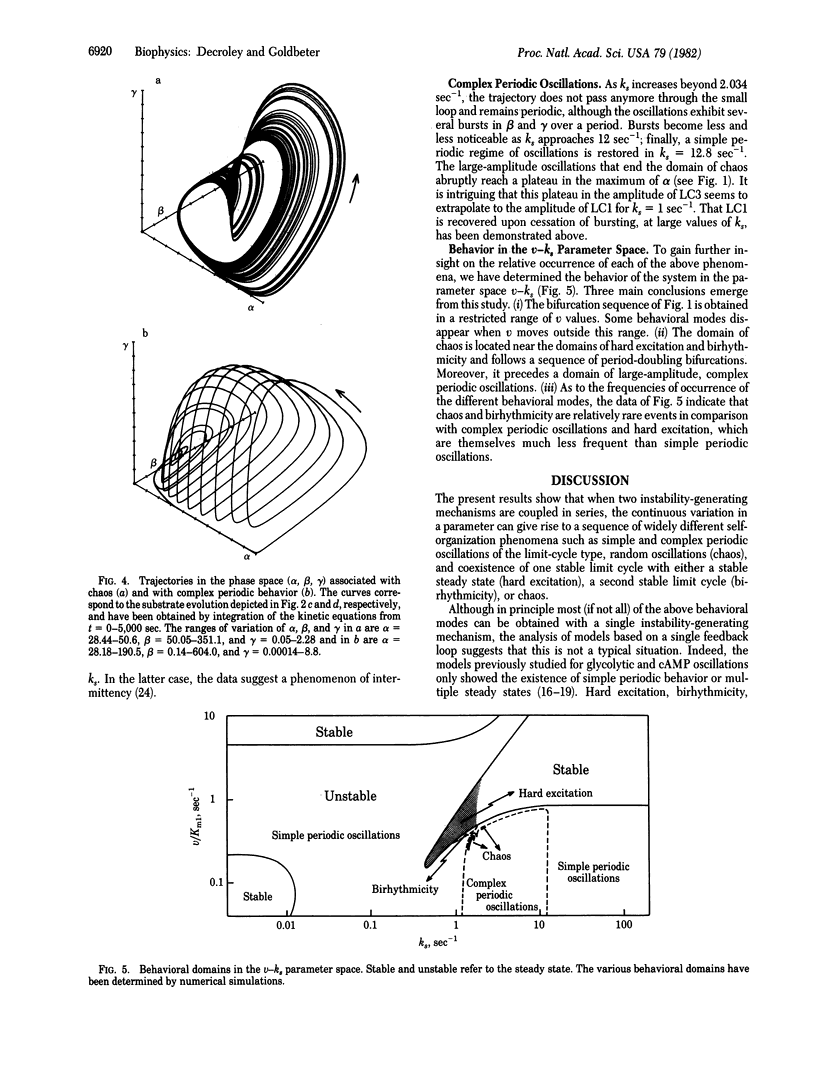
We analyze on a model biochemical system the effect of a coupling between two instability-generating mechanisms. The system considered is that of two allosteric enzymes coupled in series and activated by their respective products. In addition to simple periodic oscillations, the system can exhibit a variety of new modes of dynamic behavior; coexistence between two stable periodic regimes (birhythmicity), random oscillations (chaos), and coexistence of a stable periodic regime with a stable steady state (hard excitation) or with chaos. The relationship between these patterns of temporal self-organization is analyzed as a function of the control parameters of the model. Chaos and birhythmicity appear to be rare events in comparison with simple periodic behavior. We discuss the relevance of these results with respect to the regularity of most biological rhythms.

Full text

PDF

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Berridge M. J., Rapp P. E. A comparative survey of the function, mechanism and control of cellular oscillators. J Exp Biol. 1979 Aug;81:217–279. doi: 10.1242/jeb.81.1.217. [DOI] [PubMed] [Google Scholar]
  2. Boiteux A., Goldbeter A., Hess B. Control of oscillating glycolysis of yeast by stochastic, periodic, and steady source of substrate: a model and experimental study. Proc Natl Acad Sci U S A. 1975 Oct;72(10):3829–3833. doi: 10.1073/pnas.72.10.3829. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Degn H. Bistability caused by substrate inhibition of peroxidase in an open reaction system. Nature. 1968 Mar 16;217(5133):1047–1050. doi: 10.1038/2171047b0. [DOI] [PubMed] [Google Scholar]
  4. Frenkel R. Control of reduced diphosphopyridine nucleotide oscillations in beef heart extracts. I. Effects of modifiers of phosphofructokinase activity. Arch Biochem Biophys. 1968 Apr;125(1):151–156. doi: 10.1016/0003-9861(68)90649-8. [DOI] [PubMed] [Google Scholar]
  5. Gerisch G., Wick U. Intracellular oscillations and release of cyclic AMP from Dictyostelium cells. Biochem Biophys Res Commun. 1975 Jul 8;65(1):364–370. doi: 10.1016/s0006-291x(75)80102-1. [DOI] [PubMed] [Google Scholar]
  6. Glass L., Mackey M. C. Pathological conditions resulting from instabilities in physiological control systems. Ann N Y Acad Sci. 1979;316:214–235. doi: 10.1111/j.1749-6632.1979.tb29471.x. [DOI] [PubMed] [Google Scholar]
  7. Goldbeter A., Caplan S. R. Oscillatory enzymes. Annu Rev Biophys Bioeng. 1976;5:449–476. doi: 10.1146/annurev.bb.05.060176.002313. [DOI] [PubMed] [Google Scholar]
  8. Goldbeter A., Lefever R. Dissipative structures for an allosteric model. Application to glycolytic oscillations. Biophys J. 1972 Oct;12(10):1302–1315. doi: 10.1016/S0006-3495(72)86164-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Goldbeter A., Segel L. A. Control of developmental transitions in the cyclic AMP signalling system of Dictyostelium discoideum. Differentiation. 1980;17(3):127–135. doi: 10.1111/j.1432-0436.1980.tb01090.x. [DOI] [PubMed] [Google Scholar]
  10. Goldbeter A., Segel L. A. Unified mechanism for relay and oscillation of cyclic AMP in Dictyostelium discoideum. Proc Natl Acad Sci U S A. 1977 Apr;74(4):1543–1547. doi: 10.1073/pnas.74.4.1543. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Hess B., Boiteux A. Oscillatory phenomena in biochemistry. Annu Rev Biochem. 1971;40:237–258. doi: 10.1146/annurev.bi.40.070171.001321. [DOI] [PubMed] [Google Scholar]
  12. MONOD J., WYMAN J., CHANGEUX J. P. ON THE NATURE OF ALLOSTERIC TRANSITIONS: A PLAUSIBLE MODEL. J Mol Biol. 1965 May;12:88–118. doi: 10.1016/s0022-2836(65)80285-6. [DOI] [PubMed] [Google Scholar]
  13. May R. M. Simple mathematical models with very complicated dynamics. Nature. 1976 Jun 10;261(5560):459–467. doi: 10.1038/261459a0. [DOI] [PubMed] [Google Scholar]
  14. Olsen L. F., Degn H. Chaos in an enzyme reaction. Nature. 1977 May 12;267(5607):177–178. doi: 10.1038/267177a0. [DOI] [PubMed] [Google Scholar]

Articles from Proceedings of the National Academy of Sciences of the United States of America are provided here courtesy of National Academy of Sciences

RESOURCES