Figure 4.

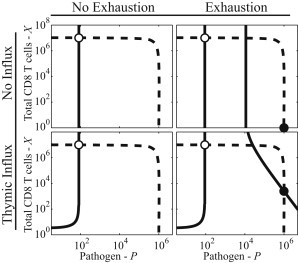
Comparison of four limits of the model: no influx and no exhaustion (top left), exhaustion but no influx (top right), influx but no exhaustion (bottom left), and the full model (bottom right). Each subplot shows the nullcline for Eq. 4 (dashed) and the total T cells at equilibrium given by Eq. 20 (solid). Stationary points exist at the intersections of the curves and stable fixed points are annotated (circle). The systems without exhaustion have no persistent state, only a stable fixed point that acute infections orbit around (open circles). The orbits of acute infections drop to values corresponding to pathogen clearance and do not typically converge to these points. The plots illustrate the requirement of exhaustion and constraints on pathogen growth (resource limitations and innate immunity) in the existence of the second stable point (solid circles). We also see the requirement of thymic influx in maintaining a population of T cells during the infection. Without resource limitations or innate immune mechanisms, the dashed curve would be a horizontal line with no persistent state.