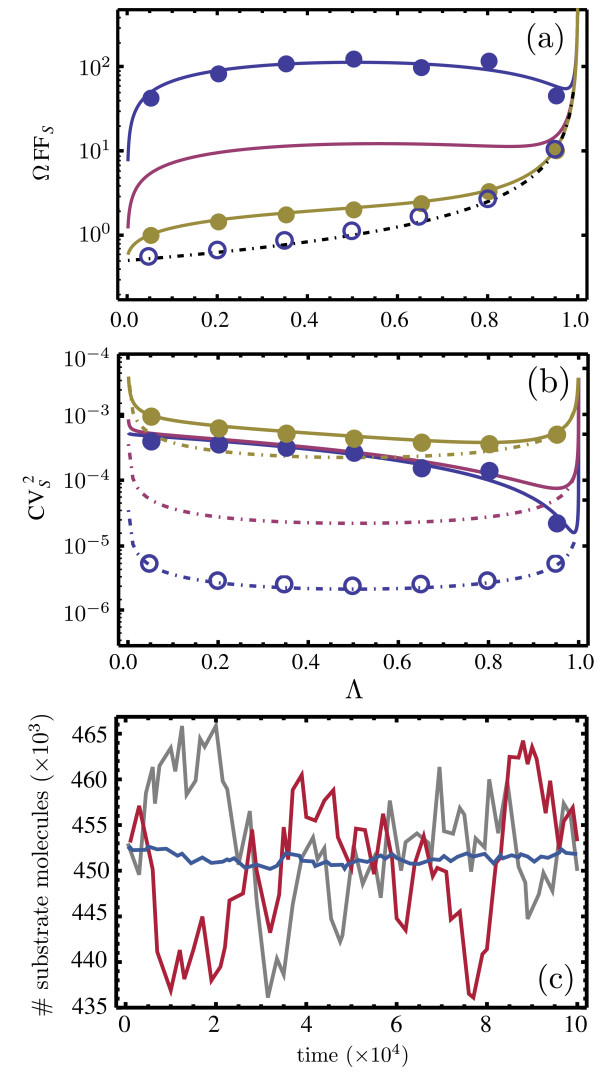
Figure 4.

Noise statistics for cooperative two-subunit enzyme network. Plots showing the Fano factor multiplied by the volume, ΩFF_S, (a) and the coefficient of variation squared, ${\text{CV}}_{\text{S}}^2$, (b) for the substrate fluctuations as a function of the non-dimensional fraction Θ, in steady-state conditions. The latter is a measure of enzyme saturation. The solid lines are the ssLNA predictions, the dashed lines are the hLNA predictions, the solid circles are obtained from stochastic simulations of the full network and the open circles are obtained from stochastic simulations of the coarse-grained network using the SSA with heuristic propensities. The color coding indicates different values of the bimolecular rate constant k₁: 5×10⁻³(yellow), 5×10⁻⁵(purple), and 5×10⁻⁷(blue). The remaining parameters are given by $\left[E{E}_T\right]=1$, k₂=1000, k₋₁=k₋₂=100, k₃=k₄=1. Note that in (a) the black dashed line indicates the hLNA prediction for all three different values of k₁, which are indistinguishable in this figure. The stochastic simulations were carried out for a volume Ω=100. In (c) sample paths of the SSA for the full network (gray), the slow scale Langevin equation (red) as given by equation (18) and the SSA with heuristic propensities (blue) are compared for Θ=0.5. The slow scale Langevin equation is numerically solved using the Euler-Maruyama method with timestep δt=0.1. Note that in all cases, the chosen parameters guarantee timescale separation (validity of the deterministic QSSA) (see Figure 5)