Are External Knee Load and EMG Measures Accurate Indicators of Internal Knee Contact Forces during Gait?

Abstract

Mechanical loading is believed to be a critical factor in the development and treatment of knee osteoarthritis. However, the contact forces to which the knee articular surfaces are subjected during daily activities cannot be measured clinically. Thus, the ability to predict internal knee contact forces accurately using external measures (i.e., external knee loads and muscle EMG signals) would be clinically valuable. This study quantifies how well external knee load and EMG measures predict internal knee contact forces during gait. A single subject with a force-measuring tibial prosthesis and post-operative valgus alignment performed four gait patterns (normal, medial thrust, walking pole, and trunk sway) to induce a wide range of external and internal knee joint loads. Linear regression analyses were performed to assess how much of the variability in internal contact forces was accounted for by variability in the external measures. Though the different gait patterns successfully induced significant changes in the external and internal quantities, changes in external measures were generally weak indicators of changes in total, medial, and lateral contact force. Our results suggest that when total contact force may be changing, caution should be exercised when inferring changes in knee contact forces based on observed changes in external knee load and EMG measures. Advances in musculoskeletal modeling methods may be needed for accurate estimation of in vivo knee contact forces.

INTRODUCTION

Researchers project that 25% of adults in the United States will have osteoarthritis (OA) by the year 2030,¹ with knee OA in particular currently afflicting over 37% of adults over age 60.² Excessive or abnormal mechanical loading has been implicated as a key contributing factor to the initiation and progression of knee OA.^3–6 Such load alterations can be caused by factors such as varus or valgus malalignment,^7–9 meniscal injury,¹⁰ or anterior cruciate ligament rupture.¹¹ Because of the strong association between load alterations and disease development, researchers have sought ways to modify knee contact forces through interventions such as high tibial osteotomy,^12–14 gait modification,^15–20 medial unloader knee braces,^21,22 and shoe modifications.^23–25 However, measurement of changes in knee contact forces resulting from any particular intervention is currently infeasible in a clinical setting.²⁶

To address this limitation, researchers have used external knee loads to estimate internal knee contact forces.^{3–6,15–20,22–25,27–36} These external loads are due to the external forces and moments acting on the foot and shank and are the negative of the internal knee loads calculated by inverse dynamics. Two external knee loads in particular have been proposed as indicators of internal knee contact force. The external knee superior force directed along the shaft of the tibia has been proposed as an indicator of total knee contact force,^{6,17,18,32–36} while the external knee adduction moment directed about an anterior-posterior axis fixed in the tibia has been proposed as an indicator of medial-lateral contact force distribution.^{3–5,15,16,19,20,22–25,27–31,36} Based on the implicit assumption that total contact force does not change, the knee adduction moment is often treated as an indicator of absolute medial contact force as well. The main advantage of using these external loads as indicators of internal knee contact force is that they are relatively easy to calculate, and the external knee adduction moment in particular has been shown to correlate well with medial contact force in a subject implanted with a force-measuring tibial prosthesis.^25,37

An important limitation of these external measures is that they do not account for all factors that contribute to internal knee contact forces. From an anatomical perspective, the net external knee force F_ext is balanced by a net internal knee force F_int calculated by inverse dynamics, which in turn is caused by forces from muscles F_musc, articular contact F_cont, and ligaments F_lig :

$$\begin{array}{cc}\hfill & {\mathbf{F}}_{ext}+{\mathbf{F}}_{int}=0\hfill \\ \hfill & {\mathbf{F}}_{int}={\mathbf{F}}_{musc}+{\mathbf{F}}_{cont}+{\mathbf{F}}_{lig}\hfill \end{array}$$ (1)

Equation (1) contains vector expressions, indicating that both magnitude and direction of each type of force must be considered. When the goal is to estimate knee contact forces, ligament forces are often assumed to be negligible, and Eq. (1) can be solved for the contact forces in terms of the net external force and muscle forces:

$${\mathbf{F}}_{cont}=-({\mathbf{F}}_{ext}+{\mathbf{F}}_{musc})$$ (2)

Equation (2) indicates that the magnitude of the contact forces acting on the tibia will increase when either external forces are increased or muscle contraction is increased. Consequently, if muscle forces have a significant contribution to knee contact forces, one might expect that external forces alone would be insufficient to estimate internal knee contact forces accurately.

The challenge of Eq. (2) then becomes how to estimate muscle contributions to knee contact forces. From the standpoint of external measures, muscle electromyographic (EMG) signals are the primary quantities available that relate to muscle force. While these signals reflect the commands sent to the muscles by the central nervous system, they are not direct indicators of muscle force due to the complexities of activation dynamics and muscle force-length-velocity properties.³⁸ Nonetheless, if these external measures were shown to be highly correlated with knee contact force, this finding would diminish the need for complicated modeling methods to estimate knee contact forces.

The goal of this study was to quantify the relationship between two types of external measures – knee loads and muscle EMG signals – and internal knee contact forces during gait. In vivo knee contact force data (medial, lateral, and total) were collected from a single subject implanted with a force-measuring tibial prosthesis. The subject performed a variety of gait patterns to generate wide variations in the external measures and internal contact forces, and these data were used in a series of linear regression analyses. The regression results provide a useful evaluation of the extent to which changes in external measures may or may not reflect changes in internal contact forces in the knee during gait.

METHODS

Experimental data collection

A single subject implanted with a cruciate retaining force-measuring tibial prosthesis (male, right knee, age 83 yr, mass 70 kg, height 1.70 m, valgus leg alignment 6.5°) performed four gait patterns with simultaneous collection of internal knee contact force/moment data and external motion capture (Motion Analysis Corporation, Santa Rosa, CA), ground reaction (AMTI Corporation, Watertown, MA), and muscle EMG (Delsys Corporation, Boston, MA) data. Institutional review board approval and subject informed consent were obtained. The implant was instrumented with a six-axis load cell (i.e., three forces and three moments) in the stem of the tibial tray, with load cell measurements being telemetered via a micro-transmitter and antenna.³⁹ Thus, the instrumented tibial prosthesis did not provide medial and lateral contact forces directly. To reduce measurement noise, implant load cell data were low pass filtered at 6 Hz using a 5^th degree Woltring filter.⁴⁰ Motion capture data were collected using a modified Cleveland Clinic marker set with additional markers placed on the feet.⁴¹ Muscle EMG data were collected from 14 lower limb muscles using surface electrodes, including the following seven muscles spanning the knee: biceps femoris longhead, vastus medialis, vastus lateralis, rectus femoris, medial gastrocnemius, lateral gastrocnemius, and tensor fascia latae. All experimental data used in this study are from the second Grand Challenge Competition to Predict In Vivo Knee Loads and are freely available from https://simtk.org/home/kneeloads.²⁶ Note that Walter et al. (2010)⁴² studied a different subject from the first competition.

To achieve wide variations in external measures and internal contact forces, the subject performed four different overground gait patterns. The first was his normal gait pattern while the remaining three (medial thrust, walking pole, and trunk sway) were gait patterns believed to alter medial contact force significantly.^15,16,20,43 Medial thrust gait involved knee medialization during stance phase, the goal being to reduce the moment arm of the ground reaction force vector about the knee center. Walking pole gait involved the use of bilateral hiking poles, where the ipsilateral pole was placed on the ground opposite the contralateral stance phase heel to bear a portion of body weight and counteract the stance leg knee adduction moment. Trunk sway gait tilted the trunk bilaterally over the stance leg knee, ideally causing the line of action of the ground reaction force vector to shift laterally due to the lateral shift in the center of mass. For each gait pattern, five trials with clean force plate strikes were obtained for analysis. All gait trials were performed at the subject's self-selected speed of 1.2 m/s, with no statistically significant differences in speed occurring between the different gait patterns.

Experimental data analysis

Internal medial and lateral contact forces were calculated from the implant load cell measurements using a deformable contact model. The model was constructed from CAD geometry of the subject's implant components. The tibial insert and tray possessed six degrees of freedom (DOFs) relative to a fixed femoral component, and elastic foundation theory was used to allow the rigid femoral component to penetrate the deformable tibial insert.⁴⁴ An optimization procedure determined the five DOF pose of the tibial insert/tray on the femoral component that reproduced the implant load cell measurements given the fixed flexion angle found with the subject's inverse dynamics model (described below). The optimization procedure was performed for each time frame to calculate medial and lateral contact forces for all gait trials. Details on linear regression models that permit calculation of medial and lateral contact force directly from the subject's implant load cell measurements without the need for a deformable contact model are provided as Supplementary Material.

Two types of external measures were calculated for regression with internal knee contact force measurements. The first type was external knee loads, which were defined as the negative of internal inverse dynamic knee loads calculated using previously published methods.⁴¹ In brief, a subject-specific full leg dynamic model consisting of foot, shank, and thigh segments was constructed using symbol manipulation software (Autolev™, OnLine Dynamics, Sunnyvale, CA) where the knee was treated as an idealized hinge joint. Segment coordinate systems were created using marker locations measured during a static trial with the subject's feet pointing forward. The shank superior axis was directed from the midpoint of the static medial and lateral ankle markers to the midpoint of the static medial and lateral knee markers. The shank anterior axis was defined to be in line with the long axis of the foot in the static pose. The location of the knee center in the shank and thigh segments was defined as the midpoint of the static medial and lateral knee markers. Marker motion (foot and shank only) and ground reaction data from five trials of each gait pattern were input into the model to calculate inverse dynamic loads at the knee. For the regression analyses, the three associated external knee loads of interest were the superior force F_sup directed along the shank superior-inferior axis, the adduction moment M_add calculated about the knee center using the shank anterior-posterior axis, and the flexion moment M_flex calculated about the knee center using the shank medial-lateral axis, where M_add and M_flex were mutually perpendicular. To assist with determination of medial and lateral contact forces as described above, the knee flexion angle was also calculated using marker motion data from the shank and thigh segments.

The second type of external measure was muscle EMG envelopes. These envelopes were calculated by following published EMG processing procedures.⁴⁵ Specifically, raw EMG data were high pass filtered at 30 Hz using a 4^th order zero phase lag Butterworth filter, demeaned, rectified, low pass filtered at 6 Hz using the same Butterworth filter, and finally normalized to the maximum value over all trials including maximum voluntary contraction trials.⁴⁶ Post-hoc analyses found that subsequent regression results were insensitive to EMG normalization procedures.

For the regression analyses, peak values were identified for both types of external measures in the region of the first and second peak in medial, lateral, and total contact force. For each of the three external knee loads, a peak value was determined within a time window of 100 ms before and after the first (near 25% of stance phase) and second (near 75% of stance phase) peak in medial, lateral, and total contact force. For two trials, a wider window (140 and 110 ms, respectively) was used before the first contact force peak to ensure that the true peak external load value was captured. For the flexion moment, the absolute value of each peak was used.⁴² Similarly, for each muscle EMG envelope, the maximum value was found within a time window of 40 to 60 ms before the first and second peak in medial, lateral, and total contact force. Thus, for each gait trial, we recorded up to six separate peak values for each external knee load and muscle EMG envelope, where each value corresponded to a peak in medial, lateral, or total contact force. However, only two peaks were needed for each external knee load, since the same peaks were identified within the time windows for each contact force peak.

Statistical analysis

We performed two sets of statistical tests on the internal and external experimental data. The first set evaluated whether the four gait patterns achieved significant variations in internal contact forces and external knee load and EMG measures. This evaluation was performed using a series of Kruskal-Wallis tests (p < 0.05), where gait pattern was the independent variable and first or second peak in contact force (medial, lateral, or total), external knee load (superior, adduction, or flexion), or EMG envelope (each of 7 muscles using values corresponding to total contact force peaks) was the dependent variable. When a significant difference between gait patterns was found, Tukey's honestly significant difference test was used to determine the gait patterns for which the variable differed from normal gait.

The second set of statistical tests evaluated the extent to which the external measures were predictive of the internal contact forces. This evaluation was performed using two different linear regression analyses, each of which fitted the first peak, the second peak, or both peaks together of medial, lateral, or total contact force. The first linear regression analysis used peak values of the three selected external knee loads (F_sup only; F_sup and M_add ; or F_sup, M_add, and |M_flex|) as the independent variables. M_add peaks were excluded from the total contact force regressions since engineering mechanics suggests that this moment should only alter the medial-lateral load distribution.³

The second linear regression analysis was identical to the first one except that peak values of |M_flex| were replaced with peak values of muscle EMG envelopes. This decision was based on the assumption that muscle EMG values provide redundant information to the knee flexion moment, which is caused primarily by muscles. Which muscle EMG peaks to include in each regression analysis was determined in a step-wise fashion.⁴⁷ All EMG peaks were initially included, and the one peak with the largest p value was removed. This process was repeated until all remaining EMG peaks had a p value of less than 0.05. The final regression models included only those EMG peaks whose coefficients were statistically significant during the step-wise regression process.

RESULTS

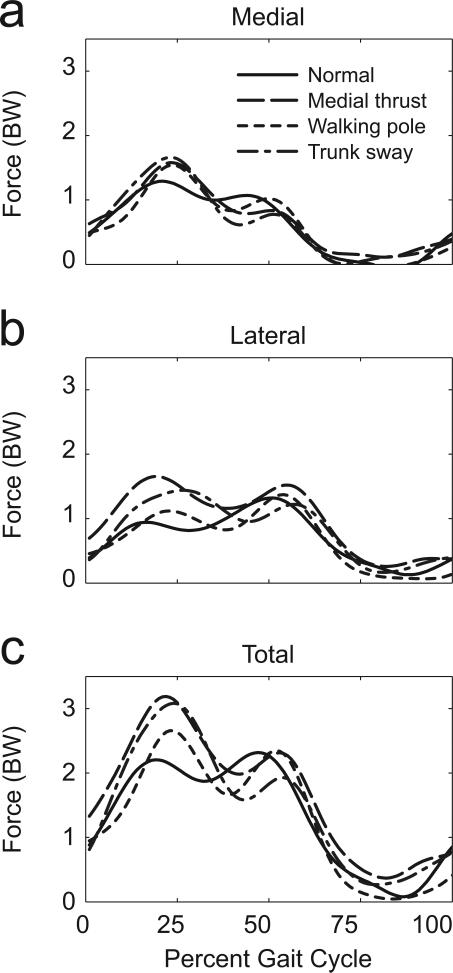
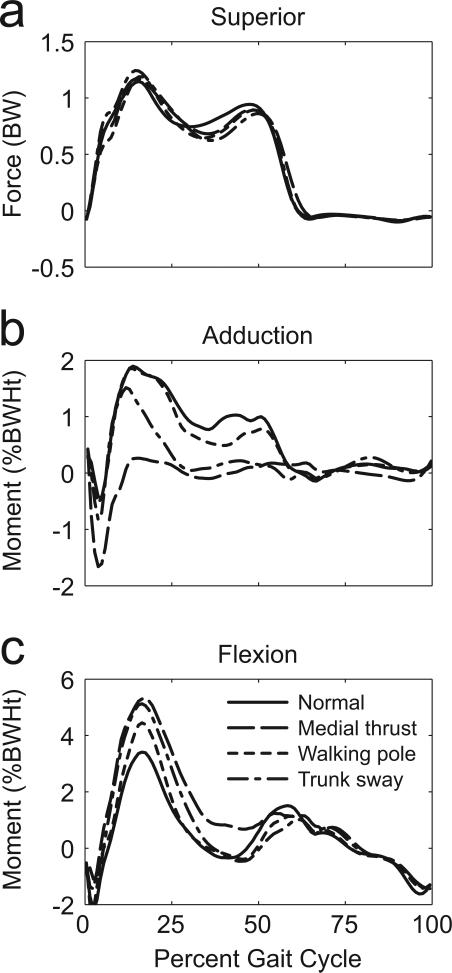
The four experimental gait patterns achieved significant variations in internal contact forces and external knee loads but not in external EMG envelopes (Figs. 1-2). Compared to normal gait, all three modified gait patterns significantly increased the first peak of total, medial, and lateral contact force during stance phase (Fig. 1, Table 1-Top). Medial thrust and walking pole gait also increased the second peak of lateral contact force, while trunk sway gait significantly decreased the second peak of medial contact force. Both peaks of the external knee adduction moment were significantly reduced for medial thrust and trunk sway gait compared to normal gait (Fig. 2, Table 1- middle). External knee superior force peaks were similar across the four gait patterns. For the external knee flexion moment, medial thrust and trunk sway gait had significantly higher first peaks compared to normal gait, while no differences were found between gait patterns for the second peak. Apart from values corresponding to the second peak in total contact force for trunk sway gait, EMG values showed few significant differences relative to normal gait.

Figure 1.

Mean instrumented implant curves for a) medial contact force, b) lateral contact force, and c) the total contact force for normal, medial thrust, walking pole, and trunk sway gait.

Figure 2.

Mean external knee load curves for a) superior force, b) adduction moment, and c) flexion moment for normal, medial thrust, walking pole, and trunk sway gait.

Table 1.

Mean (standard deviation) of internal contact forces, external knee loads, and external EMG values corresponding to first and second peaks of total contact force. Statistically significant differences relative to normal gait based on a Kruskal-Wallis test (p < 0.05) are indicated in bold text.

		Normal Gait		Medial Thrust Gait		Walking Pole Gait		Trunk Sway Gait
Quantity	Type	Peak 1	Peak 2	Peak 1	Peak 2	Peak 1	Peak 2	Peak 1	Peak 2
Contact Force (BW)	Medial force	1.33 (0.14)	1.09 (0.09)	1.61 (0.17)	0.85 (0.22)	1.55 (0.15)	1.04 (0.08)	1.67 (0.19)	0.79 (0.19)
	Lateral force	1.01 (0.14)	1.33 (0.13)	1.67 (0.12)	1.53 (0.11)	1.18 (0.13)	1.39 (0.07)	1.50 (0.18)	1.24 (0.22)
	Total force	2.30 (0.27)	2.35 (0.14)	3.20 (0.22)	2.33 (0.30)	2.70 (0.22)	2.37 (0.16)	3.09 (0.45)	1.97 (0.26)
Net Load (BW or %BWHt)	Superior force	1.14 (0.01)	0.94 (0.04)	1.16 (0.02)	0.89 (0.02)	1.19 (0.02)	0.89 (0.05)	1.24 (0.10)	0.86 (0.08)
	Adduction moment	1.92 (0.06)	1.13 (0.37)	0.35 (0.23)	0.39 (0.15)	1.93 (0.13)	0.88 (0.20)	1.57 (0.32)	0.32 (0.25)
	Flexion moment	3.21 (0.49)	-0.60 (0.25)	5.06 (0.54)	0.45 (0.41)	4.78 (0.53)	-0.26 (0.35)	5.48 (0.42)	-0.32 (0.40)
EMG Signal (Normalized)	Biceps femoris	0.38 (0.15)	0.05 (0.04)	0.20 (0.10)	0.05 (0.04)	0.13 (0.03)	0.02 (0.00)	0.20 (0.11)	0.02 (0.01)
	Vastus medialis	0.27 (0.09)	0.03 (0.02)	0.39 (0.11)	0.02 (0.01)	0.28 (0.12)	0.02 (0.01)	0.37 (0.12)	0.02 (0.02)
	Vastus lateralis	0.53 (0.15)	0.15 (0.08)	0.49 (0.12)	0.08 (0.08)	0.41 (0.10)	0.04 (0.02)	0.46 (0.09)	0.06 (0.05)
	Rectus femoris	0.12 (0.02)	0.14 (0.03)	0.11 (0.03)	0.05 (0.02)	0.07 (0.02)	0.05 (0.04)	0.09 (0.01)	0.03 (0.01)
	Medial gastroc	0.17 (0.13)	0.40 (0.04)	0.24 (0.07)	0.31 (0.12)	0.36 (0.10)	0.31 (0.14)	0.24 (0.12)	0.24 (0.19)
	Lateral gastroc	0.14 (0.12)	0.37 (0.13)	0.09 (0.03)	0.18 (0.03)	0.11 (0.03)	0.24 (0.10)	0.09 (0.03)	0.11 (0.06)
	Tensor fasciae latae	0.21 (0.09)	0.40 (0.14)	0.19 (0.08)	0.09 (0.03)	0.18 (0.03)	0.18 (0.05)	0.19 (0.06)	0.11 (0.05)

Peak values of external measures were generally weak indicators of peak values in total, medial, and lateral contact force (Table 2), with only 7 out of 25 regression models accounting for greater than 50% of the variability in peak contact force measures. For total contact force, the best R² value of 0.58 was achieved when both peaks were analyzed together and muscle EMG values were used in place of |M_flex|. For medial contact force, the best R² value of 0.84 was achieved under similar conditions. In contrast, for lateral contact force, the best R² value of 0.82 was achieved when only the first peak was analyzed using all three external knee loads. For this subject, the first peak in total and medial contact force, along with the second peak in total, medial, and lateral contact force were all poorly predicted by external measures, with the largest R² value being less than 0.48. Adding |M_flex| to the regression equations for total, medial, and lateral contact force had only a small effect on R² values and RMS errors. Muscle EMG peaks made significant contributions to the regression equations in three instances: when both peaks were analyzed for medial and lateral contact force and when the second peak was analyzed for lateral contact force. When EMG peaks replaced |M_flex| peaks, small improvements in R² values and RMS errors were observed.

Table 2.

Regression equations with corresponding R² values and RMS errors for fitting contact forces as a function of external measures. External knee loads are F_sup for superior force, M_add for adduction moment, and M_flex for flexion moment. Muscle EMG values are E_bifem, E_mvasti, E_lvasti,, and E_tfl for biceps femoris, vastus medialis, vastus lateralis, and tensor fasciae latae, respectively. All peak values used for regression analysis are available in a Microsoft Excel file provided in the Supplementary Material.

Peak(s)	Contact Force	Regression Equation	R2	RMS (BW)
First Peak	Total	c1FSI + c2	0.351	0.381
		c1FSI + c2MFE + c3	0.575	0.317
	Medial	c1FSI + c2	0.380	0.163
		c1FSI + c2MAA + c3	0.475	0.154
		c1FSI + c2MAA + c3MFE + c4	0.480	0.158
	Lateral	c1FSI + c2	0.175	0.280
		c1FSI + c2MAA + c3	0.797	0.142
		c1FSI + c2MAA + c3MFE + c4	0.822	0.137
Second Peak	Total	c1FSI + c2	0.068	0.267
		c1FSI + c2MFE + c3	0.069	0.274
	Medial	c1FSI + c2	0.162	0.184
		c1FSI + c2MAA + c3	0.417	0.157
		c1FSI + c2MAA + c3MFE + c4	0.418	0.162
	Lateral	c1FSI + c2	0.002	0.175
		c1FSI + c2MAA + c3	0.006	0.180
		c1FSI + c2MAA + c3MFE + c4	0.007	0.185
		c1FSI + c2MAA + c3EMVasti + c4ELVasti + c5	0.309	0.159
Both Peaks	Total	c1FSI + c2	0.495	0.338
		c1FSI + c2MFE + c3	0.524	0.332
		c1FSI + c2EMVasti + c3EBiFem + c4	0.603	0.308
	Medial	c1FSI + c2	0.783	0.171
		c1FSI + c2MAA + c3	0.783	0.173
		c1FSI + c2MAA + c3MFE + c4	0.791	0.171
		c1FSI + c2MAA + c3EMVasti + c4ETFL + c5	0.841	0.152
	Lateral	c1FSI + c2	0.000	0.244
		c1FSI + c2MAA + c3	0.418	0.189
		c1FSI + c2MAA + c3MFE + c4	0.418	0.189
		c1FSI + c2MAA + c3EBicFem + c4	0.482	0.180

DISCUSSION

This study used linear regression to investigate whether changes in external knee load and EMG measures are indicative of corresponding changes in internal knee contact forces during different gait motions. Experimental gait data collected from a single subject implanted with a force-measuring tibial prosthesis were used to perform the regression analyses. Overall for this subject, external measures were weak indicators of changes in internal total, medial, and lateral contact forces in the knee. Adding EMG measures to the external knee load measures produced little additional improvement. Our results for this subject suggest that inferring knee contact force changes from changes in external measures may not work well in situations where muscle forces or total contact force may be changing. For accurate patient-specific estimation of in vivo knee contact forces, validated musculoskeletal modeling methods may be needed that account for the complexities of musculoskeletal geometry and the EMG-to-muscle force conversion process.

The highest R² values occurred when both contact force peaks were analyzed together, indicating that the external measures were reasonable indicators of the large differences between the two peaks. However, the same external measures were much weaker indicators of the smaller changes in the individual peaks, the one exception being the first peak in lateral contact force. Nonetheless, RMS errors were of comparable magnitude between the two peak and single peak analyses. Thus, despite widely differing R² values, the regression equations had similar accuracy for predicting both contact force peaks together or each peak separately.

Our data suggest that the external knee adduction moment may be a better indicator of medial-lateral load distribution than of medial contact force.³ To explore this possibility further, we performed post-hoc regression analyses for both peaks together to correlate the external knee adduction moment with various contact force ratios as well as with absolute contact forces. The analyses yielded R² values of 0.54, 0.55, 0.59, 0.46 for medial-to-total, lateral-to-total, medialto-lateral, and lateral-to-medial contact force ratios, respectively, but only 0.25, 0.26, and 0.02 for medial, lateral, and total contact force, respectively. Though considerably larger R² values were observed for contact force ratios than for absolute contact forces, the knee adduction moment accounted for at most about 60% of the variability, indicating that other quantities (e.g., muscle forces) must account for the remaining variability. Consistent with the contact force ratio regression results, medial thrust gait had the lowest external knee adduction moment and lowest medial-to-total contact force ratio. Paradoxically, however, medial contact force still increased relative to normal gait, likely because total contact force increased even more. This paradox is resolved if the external knee adduction moment is viewed as an indicator of medial-to-total contact force ratio rather than of actual medial contact force.

A similar paradox between longitudinal gait studies and gait retraining studies is resolved if the external knee adduction moment is viewed as an indicator of medial-to-total contact force ratio.³ Longitudinal gait studies have found that knee osteoarthritis progression is strongly correlated with the peak external knee adduction moment at baseline.⁴ If

$$M_{add}\propto \frac{F_{med}}{F_{tot}}\text{which implies}{F}_{med}\propto M_{add}{F}_{tot}$$ (3)

then an increased M_add with an unchanged total contact force F_tot would suggest an increased medial contact force F_med, making the knee adduction moment a good indicator of medial contact force as well. In contrast, gait modification studies (where total contact force may change significantly within a single gait session) have reported that changes in medial contact force are not necessarily strongly correlated with changes in the peak knee adduction moment.⁴² Based on Eq. (3), if M_add decreased but F_tot increased more, the net effect could be an increase in F_med, making the external knee adduction moment a poor indicator of medial contact force. In contrast, if F_tot changes little, then changes in M_add may be a strong indicator of changes in medial contact force, as reported in a recent study by Erhart et al. (2010).²⁵

For this subject, external knee superior force was a surprisingly weak indicator of total contact force peaks, especially when each peak was analyzed separately. Nonetheless, this quantity (or its related internal force from inverse dynamics) is frequently used in experimental studies as a surrogate for total contact force.^{6,17,18,32–36} In our study, no differences in F_sup were found between the four gait patterns even though significant differences in F_tot were observed. Thus, use of F_sup as an indicator of F_tot would have led to erroneous conclusions about how the different gait patterns affected total knee contact force. Furthermore, when we performed post-hoc regression analyses of total contact force with F_sup removed but |M_flex| retained, R² values dropped by 0.11 for the first peak and remained largely unchanged for the second peak and both peaks together. Thus, F_sup did not have a large impact on predicting total contact force peaks accurately.

The most obvious explanation for the weak performance of F_sup is lack of accurate predictions for how muscle forces contributed to total contact force, as suggested by Eq. (2). For analysis of the first peak and both peaks together, R² values for total contact force increased by 0.17 and 0.11, respectively, when |M_flex| or EMG values were added to the regression. Since |M_flex| peaks were highly correlated with EMG peaks (R² = 0.88 based on a post-hoc regression analysis of both peaks together), these R² increases suggest that knowledge of muscle forces (which cannot be measured experimentally) may be essential for predicting total contact force accurately.⁴⁸ While ligament forces may contribute to total contact force as well, ligaments function primarily to prevent lift-off. Thus, it would be surprising if ligaments had significant total contact force contributions during stance phase when the knee is highly loaded.

The primary limitations of this study are that all data were collected from a single subject with an implanted knee and valgus alignment during a single test session. While the study demonstrated that much of the variation in the subject's knee contact forces during different gait motions was not accounted for by variations in the subject's external knee loads and muscle EMG signals, the extent to which similar findings apply to other subjects (e.g., subjects with natural knees, with or without knee OA) or motions (e.g., squatting or stair climbing) is unknown. Furthermore, our subject performed unfamiliar gait patterns, likely without sufficient time and training to learn them well. Due to the awkwardness, discomfort, and/or instability associated with performing an unfamiliar gait pattern, there may have been a significant increase in muscle co-contraction and thus total contact force, which would reduce the strength of any correlations found. For studies of subjects performing their normal walking motions or a comfortable gait pattern, it is possible that external measures will be better predictors of internal knee contact forces. Finally, since our subject was implanted with a force-measuring knee replacement and had a residual valgus alignment, the extent to which our findings apply to individuals with healthy or diseased natural knees with a neutral or varus alignment is also unknown. The changes in knee contact forces observed in this unique subject performing modified gait motions are likely to be different from those that would occur in healthy subjects or subjects with knee OA and a varus alignment. Our subject would not be a candidate for gait modification as a clinical intervention, and conclusions about the effectiveness of different gait modifications for patients with medial knee OA should not be inferred from the results of our study.

In conclusion, this study demonstrated that external knee load and EMG measures were not strong indicators of medial, lateral, and total contact force in the knee for this particular subject. Erroneous conclusions about how different gait patterns affected total contact force would have been reached if inferior knee force from inverse dynamics was used as an indicator of total contact force. Furthermore, the external knee adduction moment was more strongly correlated with medial-to-total contact force ratio than with medial contact force, a result that explains the paradoxical observation of a decrease in peak knee adduction moment with a corresponding increase in peak medial contact force. Our findings suggest that it may be unwise to focus on only the knee adduction moment as an indicator of medial contact force in situations where total contact force may be changing (e.g., due to changes in muscle forces). Advances in musculoskeletal modeling methods may eventually make it possible to estimate internal contact forces in the knee accurately for individual patients during gait.²⁶