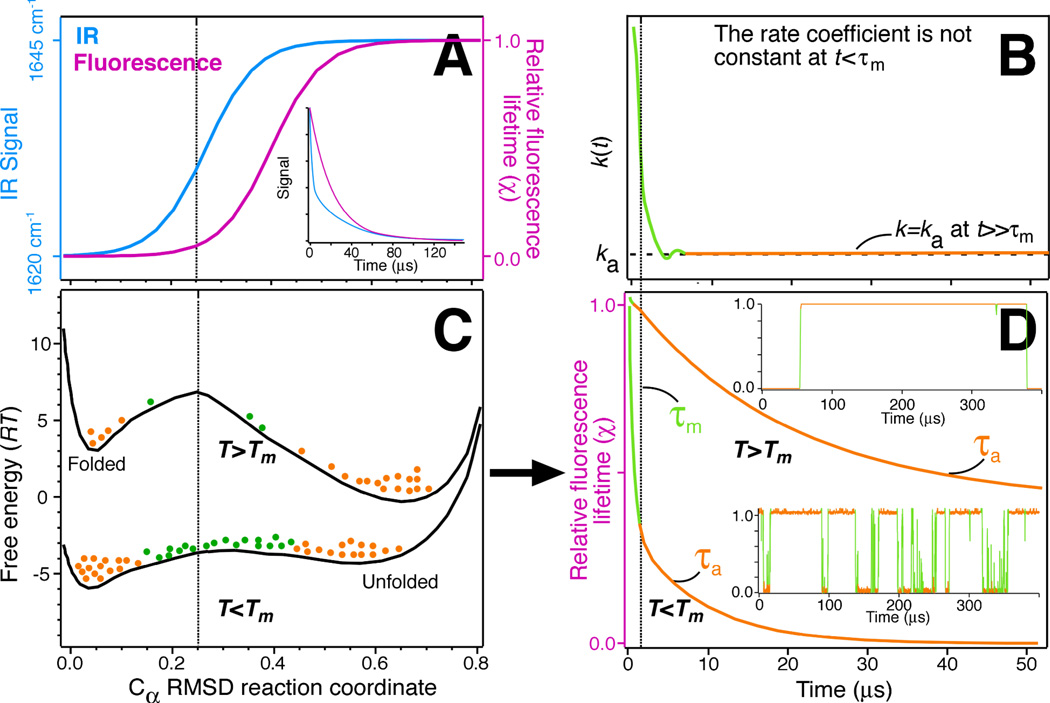
Figure 2.

Folding from activated to downhill, using WW domain experiments and simulations as an example. (A and C) The bottom shows schematic free energy profiles⁷,⁴⁹ along the C_α-RMSD reaction coordinate at temperatures below and above the melting temperature T_m. Protein populations in folded/unfolded minima (orange) and near the barrier (green) are shown as dots. The top (A) shows two probe signal profiles. They increase monotonically with reaction coordinate, and can therefore serve as experimental reaction coordinates. Different probes progress differently as the protein folds,⁴⁹ yielding probe-dependent kinetics particularly when the barrier is low and the protein population samples the transition region (vertical dashed line). (B). On the molecular time scale τ_m (vertical dashed line) the rate coefficient is not a ‘rate constant,’ but depends on time:⁵¹ pre-activated population (green in C) reacts promptly, much faster than population that needs to be activated (orange in C). Only later does the rate coefficient ‘settle down.’ (D) In the example, at T>T_m the pre-activated population is negligible, and exponential-decay kinetics with a ‘slow’ time constant τ_a is observed. At T<T_m, the pre-activated population is large, and a prompt phase precedes the ‘slow’ exponential-decay kinetics. The insets in (D) show corresponding single molecule traces: For a high barrier (T>T_m in the example), the activated protein (green), is sampled rarely. For a low barrier (T<T_m), the activated protein is sampled frequently. In essence, there are always pre-activated proteins that fold promptly downhill at the ‘speed limit.’ If the barrier is large, this population is unobservably small due to the Boltzmann factor. If the barrier is small, the population becomes easy to observe.⁸⁵ The terms ‘molecular time,’ ‘speed limit’, ‘transition state transit time,’ ‘downhill folding time’ refer to the same time scale, but are not identical. Also, the decay in (D) at t<τ_m is not necessarily an exponential with time constant τ_m,⁸⁷ although frequently fitted as such.^{49, 85, 100}