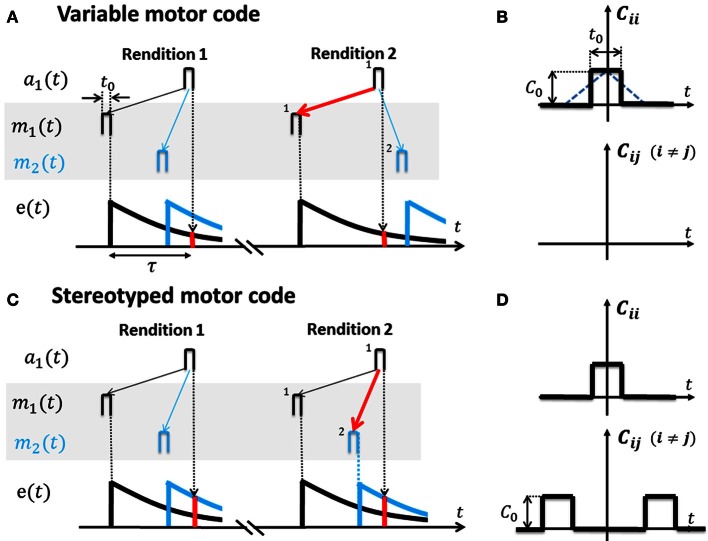
Figure 3.

Cross-correlation functions for variable and stereotyped motor codes. (A) In a variable motor code m(t) (shaded area). Activity bursts m₁ (black) and m₂ (blue) of width t₀ in two example motor neurons occur at diverse time lags relative to each other across renditions of the song motif. Auditory tuning in the shown sensory neuron is such that it responds a₁ to bursts m₁ after a time lag τ. Repeated co-activation m₁ → a₁ and non-zero eligibility e(τ) (red bar) at time lag τ leads to increased synaptic weight V₁₁ (red arrow) and to a causal inverse. Lack of correlation between m₂ and a₁, as well as heterosynaptic competition, prevents V₂₁ from similarly increasing (blue thin arrow). (B) The cross-correlation function C_ij(t) for variable codes is flat except the auto-correlation peak at zero time lag (motor activity is uncorrelated among neuron pairs). Note: based on square activity pulses in motor neurons in (A) the true cross-correlation shape is triangular (blue dotted line) which we approximate by a square pulse of width t₀ ≃ 10 ms. The auto-correlation peak height is C₀. (C) In a stereotyped motor code m(t) (shaded area), bursts m₁ (black) and m₂ (blue) occur at a fixed time lag relative to each other across renditions of the song motif (traveling pulse of activity). Repeated co-activation m₂ → a₁ at higher eligibility (red bar) than the eligibility of m₁ → a₁ leads to strengthening of synapse V₂₁ (red arrow) and to a predictive inverse. (D) The cross-correlation function C_ij(t) for stereotyped codes peaks also at non-zero time lags.