Figure 1.

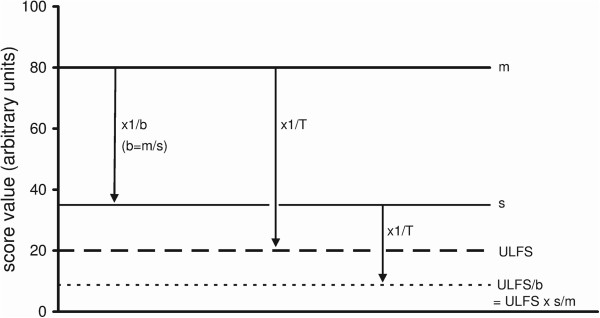
Score levels of two patients, with a maximal baseline ‘m’ and a smaller baseline ‘s’ respectively. ’ULFS’, upper limit of functional status. The zone of functional status with residual score levels is located between zero and ULFS. T, treatment factor by which the maximal baseline ‘m’ is just decreased to level ULFS (by a factor 4 in this example; m decreases from 80 to 20 units). When the same factor T is applied to the smaller baseline ‘s’of 35 units, this baseline is decreased below ULFS to ULFS/b, from 35 to 8.75 units. If factor T is tuned to the patient with baseline ‘m’, for reaching ULFS, the end level of any smaller baseline will enter the zone of functional status when the same factor T is applied to this smaller baseline. Mathematical proof: For the patient with level m, treatment must be so effective that m decreases at least by a treatment factor T to reach ULFS, thus: m/T = ULFS (T > 1) [equation (1)]. The ratio between the patients’ baselines equals m/s = b (b, baseline factor, b > 1 ). Thus m = s.b and substituting s.b for m in equation (1) yields: (s.b)/T = ULFS, thus the score level reached by factor T for the patient with baseline s is given by: s/T = ULFS/b. Level ULFS/b (b > 1) is lower than level ULFS. Therefore, the value of factor T derived from a patient with the highest score level is applicable to any patient with a lower level for attaining a final level that falls within the zone of functional status. The end level (ULFS/b) for the patient with initially level ‘s’ equals (ULFS.s/m). Thus an end score will be located between zero and ULFS, proportionally with the baseline level ‘s’.