Figure 2.

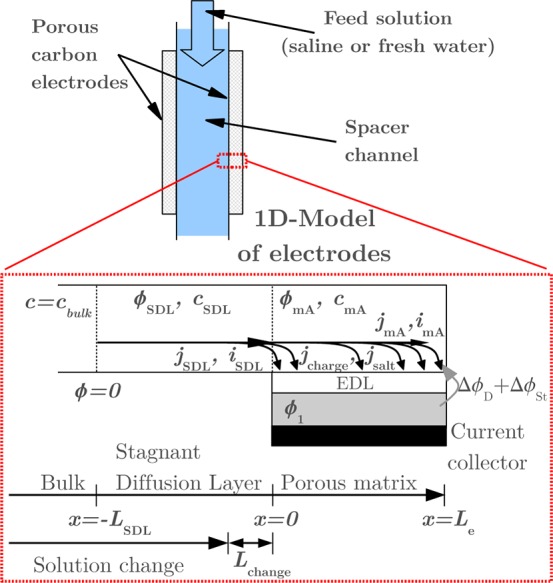
One-dimensional model of electro-diffusion of ions in porous electrodes applied to CAPMIX. The uniform rates of charge (i_SDL(t)) and neutral salt (j_SDL(t)) transport in the stagnant diffusion layer (SDL) due to gradients of salt concentration and electric potential are modified inside the porous matrix due to adsorption of both charge (j_charge(x,t)) and salt (j_salt(x,t)) into the EDLs that form at the solid–liquid interface, leading to position- (and time-) dependent quantities i_mA(x,t) and j_mA(x,t). The local values of electric potential (ϕ(x,t)) and salt concentration (c(x,t)) determine, together with the adsorbed charge in the EDLs, the (unique) electrode potential through appropriate EDL models. At the beginning of the open circuit steps, the solution in the bulk and in part of the SDL is substituted. The part of the SDL that is not changed instantaneously is determined by the length L_change. C_bulk is either C_saline or C_fresh.