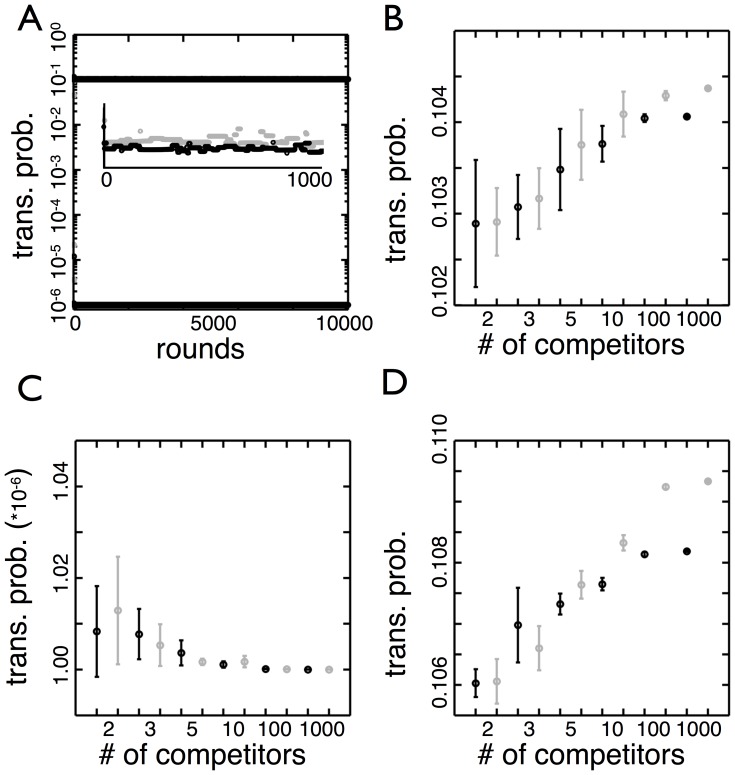
Figure 6. Competition between more than two genotypes.
(A) The transition probabilities of the winning genotype are shown over 10,000 environmental cycles (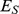 to
to 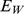 to
to 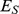 ). Each cycle begins with 1,000 genotypes in equal number and at the end of a full cycle the most abundant genotype (the winner) advances to the next round to compete against new, randomly generated genotypes. The transition probabilities of the winner are grouped by environmental state: black is
). Each cycle begins with 1,000 genotypes in equal number and at the end of a full cycle the most abundant genotype (the winner) advances to the next round to compete against new, randomly generated genotypes. The transition probabilities of the winner are grouped by environmental state: black is 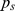 and
and 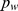 in
in 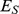 and gray is
and gray is 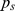 and
and 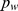 in
in 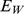 . The inset is a magnification that shows the winner changes frequently but in the long run the winner appears as unbroken lines. (B) The transition probabilities (
. The inset is a magnification that shows the winner changes frequently but in the long run the winner appears as unbroken lines. (B) The transition probabilities (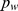 in
in 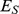 is black and
is black and 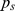 in
in 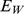 is gray) of the winning genotypes are shown as a function of the numbers of competitors. Each point is the average of 10 runs of tournaments (as shown in A) sampled every 100 rounds after 2500 rounds (“burn-in phase”). The error bars are the standard deviation of the 10 runs. The values remain close to
is gray) of the winning genotypes are shown as a function of the numbers of competitors. Each point is the average of 10 runs of tournaments (as shown in A) sampled every 100 rounds after 2500 rounds (“burn-in phase”). The error bars are the standard deviation of the 10 runs. The values remain close to 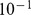 for different numbers of competitors. (C) The same as B but this shows the other two transition probabilities (
for different numbers of competitors. (C) The same as B but this shows the other two transition probabilities (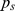 in
in 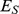 is black and
is black and 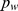 in
in 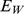 is gray) for winning genotypes. The values are all close to the lower bound of transition probabilities considered (
is gray) for winning genotypes. The values are all close to the lower bound of transition probabilities considered (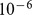 ), so the number of competitors has no effect. (D) The transition probabilities of the winning genotype are shown when the probabilities do not depend on the environmental state. Due to the symmetry of the system the optimal
), so the number of competitors has no effect. (D) The transition probabilities of the winning genotype are shown when the probabilities do not depend on the environmental state. Due to the symmetry of the system the optimal 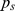 (gray) and
(gray) and 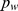 (black) are identical. There is a statistically significant increase in the transition probabilities for the competition of 1000 competitors versus 2, but the magnitude of difference is small.
(black) are identical. There is a statistically significant increase in the transition probabilities for the competition of 1000 competitors versus 2, but the magnitude of difference is small.