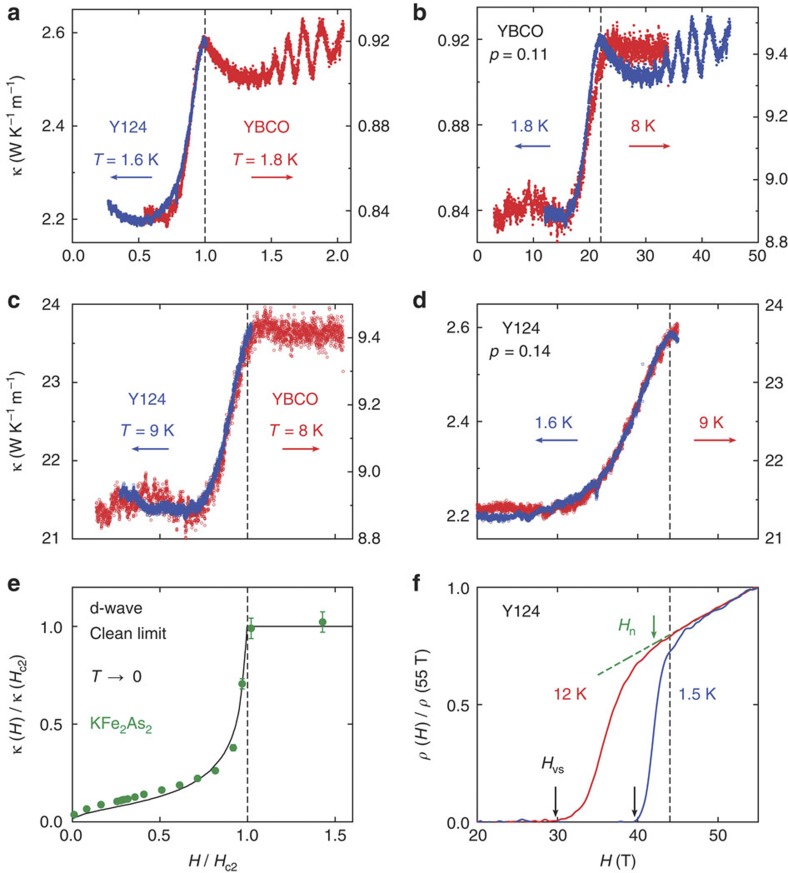
Figure 1. Thermal conductivity of YBCO and Y124.

(a–d) Magnetic field dependence of the thermal conductivity κ in YBCO (p=0.11) and Y124 (p=0.14), for temperatures as indicated. The end of the rapid rise marks the end of the vortex state, defining the upper critical field H_c2 (vertical dashed line). In Fig. 1a,c, the data are plotted as κ vs H/H_c2, with H_c2=22 T for YBCO and H_c2=44 T for Y124. The remarkable similarity of the normalized curves demonstrates the good reproducibility across dopings. The large quantum oscillations seen in the YBCO data above H_c2 confirm the long electronic mean path in this sample. In Fig. 1b,d, the overlap of the two isotherms plotted as κ vs H shows that H_c2(T) is independent of temperature in both YBCO and Y124, up to at least 8 K. (e) Thermal conductivity of the type-II superconductor KFe₂As₂ in the T=0 limit, for a sample in the clean limit (green circles). Error bars represent the uncertainty in extrapolating κ/T to T=0. The data9 are compared with a theoretical calculation for a d-wave superconductor in the clean limit8. (f) Electrical resistivity of Y124 at T=1.5 K (blue) and T=12 K (red) (ref. 11). The green arrow defines the field H_n below which the resistivity deviates from its normal-state behaviour (green dashed line). While H_c2(T) is essentially constant up to 10 K (Fig. 1d), H_vs(T)—the onset of the vortex-solid phase of zero resistance (black arrows)—moves down rapidly with temperature (see also Fig. 3b).