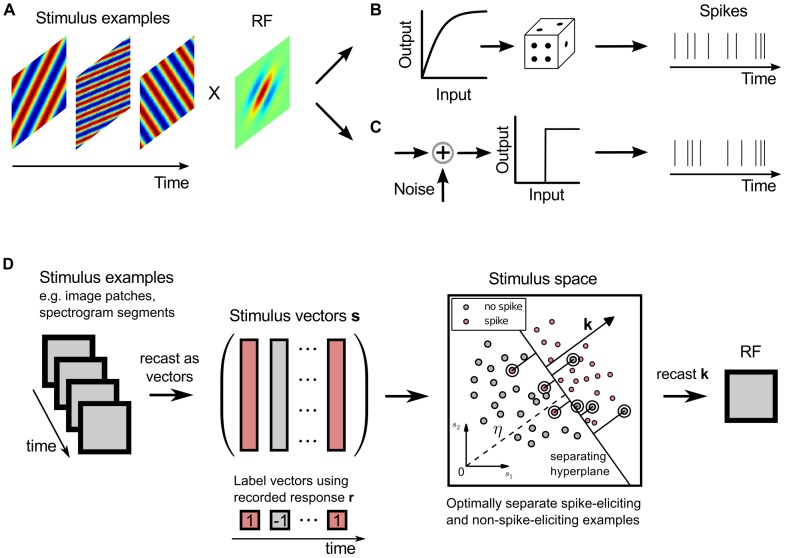
Figure 1. Classification-based receptive field estimation.

(A) Linear models of neural response generation perform integration of stimulus features using a linear filter corresponding to the neuron's receptive field (RF). (B) Standard linear-nonlinear Poisson (LNP) model: a static memoryless nonlinearity is applied to the linear stimulus projection with subsequent Poisson spike generation. (C) The binary model assumed here generates spikes from the (noisy) linear projection through an explicit threshold operation. (D) Stimulus examples, e.g., image or spectrogram patches, are recast as vectors in order to estimate the linear filter in the binary model. The binary responses recorded in experiments are used to label the resulting vectors as spike-eliciting (red) or non-spike-eliciting (gray). The proposed classification-based receptive field (CbRF) estimation method aims to identify the hyperplane that optimally separates the spike and non-spike classes in a high-dimensional space, whose dimensionality corresponds to the stimulus vectors' dimensionality. The box illustrates the principle in two dimensions: a large-margin classifier adjusts the separating hyperplane with normal vector k such that the risk of misclassifying stimulus examples is minimized. Misclassified stimulus examples are marked by white circles and their distance to the hyperplane by black lines. The estimate of the linear RF filter corresponds to the recast normal vector of the hyperplane.