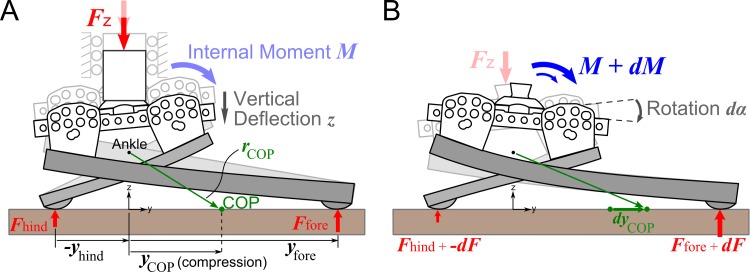
Fig. 1.

Two-phase loading demonstrates superposition of linear and angular terms in determining the force and deflection of the hindfoot and forefoot. (a) Pure vertical compression results in equal deflections of the forefoot and hindfoot, each with a force proportional to its linear component stiffness. The resultant equals the applied force (F _z), acting at a COP that remains constant as load increases (Eq. (2)). The moment supported by the ankle constraint is $M=y_{\mathrm{COP}-\mathrm{compression}}{F}_{\mathrm{z}}$. (b) The angle constraint can be replaced by its equivalent moment. Adding a differential moment (dM) shifts the load, moving the COP and changing the forefoot and hindfoot forces by ±dF. The accompanying deflection changes in each component result in an angular rotation dα. The overall angular stiffness relates this angle to the moment: $\mathit{dM}=K_{\mathrm{angular}}d\alpha$. The final state is equivalent to rotating first and then compressing, as in the proposed test method. This equivalence is used to derive an expression for angular stiffness based on measured changes in F _z and y _COP (Eq. (6)).