Figure 6.

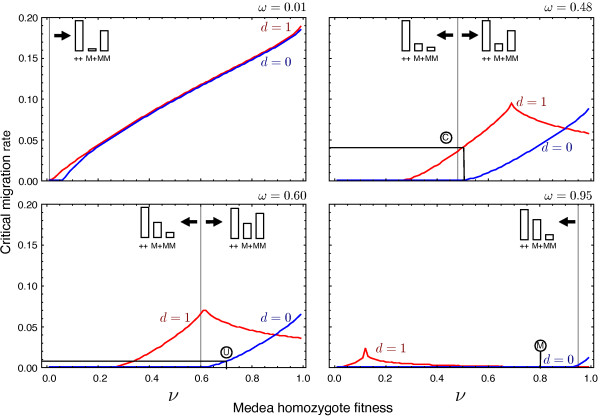
Critical migration rates allowing stable local transformations over a range of genotype and Medea parameter configurations. Using the recursion equations Eq. (1) with modifications as described in the “Population structure dynamics” section we explore the pattern when there is no Medea effect (d=0) and complete Medea lethality (d=1). For different values of the heterozygote fitness (ω) we explore the genotype configurations going from directional selection to underdominance. The transition in the fitness structure between these two states is indicated within the plots using token bar charts (illustrated graphically within the plots). Over a wide range of parameter space the combined construct exhibits substantially higher critical migration thresholds than ‘underdominance only’. Interestingly the d=1 dynamics are not monotonic. The figure illustrate how migrational stability can be enhanced, even with a reduction in fitness of the genetically modified homozygote. Disks indicate the positions of results plotted in Figure 2 for the ‘underdominance only’ system (U), the combined system (C) and ‘Medea only’ (M). Comparing the combined system with with ‘Medea only’ system we see that not only Medea but underdominance also is necessary to get the desired migrational stability in experimental systems.