Skip to main content
. Author manuscript; available in PMC: 2014 Aug 12.
Published in final edited form as: Psychometrika. 2012 Dec 8;78(3):441–463. doi: 10.1007/s11336-012-9304-2

Figure 1.

Figure 1

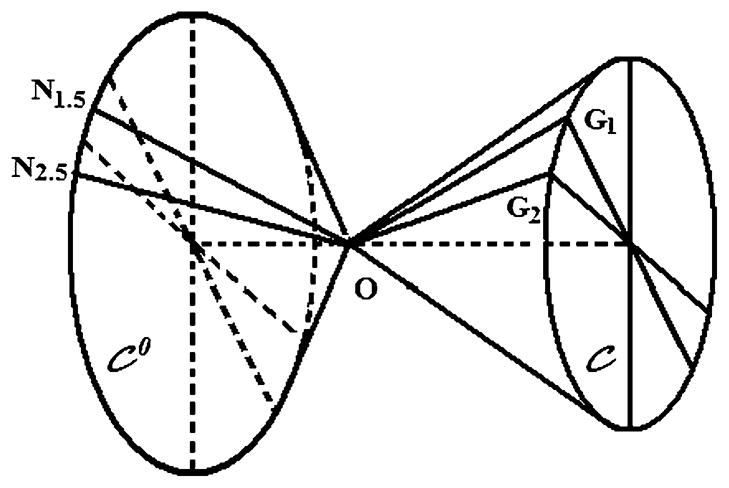
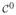An elliptic cone Inline graphic and its polar cone Inline graphic. Suppose the cone Inline graphic is approximated by a polyhedral cone with edges g1 =OG1, g2 =OG2, etc. If the discretization is fine, the normal vector of the face OG1G2 can be approximated by ON1.5, which is a generatrix on the surface of Inline graphic with longitude between those of g1 and g2. The projections of points inside the pyramid O – N1.5G1G2 to the polyhedral cone lie on the face OG1G2; the projections of points inside the pyramid O – G2N1.5N2.5 to the polyhedral cone lie on the edge OG2.