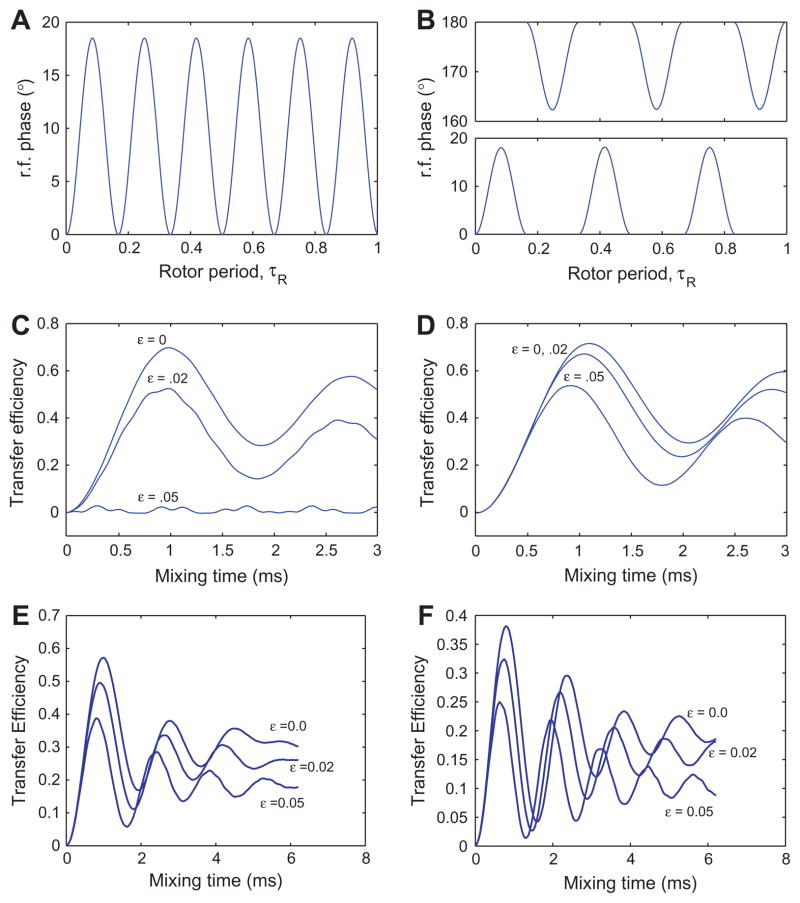
Fig. 1.

The top two panels show the phase of the rf irradiation as a function of time (in the units of τ_R) for the CMRR (A) and PAMORE (B) pulse sequences when C = 6ω_r. The bottom two panels show numerical simulations of the transfer efficiency for the ¹³C–¹³C spin-pair, 1.52 Å apart in a powder sample subject to 8 kHz MAS, an external magnetic field corresponding to a 360 MHz (Larmor frequency for ¹H) spectrometer and nominal rf-field strength on the ¹³C channel of 48 kHz, for the CMRR (C) and PAMORE (D) pulse sequences, for three different values of rf-inhomogeneity parameter, ε(0, .02, .05). Simulations use C = 6ω_r, giving τ_c = 125/6 μs and rf-power of ${\omega }_{rf}^C/2\pi =48\text{kHz}$. The efficiency is reduced with increasing ε. In panel C at ε = .05, there is almost no transfer. Simulations were done with SPINEVOLUTION software [14]. In Fig. C and D, we assume spins on resonance with no CSA. In Fig. E and F, we simulate the performance of the PAMORE pulse sequence with phase modulation implemented as constant phases over interval of .2 (as in C and D) and 1.3 μs respectively. The later correspond to experimental realization shown in Figs. 3 and 5. The simulated transfer is for I_y → S_y, where I and S spins are C_α and CO, respectively. Carrier is placed on CO resonance with C_α and CO resonances at 35 and 170 ppm respectively. Chemical shift ansiotropy of 19.3 and 70.2 ppm and asymmetry parameter η = 1.225 and η = 1.05 are used for C_α and CO spins respectively. The Euler angles (α, β, γ) relating the chemical shielding tensors to the principle axis system of the dipolar tensor are ${\mathrm{\Omega }}_{PC}^{{\mathrm{C}}_{\alpha }}=(-33.7,-95.3,48.92)$ and ${\mathrm{\Omega }}_{PC}^{\text{CO}}=(154.32,-2.9250,-106.8120)$ [12].