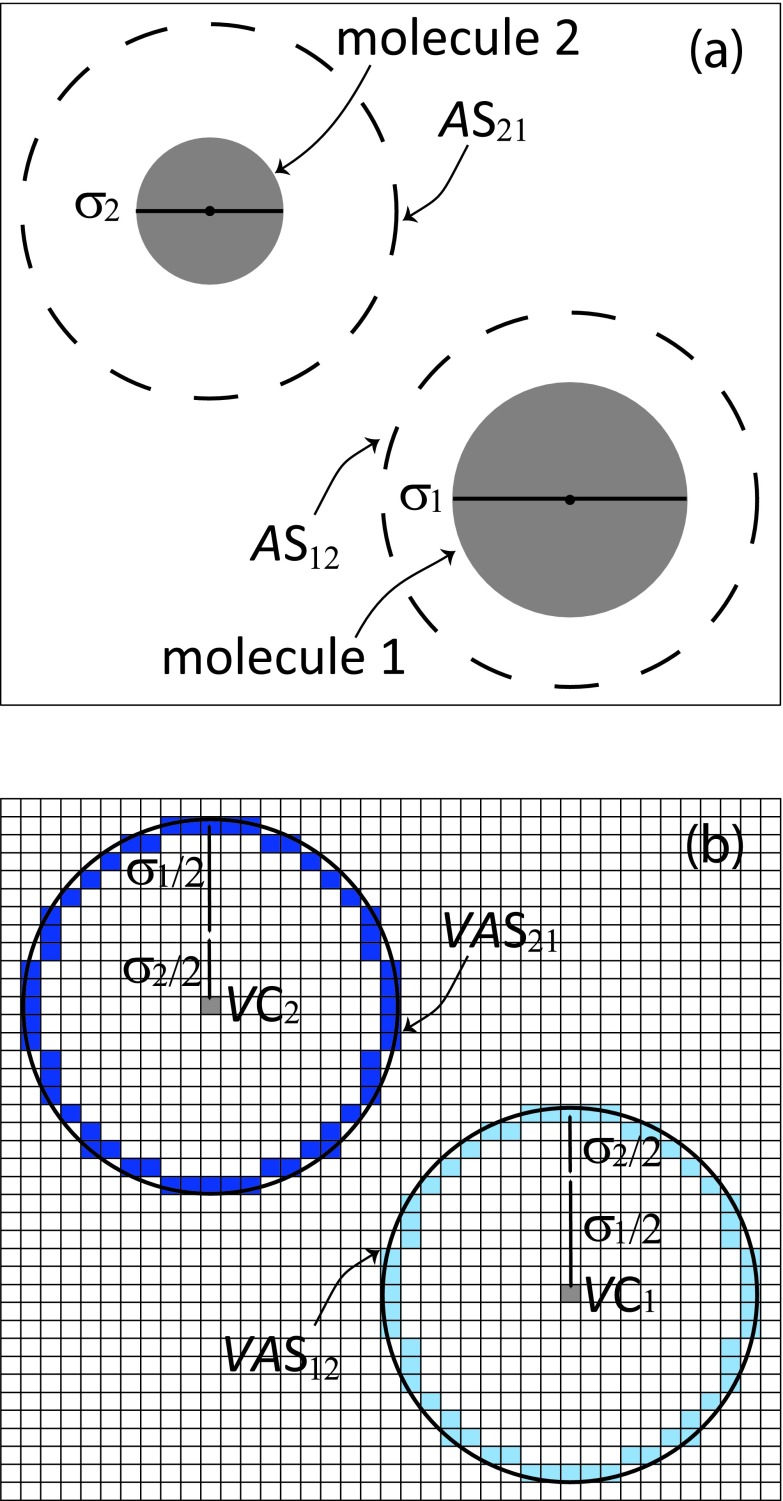
FIG. 5.

(a) Molecules 1 and 2 with their respective diameters σ₁ and σ₂. We imagine each molecule to be concentrically surrounded by an “action sphere” of radius $\frac{1}{2}({\sigma }_1+{\sigma }_2)\equiv {\sigma }_{12}$. When the two molecules collide, the center of each molecule will be in contact with the action sphere about the other molecule. (b) In the SVTA, the centers of molecules 1 and 2 are assumed to be distributed randomly and uniformly inside voxels VC₁ and VC₂, respectively. The action sphere about molecule j is now represented by the set of all voxels whose centers are ⩽σ₁₂ from the center of voxel VC_j and which have at least one adjacent voxel that does not satisfy that condition. Here the voxelated action sphere VAS₂₁ about molecule 2 relative to molecule 1 is the set of dark blue voxels, and the voxelated action sphere VAS₁₂ about molecule 1 relative to molecule 2 is the set of light blue voxels. In the SVTA, the two molecules collide with each other whenever the center of either molecule comes into contact with the boundary of the voxelated action sphere about the other molecule. That will be possible only if either VC₁ has hopped to a voxel that is adjacent to VAS₂₁, or VC₂ has hopped to a voxel that is adjacent to VAS₁₂. VAS₁₂ hops rigidly with VC₁, and VAS₂₁ hops rigidly with VC₂; however, in an actual simulation we do not need to explicitly execute the hops of either voxelated action sphere.