Table 1. Systematic errors.
| Error source | Normalized variation s Category A (10−9/mm) | ‹z›Δ Category B (mm) | Time invariant ‹z› Category D (mm) | Q error net (10−8) |
|---|---|---|---|---|
| 10 s Hold time. | − | − | ±0.04* | − |
| Improved B(r,t), dB/dt terms, adaptive Runge-Kutta propagator. | ±0.01 [±0.01]* | ±0.09 [±0.03]† | ±0.20† | ±0.03† |
| Degraded E(r,t). | ±0.01 [±0.01]* | ±0.10 [±0.03]† | ±0.24† | ±0.03† |
|c> Space 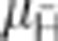 included. included. |
±0.01 [±0.01]* | − | ±0.07* | ±0.01* |
| ±1% Differential mirror drift. | − | ±0.13 [±0.04]‡ | ±0.29‡ | ±0.05‡ |
| ±1% Common mode mirror drift. | ±0.01 [±0.01]‡ | ±0.02 [±0.00]‡ | ±0.03‡ | ±0.02‡ |
| 2 mrad Solenoid tilt. | ±0.01 [±0.02]* | ±0.07 [±0.02]* | ±0.15* | ±0.03* |
| ±1% Octupole drift. | − | ±0.01 [±0.00]* | ±0.03* | ±0.01* |
| External magnet. | − | ±0.06 [±0.02]‡ | ±0.11‡ | ±0.02‡ |
| 3 × Radius, 2 × length. | ±0.01 [±0.02]* | ±0.07 [±0.02]* | ±0.15* | ±0.03* |
| Initial energy distribution. | ±0.22 [±0.29]‡ | ±0.04 [±0.01]‡ | ±0.05‡ | ±0.31‡ |
| Anisotropic initial distribution. | ±0.08 [±0.11]† | ±0.17 [±0.06]† | ±0.23† | ±0.12† |
| Detector z-centre relative to electrode z-centre. | − | ±0.17 [±0.06]‡ | ±0.2‡ | ±0.06‡ |
| Mirror z-centre relative to electrode z-centre. | − | − | ±0.5‡ | − |
| Detector efficiency. | ±0.03 [±0.04]‡ | ±0.02 [±0.01]‡ | ±3.6‡ | ±0.04‡ |
| Long-term detector drift. | − | ±0.04 [±0.01]‡ | ±0.09‡ | ±0.01‡ |
| Cosmic background. | − | ±0.25 [±0.08]§ | − | ±0.08§ |
| Antiproton background. | − | ±0.13 [±0.04]‡ | − | ±0.04‡ |
Entries in [] are the induced Q error associated with the adjacent entries, scaled by 10−8. (These entries do not always sum to the corresponding entries in the Q Error Net column because of rounding and because the entries in the sum are sometimes known to have opposite sign.) The normalized variation in the sensitivity s=dQ/d‹z›Δ is defined to be s/sNom−1, where sNom is the sensitivity evaluated with nominal parameters. Entries that are zero to two digits are designated with a ‘−’.
*Effects for which the 1σ sampling errors in the simulations used to study the effects are larger than the size of the effect predicted by the simulation; thus, we cannot determine whether these effects would cause an actual change in the experimental observations. More precisely, the simulations generally predict an effect of size a±b. For these entries, |a|<b; to give an estimate of the worst case errors possible for these effects, we report the value of b, not that of a. For all other entries, we report a.
†Comparisons between simulations where |a|>b.
‡The potential effect caused by some independently measurable parameter c±d is real, that is, the simulations or calculations predict |a(c)|>b(c), but the 1σ errors in the measurement of the relevant parameter are compatible with zero, that is, |c|<d. Consequently, we do not know whether these error sources cause an actual change in our data.
§An effect based on an independently measured parameter that is not compatible with zero; this effect is likely to have caused a change to our data.
