Abstract
The sequence of redox reactions in the natural environment generally follows the electron affinity of the electron acceptors present and can be rationalized by the redox potentials of the appropriate half-reactions. Answering the question how halogenated aromatics fit into this sequence requires information on their Gibbs free energy of formation values. In 1992 Gibbs free energy data for various classes of halogenated aromatic compounds were systematically explored for the first time based on Benson’s group contribution method. Since then more accurate quantum chemical calculation methods have become available. Here we use these methods to estimate enthalpy and Gibbs free energy of formation values of all chlorinated and brominated phenols. These data and similar state-of-the-art datasets for halogenated benzenes and benzoates were then used to calculate two-electron redox potentials of halogenated aromatics for standard conditions and for pH 7. The results underline the need to take speciation into consideration when evaluating redox potentials at pH 7 and highlight the fact that halogenated aromatics are excellent electron acceptors in aqueous environments.
Electronic supplementary material
The online version of this article (doi:10.1007/s10532-014-9710-5) contains supplementary material, which is available to authorized users.
Keywords: Chlorophenol, Bromophenol, Dehalogenation, Redox potential, Chlorobenzoate, Chlorobenzene
Introduction
The seminal observations by Tiedje and co-workers on microbial dehalogenation have profoundly altered our perception of biodegradability of halogenated compounds (Suflita et al. 1982). We now know that anaerobic bacteria reductively dehalogenate a wide variety of organohalogens in a process called organohalide respiration, where organohalogens are used as electron acceptor by bacteria harnessing part of the energy released in the form of ATP (McCarty 1997; Farai et al. 2010; Leys et al. 2013). Based on this information treatment processes have been developed for the biodegradation of various classes of organohalogens including halophenols (Field and Sierra-Alvarez 2008). Insight in the microbial and thermodynamic logic behind the sequence of dehalogenation steps observed in these degradation processes (Dolfing 2003) requires an internally consistent set of data on the standard aqueous phase Gibbs free energy of formation (ΔG of) and enthalpy of formation (ΔH of) values for all congeners. For chlorophenols such a data set is currently not available. A previous set of data for chlorinated phenols (Dolfing and Harrison 1992) lacked values for ΔH of, thus precluding its use at temperatures other than 298.15 K. Also, with the advent of accurate quantum chemical calculation methods and readily available computer power it is now possible to generate data sets that are more accurate than those generated 20 years ago. The objectives of the present paper are therefore (i) to present a state-of-the-art data set of ΔG of and ΔH of values for chlorinated phenols, and (ii) to do the same for brominated phenols. Speciation of halogenated phenols is pH dependent, and potentially affects the energetics of the dehalogenation reactions (Dolfing et al. 2010). Thus, our third objective is to outline the effect of pH on the change in Gibbs free energy for the reductive dehalogenation of halogenated phenols and on the redox potentials for the corresponding redox couples. In recent years Tang et al. (2010) and Sadowsky et al. (2013) have updated the existing database of thermochemical properties for halogenated aromatics with state-of-the-art quantum chemical information on chlorobenzoates and halobenzenes. The fourth objective of the present paper is to integrate these data into a consistent set of redox potentials for various classes of halogenated aromatics.
There is currently considerable interest in the use of microbial fuel cells for waste treatment, including waste containing halogenated phenols (Strycharz et al. 2010; Huang et al. 2012, 2014). Rational design and implementation of such systems hinges on precise knowledge of the amount of energy present in the waste (Heidrich et al. 2011). In addition to providing data for the prediction and rationalization of degradation pathways of various classes of halogenated aromatics in anaerobic environments (Dolfing 2003) the data presented here will allow a thermodynamic evaluation of the fraction of energy that is stored in the carbon-halogen bond of halogenated benzenes, benzoates and phenols.
Materials and methods
Computational methods
Ab initio quantum chemical calculations to estimate ΔH of and So values of phenol and all chlorinated and brominated phenol congeners were performed with the Gaussian 03 software, Revision E1 (Frisch et al. 2003). The use of this software for thermochemical calculations is well established (Novak 2004). The composite G3(MP2)/B3LYP method was used for calculation of total energies; the method (Baboul et al. 1999) has typical precision of 4 kJ/mol. The G3(MP2)/B3LYP method yields ΔG of and ΔH of values for the gas phase. For environmental applications data for the aqueous phase are generally more relevant. We therefore used the universal solvation model (Marenich et al. 2009) and G3(MP2)/B3LYP method to simulate water solvent as implemented in the Gaussian software to calculate ΔG of and ΔH of values for the aqueous solution.
Gibbs free energy values
The standard molar Gibbs free energy of formation was calculated from the equation where ΔH of is the standard enthalpy of formation at 1 bar (100 kPa), T is the temperature of interest (298.15 K), S o is the absolute standard entropy, νi is the stoichiometric coefficient of element I, and S οi is the absolute entropy of element I in its standard reference state. S οi values used for carbon, hydrogen, oxygen, bromine and chlorine were 5.74, 65.34, 102.58, 76.11 and 111.54 J.K−1 mol−1 respectively (Cox et al. 1989). We have also calculated the appropriate total energies H mol, H at, G mol, G at in the water solvent which allowed us to deduce the solvation energy correction for gas phase data and thus convert ΔH of (g) and ΔG o of(g) values to ΔH of (l) and ΔG of (l). The exact expressions for H mol, H at, G mol, G at are given in Gaussian G03 manual (Frisch et al. 2003).
The amount of free energy available from a reaction is given by the relationship (Thauer et al. 1977). In aqueous solutions the standard state of all solutes is 1 mol/kg activity, that of water is the pure liquid. Under environmentally relevant conditions the concentrations of reactants and products are not 1 mol/kg. This is considered in ΔG′ values. For a hypothetical reaction aA + bB → cC + dD, ΔG′ values are calculated by using the mass equation
| 1 |
The ΔG o′ value is obtained from the ΔG o value by making the appropriate corrections for pH 7 (Thauer et al. 1977). ΔG of values for inorganics were taken from Stumm and Morgan (1996).
For example for the hydrogen driven reductive dehalogenation of chlorobenzene to benzene, that is for chlorobenzene + H2 → benzene + H+ + Cl−: and
Speciation and pH
Halophenols are weak acids, but stronger than phenol. In waste water weak acids are partially ionized and are in thermodynamic equilibrium with their conjugate bases. The notion that these species are in equilibrium implies that Gibbs free energy values for reactions where these compounds are reactants or products are calculated by using the ΔG f values of either the acid, with the formula
| 2 |
or the conjugated base with the formula
| 3 |
where (Dolfing et al. 2010). ΔG f values for halobenzoates were calculated after Dolfing and Harrison (1992) as
| 4 |
Gibbs free energies of chlorinated benzenes
Gibbs free energy of formation data for chlorinated benzenes in the aqueous phase (in kcal mol−1) were taken from Sadowsky et al. (2013) and converted to kJ mol−1 (1 kcal = 4.184 kJ). These values were used to calculate the change in Gibbs free energy for the reductive dehalogenation reactions as described previously (Dolfing and Harrison 1992).
Redox potentials
Two electron reduction potentials were calculated after Thauer et al. (1977). For example: based on G of values of −138.5 and −133.5 kJ mol−1 for C6Cl6 and C6Cl5H respectively and values of 0 and −39.95 for H+ at pH 0 and pH 7 respectively, and with ΔG f H2 (gas) = 0 kJ mol−1; ΔG f Cl− = −131.3 kJ mol−1 (Stumm and Morgan 1996) reductive dechlorination of hexachlorobenzene to pentachlorobenzene according to C6Cl6 + H2 (gas) → C6Cl5H + H+ + Cl− yields −126.3 kJ mol−1 under standard conditions (pH 0) and −166.2 kJ mol−1 at pH 7. To calculate the corresponding redox potentials these values are then divided by −nF/1,000,000 where n is the number of electrons transferred in the reaction and F is the Faraday constant (96485 J/V) (Stumm and Morgan 1996) and 1,000,000 is the multiplication factor to account for conversion of kJ to mV rather than J to V. This would yield reduction potentials of 654 and 861 mV for pH 0 and pH 7 respectively. For pH 7 the latter value still needs to be corrected for the redox potential of the H+/H2 redox couple, which is −414 mV at pH 7 (and indeed 0 at pH 0). Thus the redox potentials of the C6Cl6/C6Cl5H redox couple are 654 mV at pH 0 and 447 mV at pH 7 respectively.
Results and discussion
ΔGof and ΔHof values of chlorinated phenols
Quantum mechanical methods discriminate between conformers that are deemed to represent the same compound in the environment. For example, ΔG of for syn-2-chlorophenol (2-chlorophenol) differs from ΔG of for anti-2-chlorophenol (6-chlorophenol) (Supporting Information (SI) Table S1). This difference reflects the presence or absence of intramolecular hydrogen interaction (“bond”) between hydroxyl hydrogen and the halogen. In environmental chemistry this distinction between conformers is not made, because in the environment each congener is present in the conformation that has the lowest energy. In the supporting material ΔG of and ΔH of values for all 31 chlorophenol congeners are provided. In Table 1 we present ΔG of and ΔH of values for the environmentally relevant congeners.
Table 1.
Thermodynamic data for chlorinated phenols under standard conditions (in kJ mol−1 ) a
| ΔH of gas | ΔG of gas | ΔH of aq | ΔG of aq | ΔH of bgas(exp) | |
|---|---|---|---|---|---|
| Phenol | −96.4 | −51.2 | −118.0 | −72.8 | −96.4 |
| 2-chlorophenol | −132.8 | −83.7 | −143.9 | −95.2 | |
| 3-chlorophenol | −126.3 | −77.8 | −148.4 | −99.9 | |
| 4-chlorophenol | −124.2 | −75.7 | −147.0 | −98.6 | |
| 2,3-dichlorophenol | −154.7 | −101.7 | −165.1 | −112.4 | −151.6 |
| 2,4-dichlorophenol | −156.6 | −104.2 | −168.1 | −116.3 | −156.3 |
| 2,5-dichlorophenol | −162.2 | −109.7 | −173.1 | −120.9 | −158.4 |
| 2,6-dichlorophenol | −152.6 | −99.9 | −164.7 | −112.8 | −146.3 |
| 3,4-dichlorophenol | −145.9 | −93.7 | −168.3 | −116.3 | −150.3 |
| 3,5-dichlorophenol | −151.8 | −99.9 | −173.0 | −121.2 | −148.2 |
| 2,3,4-trichlorophenol | −174.8 | −118.1 | −184.3 | −128.0 | |
| 2,3,5-trichlorophenol | −179.6 | −123.3 | −188.5 | −132.3 | |
| 2,3,6-trichlorophenol | −173.9 | −117.4 | −184.4 | −131.0 | |
| 2,4,5-dichlorophenol | −177.3 | −121.1 | −187.6 | −131.8 | |
| 2,4,6-trichlorophenol | −180.5 | −124.5 | −191.3 | −136.5 | |
| 3,4,5-trichlorophenol | −167.2 | −111.2 | −188.1 | −132.0 | |
| 2,3,4,5-tetrachlorophenol | −191.7 | −131.4 | −199.6 | −139.4 | |
| 2,3,4,6-tetrachlorophenol | −192.4 | −132.2 | −200.5 | −140.0 | |
| 2,3,5,6-tetrachlorophenol | −193.8 | −133.5 | −201.4 | −142.2 | |
| Pentachlorophenol | −202.5 | −137.9 | −207.9 | −144.3 |
aStandard conditions are: 25 °C; 100 kPa (gas phase) or 1 M (aqueous solution)
bExperimental values are from Linstrom and Mallard (2012)
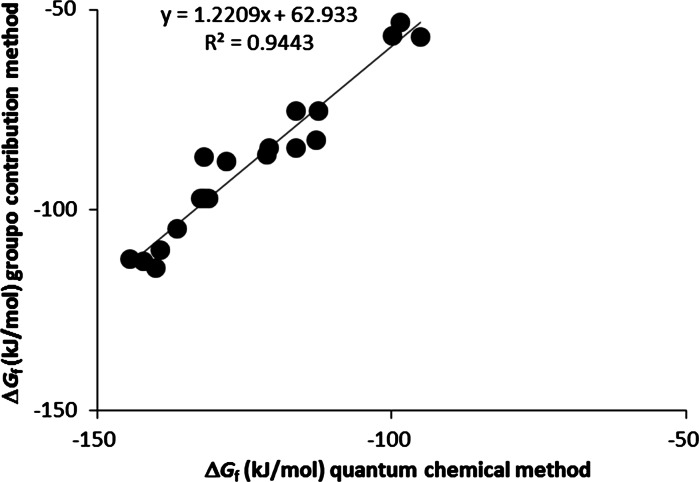
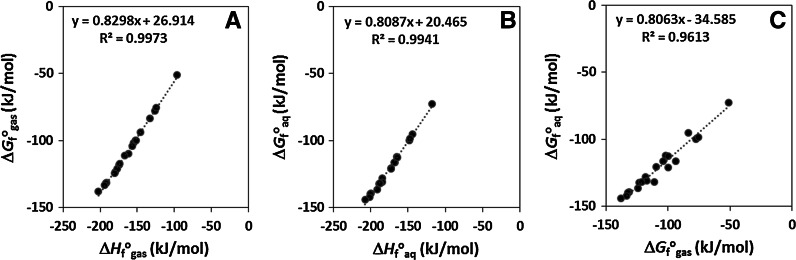
Table 1 shows the ΔH of and ΔG of values for all 19 environmentally relevant chlorophenols for both the gaseous and the aqueous phase. The ΔG of values range between −75.7 and −137.9 kJ/mol for the gas phase and between −95.2 and −144.3 kJ/mol for the aqueous phase. These values are lower than those previously reported (Dolfing and Harrison 1992). There is considerable scatter in plots of the new versus these “old” data (Fig. 1). This is not surprising since quantum mechanical methods incorporate interactions that are not taken into account by group contribution methods which rely on transferability and averaging of properties of a particular functional group. The correlations between ΔH of gas and ΔG of gas, and between ΔH of aq and ΔG of aq (Fig. 2) are excellent, while the correlation between ΔG of gas and ΔG of aq (Fig. 2) is less perfect, which reflects inter alia the influence of molecular structure on solvent solute interactions.
Fig. 1.
Gibbs free energy of formation values of chlorinated phenols in the aqueous phase (1 M; 25 °C). Values obtained with a group contribution method are from Dolfing and Harrison (1992)
Fig. 2.
Relationships between thermodynamic parameters of chlorophenols. a Relationship between ΔG of gas and ΔH of gas, b relationship between ΔG of aq and ΔH of aq, and c relationship between ΔG of aq and ΔG of gas
ΔGof and ΔHof values of brominated phenols
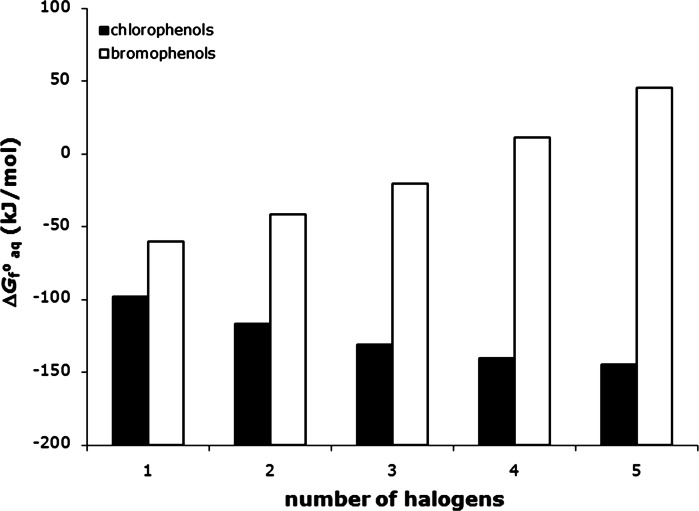
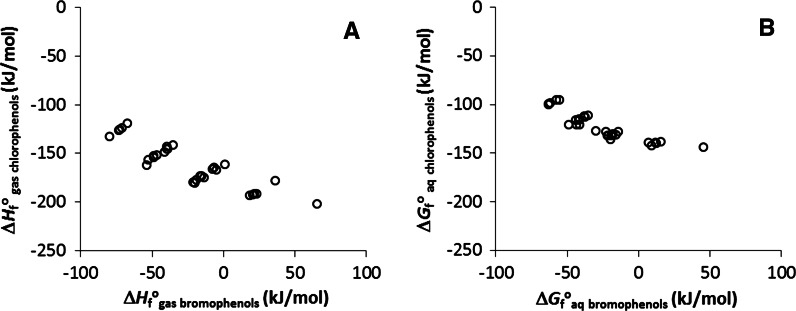
Table 2 shows the ΔH of and ΔG of values for all 19 environmentally relevant bromophenols for both the gas and the aqueous phase (the data for the full series of 31 congeners is provided in SI Table S2. The ΔG of values range between −45.1 and 59.1 kJ/mol for the gas phase and between −63.0 and 45.7 kJ/mol for the aqueous phase. Contrary to the case for chlorophenols ΔG of aq values for bromophenols decrease with increasing degree of halogenation (Fig. 3). Plots of ΔH of gas and ΔG of aq values of chlorinated phenols versus those of bromophenols illustrate that the effect of chloro substituents on the stability of compound is fundamentally different from that of bromo substituents (Fig. 4). This is due to the fact that chlorine is a more electronegative element than bromine, and because bromine is a larger atom, which will introduce steric repulsion (and hence destabilization) with neighboring substituents (be these substituents hydrogens, bromines or OH groups).
Table 2.
Thermodynamic data for brominated phenols under standard conditions (in kJ mol−1)a
| ΔH of gas | ΔG of gas | ΔH of aq | ΔG of aq | ΔH of bgas(exp) | |
|---|---|---|---|---|---|
| Phenol | −96.4 | −51.2 | −118.0 | −72.8 | −96.4 |
| 2-bromophenol | −79.9 | −45.1 | −91.8 | −57.3 | |
| 3-bromophenol | −72.9 | −38.6 | −97.4 | −63.0 | |
| 4-bromophenol | −71.0 | −37.0 | −96.0 | −62.0 | |
| 2,3-dibromophenol | 48.7 | 24.3 | −62.0 | −37.9 | |
| 2,4-dibromophenol | 52.5 | 28.7 | −66.9 | −43.6 | |
| 2,5-dibromophenol | 53.7 | 29.9 | −67.3 | −43.3 | |
| 2,6-dibromophenol | −49.0 | −24.9 | −60.6 | −37.6 | |
| 3,4-dibromophenol | −39.4 | −15.8 | −65.6 | −42.0 | |
| 3,5-dibromophenol | −46.7 | −23.5 | −72.1 | −49.0 | |
| 2,3,4-tribromophenol | −13.3 | 0.1 | −42.5 | −29.7 | |
| 2,3,5-tribromophenol | −21.2 | −7.7 | −34.6 | −21.2 | |
| 2,3,6-tribromophenol | −16.3 | −2.7 | −27.9 | −15.4 | |
| 2,4,5-tribromophenol | −18.9 | −5.6 | −34.0 | −21.6 | |
| 2,4,6-tribromophenol | −19.8 | −6.9 | −30.9 | −19.6 | −0.9 |
| 3,4,5-tribromophenol | −4.7 | 8.5 | −32.0 | −19.0 | |
| 2,3,4,5-tetrabromophenol | 23.4 | 26.8 | 8.2 | 12.0 | |
| 2,3,4,6-tetrabromophenol | 20.5 | 23.7 | 9.9 | 7.3 | |
| 2,3,5,6-tetrabromophenol | 18.7 | 21.9 | 8.1 | 9.3 | |
| Pentabromophenol | 65.6 | 59.1 | 54.8 | 45.7 |
aStandard conditions are: 25 °C; 100 kPa (gas phase) or 1 M (aqueous solution)
bExperimental values are from Linstrom and Mallard (2012)
Fig. 3.
Effect of the number of halogen substituents on the Gibbs free energy of formation of chloro- and bromophenols
Fig. 4.
Correlation between thermodynamic parameters of chlorophenols and bromophenols; a ΔH of gas, and b ΔG of aq
Reliability of calculated standard enthalpy of formation values of halophenols
The experimental standard enthalpies of formation in the gas phase for some halophenols and the parent phenol (Linstrom and Mallard 2012) were used to assess the reliability of our calculated values (Tables 1–2). We note that that agreement with experimental and calculated values for most chlorophenols is close to the stated uncertainty of 4 kJ/mol. However, the discrepancy between calculated and experimental standard enthalpy for 2,4,6-tribromophenol (the only one for which ΔH of had been reported) is much larger and suggests that the measured value (Linstrom and Mallard 2012) needs to be reassessed.
Halogenated phenols, speciation and pH
pH affects the speciation of halophenols (Mun et al. 2008). Dissociation of a halophenol results in the formation of a halophenolate and hence a decrease in the concentration of the halophenol. The degree of dissociation depends on the pH and the pK a value of the halophenol congener (Dolfing et al. 2010). Table 3 (chlorophenols) and SI Table 3 (bromophenols) list the ΔG of values corrected for dissociation at pH 7 for chlorophenols and bromophenols, illustrating that the effect of dissociation is not necessarily negligible. This has implications for the energetics of the dehalogenation reaction. The pK a increases with decreasing degree of halogenation; this implies that deprotonation has a stabilizing effect on highly halogenated compounds. Ortho halophenols are more acidic than meta and para halophenols because of the large inductive effect of the halogen on the vicinal hydroxyl group (Han et al. 2004). For the same reason the acidity of halophenols increases with the number of halogen substitutions (Table 3 and SI Table S3).
Table 3.
Effect of pK a on speciation and ΔG of aq at pH 7 for chlorophenolsa
| ΔG of aq | pK a | αb | ΔG o′f aq c | ||
|---|---|---|---|---|---|
| Phenol | −72.8 | 10.00 | 1.00 | −72.8 | |
| 2-chlorophenol | −95.2 | 8.46 | 0.97 | −95.3 | |
| 3-chlorophenol | −99.9 | 8.92 | 0.99 | −99.9 | |
| 4-chlorophenol | −98.6 | 9.13 | 0.99 | −98.6 | |
| 2,3-dichlorophenol | −112.4 | 7.90 | 0.89 | −112.7 | |
| 2,4-dichlorophenol | −116.3 | 7.94 | 0.90 | −116.5 | |
| 2,5-dichlorophenol | −120.9 | 7.35 | 0.69 | −121.8 | |
| 2,6-dichlorophenol | −112.8 | 6.49 | 0.24 | −116.3 | |
| 3,4-dichlorophenol | −116.3 | 8.43 | 0.96 | −116.4 | |
| 3,5-dichlorophenol | −121.2 | 7.87 | 0.88 | −121.5 | |
| 2,3,4-trichlorophenol | −128.0 | 7.53 | 0.77 | −128.7 | |
| 2,3,5-trichlorophenol | −132.3 | 6.79 | 0.38 | −134.7 | |
| 2,3,6-trichlorophenol | −131.0 | 5.65 | 0.04 | −138.8 | |
| 2,4,5-dichlorophenol | −131.8 | 6.90 | 0.44 | −133.8 | |
| 2,4,6-trichlorophenol | −136.5 | 5.78 | 0.06 | −143.6 | |
| 3,4,5-trichlorophenol | −132.0 | 7.39 | 0.71 | −132.8 | |
| 2,3,4,5-tetrachlorophenol | −139.4 | 6.63 | 0.30 | −142.4 | |
| 2,3,4,6-tetrachlorophenol | −140.0 | 5.11 | 0.01 | −150.8 | |
| 2,3,5,6-tetrachlorophenol | −142.2 | 5.05 | 0.01 | −153.3 | |
| Pentachlorophenol | −144.3 | 4.84 | 0.01 | −156.7 | |
apK a values are taken from Han and Tao (2006); ΔG of aq and are in kJ mol−1
bα is the fraction of chlorinated phenol present as chlorophenols; the fraction present as chlorophenolate is 1−α
cΔG of ’ aq: at pH 7
Redox potentials of halogenated phenols
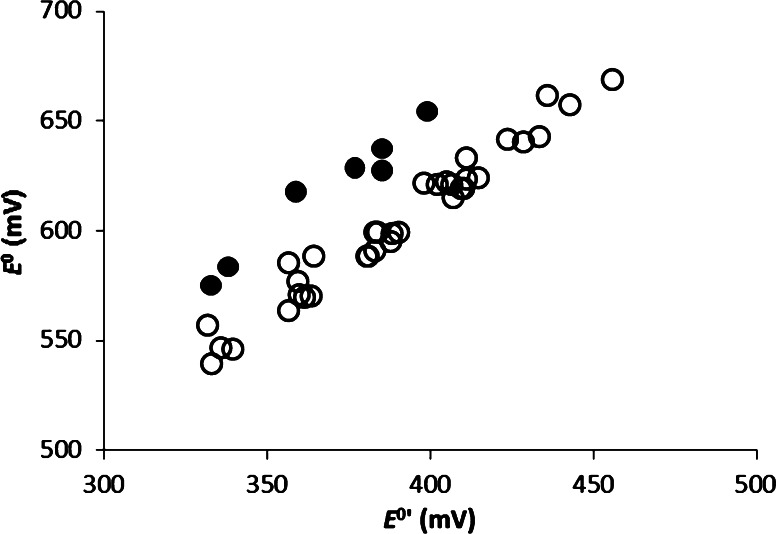
With H2 as electron donor reductive dehalogenation of halophenols is an exergonic process. Under standard conditions the change in Gibbs free energy values for reductive dehalogenation of chlorophenols and bromophenols are in the range of −104 to −129 kJ per mol chloride released and of −112 to −146 kJ per mol bromide released respectively. At pH 7 reductive dehalogenation is significantly more favorable than at pH 0 because protons are generated as reaction product, and because with increasing pH an increasing fraction of the phenols is deprotonated. The two electron reduction potentials naturally follow this drift (Table 4 for chlorophenols; SI Table S4 for bromophenols). A plot of the redox potentials of all redox couples for chlorinated phenols at pH 7 versus the corresponding redox potentials under standard conditions illustrates that compared to pH 0 pH 7 especially favors meta and para dechlorination over ortho dechlorination (Fig. 5). A similar ortho effect was not observed for brominated phenols (data not shown).
Table 4.
Gibbs free energy values and redox potentials for the reductive dechlorination of chlorophenols with H2 (gas) as electron donora
| Reactant | Product | ΔG o | ΔG o′ | E o | E o′ |
|---|---|---|---|---|---|
| Pentachlorophenol | 2,3,4,5-tetrachlorophenol | −126.3 | −157.0 | 655 | 399 |
| 2,3,4,6-tetrachlorophenol | −127.0 | −165.4 | 658 | 443 | |
| 2,3,5,6-tetrachlorophenol | −129.2 | −167.9 | 669 | 456 | |
| 2,3,4,5-tetrachlorophenol | 2,3,4-trichlorophenol | −120.0 | −157.6 | 622 | 402 |
| 2,3,5-trichlorophenol | −124.3 | −163.6 | 644 | 434 | |
| 2,4,5-trichlorophenol | −123.7 | −162.7 | 641 | 429 | |
| 3,4,5-trichlorophenol | −123.9 | −161.7 | 642 | 424 | |
| 2,3,4,6-tetrachlorophenol | 2,3,4-trichlorophenol | −119.3 | −149.1 | 618 | 358 |
| 2,3,6-trichlorophenol | −122.3 | −159.2 | 634 | 411 | |
| 2,4,5-trichlorophenol | −123.0 | −154.2 | 638 | 385 | |
| 2,4,6-trichlorophenol | −127.8 | −164.0 | 662 | 436 | |
| 2,3,5,6-tetrachlorophenol | 2,3,5-trichlorophenol | −121.5 | −152.7 | 629 | 377 |
| 2,3,6-trichlorophenol | −120.1 | −156.7 | 622 | 398 | |
| 2,3,4-trichlorophenol | 2,3-dichlorophenol | −115.7 | −155.3 | 599 | 391 |
| 2,4-dichlorophenol | −119.5 | −159.1 | 619 | 410 | |
| 3,4-dichlorophenol | −119.6 | −159.0 | 619 | 410 | |
| 2,3,5-trichlorophenol | 2,3-dichlorophenol | −111.4 | −149.2 | 577 | 359 |
| 2,5-dichlorophenol | −119.9 | −158.4 | 621 | 407 | |
| 3,5-dichlorophenol | −120.1 | −158.0 | 622 | 405 | |
| 2,3,6-trichlorophenol | 2,3-dichlorophenol | −112.7 | −145.1 | 584 | 338 |
| 2,5-dichlorophenol | −121.2 | −154.3 | 628 | 385 | |
| 2,6-dichlorophenol | −113.1 | −148.8 | 586 | 357 | |
| 2,4,5-trichlorophenol | 2,4-dichlorophenol | −115.8 | −154.0 | 600 | 384 |
| 2,5-dichlorophenol | −120.4 | −159.3 | 624 | 411 | |
| 3,4-dichlorophenol | −115.8 | −153.9 | 600 | 383 | |
| 2,4,6-trichlorophenol | 2,4-dichlorophenol | −111.1 | −144.2 | 575 | 333 |
| 2,6-dichlorophenol | −107.6 | −144.0 | 557 | 332 | |
| 3,4,5-trichlorophenol | 3,4-dichlorophenol | −115.6 | −154.8 | 599 | 388 |
| 3,5-dichlorophenol | −120.5 | −159.9 | 624 | 415 | |
| 2,3-dichlorophenol | 2-chlorophenol | −114.1 | −153.9 | 591 | 383 |
| 3-chlorophenol | −118.8 | −158.5 | 615 | 407 | |
| 2,4-dichlorophenol | 2-chlorophenol | −110.2 | −150.0 | 571 | 363 |
| 4-chlorophenol | −113.7 | −153.4 | 589 | 381 | |
| 2,5-dichlorophenol | 2-chlorophenol | −105.6 | −144.7 | 547 | 336 |
| 3-chlorophenol | −110.2 | −149.3 | 571 | 360 | |
| 2,6-dichlorophenol | 2-chlorophenol | −113.7 | −150.2 | 589 | 364 |
| 3,4-dichlorophenol | 3-chlorophenol | −114.9 | −154.8 | 595 | 388 |
| 4-chlorophenol | −113.7 | −153.5 | 589 | 382 | |
| 3,5-dichlorophenol | 3-chlorophenol | −110.0 | −149.7 | 570 | 362 |
| 2-chlorophenol | Phenol | −108.9 | −148.8 | 564 | 357 |
| 3-chlorophenol | Phenol | −104.3 | −144.2 | 540 | 333 |
| 4-chlorophenol | Phenol | −105.5 | −145.4 | 546 | 339 |
aStandard conditions are 25 °C; solutes at 1 M, H2 gas at 1 atm; ΔG o ′ and E o′ are for pH 7. ΔG values are in kJ reaction−1; E values are in mV
Fig. 5.
Redox potentials for reductive dechlorination of chlorinated phenols under standard conditions (E 0) versus the redox potentials for the same redox couple at pH 7 (E 0′). Black dots indicate redox couples representing ortho dechlorination of double ortho flanked hydroxyl groups
Redox potentials of chlorinated benzoates
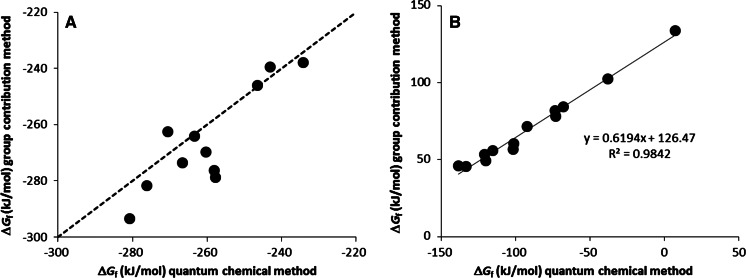
Chlorinated benzoates are the compounds with which Tiedje and co-workers made their seminal observations on microbial dehalogenation (Suflita et al. 1982). (Tang et al. 2010) recently used quantum chemical methods (at the G3XMP2 level) plus a polarizable conductor model to estimate Gibbs free energy of formation values of chlorinated benzoic acids for both the gas and the aqueous phase. At pH 7 chlorinated benzoic acids are essentially fully deprotonated: their pK a values range between −3.3 and 3.6 (Tang et al. 2010). Table 5 lists the Gibbs free energy values of all 19 chlorobenzoate congeners. A plot of these values versus those obtained with Benson’s group contribution method (Dolfing and Harrison 1992) reveals a less than perfect correlation (Fig. 6a) indicating that analogous to the case for halophenols (Fig. 1) quantum chemical methods incorporate electronic interactions that are not taken into account by group contribution methods. The two electron reduction potentials for chlorobenzoic acids (Table 6) range between 560 and 707 mV. The redox potentials for chlorobenzoates at pH 7 range between 285 and 501 mV. These values are systematically different from those reported previously by Tang et al. (2010) who neglected to make the appropriate correction for the H+/H2 redox couple at pH 7.
Table 5.
ΔG of aq (in kJ mol−1) for chlorobenzoates
| Benson’s methoda | Quantum chemical methodb | |
|---|---|---|
| 2-chlorobenzoate | −237.9 | −234.2 |
| 3-chlorobenzoate | −246.0 | −246.5 |
| 4-chlorobenzoate | −239.5 | −243.0 |
| 2,3-dichlorobenzoate | −269.7 | −260.4 |
| 2,4-dichlorobenzoate | −276.4 | −258.0 |
| 2,5-dichlorobenzoate | −287.7 | −257.8 |
| 2,6-dichlorobenzoate | −262.6 | −270.5 |
| 3,4-dichlorobenzoate | −264.2 | −263.3 |
| 3,5-dichlorobenzoate | −273.5 | −266.6 |
| 2,3,4-trichlorobenzoate | −273.4 | |
| 2,3,5-trichlorobenzoate | −293.4 | −280.7 |
| 2,3,6-trichlorobenzoate | −287.4 | |
| 2,4,5-dichlorobenzoate | −271.0 | |
| 2,4,6-trichlorobenzoate | −286.6 | |
| 3,4,5-trichlorobenzoate | −281.6 | −276.1 |
| 2,3,4,5-tetrachlorobenzoate | −275.3 | |
| 2,3,4,6-tetrachlorobenzoate | −296.8 | |
| 2,3,5,6-tetrachlorobenzoate | −296.0 | |
| Pentachlorobenzoate | −299.6 |
Fig. 6.
Gibbs free energy of formation values of a chlorinated benzoates and b chlorinated benzenes in the aqueous phase (1 M; 25 °C). Values obtained with quantum chemical methods are based on Tang et al. (2010) and on Sadowsky et al. (2013) respectively; values obtained with group contribution methods are from Dolfing and Harrison (1992). The dotted line in a is the 1:1 line; a trendline in a (not shown) would have R 2 = 0.76
Table 6.
Gibbs free energy values and redox potentials for the reductive dechlorination of chlorobenzoic acids (at pH 0) and chlorobenzoates (at pH 7) with H2 (gas) as electron donora
| Reactant | Product | ΔG o | ΔG o′ | E o | E o′ |
|---|---|---|---|---|---|
| Pentachloro | 2,3,4,5-tetrachloro | −129.6 | −146.8 | 672 | 347 |
| 2,3,4,6-tetrachlorol | −128.7 | −168.4 | 667 | 458 | |
| 2,3,5,6-tetrachlorol | −126.9 | -167.5 | 658 | 454 | |
| 2,3,4,5-tetrachloro | 2,3,4-trichloro | −124.7 | -169.3 | 646 | 463 |
| 3,4,5-trichloro | −128.0 | -176.6 | 663 | 501 | |
| 2,4,5-trichloro | −128.0 | -166.9 | 663 | 451 | |
| 2,3,5-trichloro | −136.5 | -172.0 | 707 | 477 | |
| 2,3,4,6-tetrachloro | 2,3,4-trichloro | −125.6 | -147.7 | 651 | 352 |
| 2,3,6-trichloro | −124.7 | -161.7 | 646 | 424 | |
| 2,4,5-trichloro | −128.9 | −145.3 | 668 | 339 | |
| 2,4,6-trichloro | −126.2 | −160.9 | 654 | 420 | |
| 2,3,5,6-tetrachloro | 2,3,5-trichloro | −130.7 | −155.9 | 677 | 394 |
| 2,3,6-trichloro | −126.5 | −162.6 | 656 | 428 | |
| 2,3,4-trichloro | 2,3-dichloro | −121.5 | −158.1 | 630 | 405 |
| 2,4-dichloro | −125.1 | −155.8 | 648 | 393 | |
| 3,4-dichloro | −135.5 | −161.1 | 702 | 421 | |
| 2,3,5-trichloro | 2,3-dichloro | −118.2 | −150.7 | 613 | 367 |
| 3,5-dichloro | −122.3 | −148.2 | 634 | 354 | |
| 2,5-dichloro | −133.3 | −157.0 | 691 | 400 | |
| 2,3,6-trichloro | 2,3-dichloro | −122.4 | −144.1 | 634 | 333 |
| 2,6-dichloro | −126.5 | −141.5 | 656 | 319 | |
| 2,5-dichloro | −119.9 | −154.2 | 621 | 385 | |
| 2,4,5-trichloro | 2,4-dichloro | −121.8 | −158.2 | 631 | 406 |
| 2,5-dichloro | −122.3 | −157.9 | 634 | 404 | |
| 3,4-dichloro | −132.2 | −163.5 | 685 | 433 | |
| 2,4,6-trichloro | 2,4-dichloro | −124.5 | −142.6 | 645 | 325 |
| 2,6-dichloro | −118.4 | −155.0 | 614 | 389 | |
| 3,4,5-trichloro | 3,4-dichloro | −123.7 | −158.3 | 641 | 407 |
| 3,5-dichloro | −124.8 | −161.6 | 647 | 424 | |
| 2,3-dichloro | 2-chloro | −118.3 | −145.1 | 613 | 338 |
| 3-chloro | −131.9 | −157.4 | 684 | 401 | |
| 2,4-dichloro | 2-chloro | −114.7 | −147.4 | 594 | 350 |
| 4-chloro | −127.2 | −156.1 | 659 | 395 | |
| 2,5-dichloro | 2-chloro | −114.2 | −147.6 | 592 | 351 |
| 3-chloro | −127.8 | −159.9 | 662 | 415 | |
| 2,6-dichloro | 2-chloro | −120.8 | −135.0 | 626 | 285 |
| 3,4-dichloro | 3-chloro | −117.9 | −154.4 | 611 | 386 |
| 4-chloro | −116.8 | −150.8 | 605 | 368 | |
| 3,5-dichloro | 3-chloro | −116.8 | −151.1 | 605 | 369 |
| 2-chloro | Benzoic acid/benzoate | −121.6 | −154.1 | 630 | 385 |
| 3-chloro | Benzoic acid/benzoate | −108.0 | −141.8 | 560 | 321 |
| 4-chloro | Benzoic acid/benzoate | −109.1 | −145.4 | 565 | 339 |
Redox potentials of halogenated benzenes
Sadowsky et al. (2013) recently used quantum chemical methods at the 6−311+G(3df,2p) level plus the SMD implicit solvation model to estimate thermochemical properties of (poly)halobenzenes. A plot of the aqueous Gibbs free energy of formation values of chlorinated benzenes as obtained with Benson’s group contribution method (Dolfing and Harrison 1992) versus the values obtained by Sadowsky et al. (2013) (Fig. 6b) shows a reasonably good correlation between the two approaches. A large part of the discrepancy between the two data sets appears due to the estimate for benzene itself, without any substituents. The scatter seems less than for the analogous comparison for chlorinated benzenes and benzoates (cf Fig. 1 and Fig. 6a), suggesting that one of the major weaknesses of the group contribution method was the lack of detail of important interactions between the hydroxyl group and the halogen substituents in the case of the chlorophenols and between the carboxyl group and the halogen substituents in the case of the chlorobenzoates. The redox potentials for polyhalogenated benzenes range between 446 and 654 mV at pH 0 and between 239 and 447 mV at pH 7 respectively (Table 7). The latter values are considerably lower than those listed by Sadowsky et al. (2013).
Table 7.
Gibbs free energy values and redox potentials for the reductive dechlorination of chlorinated benzenes with H2 (gas) as electron donora
| Reactant | Product | ΔG 0 | ΔG 0′ | E 0 | E 0′ |
|---|---|---|---|---|---|
| Hexachlorobenzene | Pentachlorobenzene | −126.3 | −166.2 | 654 | 447 |
| Pentachlorobenzene | 1,2,3,4-tetrachlorobenzene | −113.3 | −153.3 | 587 | 380 |
| 1,2,3,5-tetrachlorobenzene | −117.9 | −157.9 | 611 | 404 | |
| 1,2,4,5-tetrachlorobenzene | −118.7 | −158.7 | 615 | 408 | |
| 1,2,3,4-tetrachlorobenzene | 1,2,3-trichlorobenzene | −108.3 | −148.2 | 561 | 354 |
| 1,2,4-trichlorobenzene | −117.1 | −157.0 | 607 | 400 | |
| 1,2,3,5-tetrachlorobenzene | 1,2,3-trichlorobenzene | −103.7 | −143.6 | 537 | 330 |
| 1,3,5-trichlorobenzene | −112.9 | −152.8 | 585 | 378 | |
| 1,2,4,-trichlorobenzene | −112.5 | −152.4 | 583 | 376 | |
| 1,2,4,5-tetrachlorobenzene | 1,2,4-trichlorobenzene | −111.6 | −151.6 | 579 | 372 |
| 1,2,3-trichlorobenzene | 1,2-dichlorobenzene | −106.6 | −146.6 | 552 | 346 |
| 1,3-dichlorobenzene | −112.5 | −152.4 | 583 | 376 | |
| 1,2,4-trichlorobenzene | 1,2-dichlorobenzene | −97.8 | −137.8 | 507 | 300 |
| 1,3-dichlorobenzene | −103.7 | −143.6 | 537 | 330 | |
| 1,4-dichlorobenzene | −103.3 | −143.2 | 535 | 328 | |
| 1,3,5-trichlorobenzene | 1,3-dichlorobenzene | −103.3 | −143.2 | 535 | 328 |
| 1,2-dichlorobenzene | Monochlorobenzene | −101.6 | −141.5 | 526 | 320 |
| 1,3-dichlorobenzene | Monochlorobenzene | −95.7 | −135.7 | 496 | 289 |
| l,4-dichlorobenzene | Monochlorobenzene | −96.2 | −136.1 | 498 | 291 |
| Monochlorobenzene | Benzene | −86.1 | −126.1 | 446 | 239 |
aStandard conditions are 25 °C; solutes at 1 M, H2 gas at 1 atm; ΔG o and E o are for pH 0; and E o′ are for pH 7. ΔG values are in kJ reaction−1; E values are in mV. Values are based on Gibbs free energies in (Sadowsky et al. 2013); see Materials and methods for details
Quantum chemical methods versus Benson’s group contribution method
A comparison of Gibbs free energy of formation values and redox potentials for various classes of halogenated aromatics obtained with Benson’s method versus datasets obtained with quantum chemical methods illustrates that quantum chemical methods allow a level of precision not achievable with group contribution methods. Not only was there a less than perfect agreement between the respective datasets for chlorobenzoates and chlorophenols there was also considerable scatter (Fig. 1 and Fig. 6a). Interestingly, this scatter was far less for the chlorinated benzenes (Fig. 6b). Thus it appears that the group contribution method did especially poor for interactions between the carboxy and the hydroxy group on the one hand and the chloro substituents on the other hand. Another interesting observation is that the consensus Gibbs free energy value for benzene in the aqueous phase in the 1990s (32.0 kcal/mol; 133.9 kJ/mol) (Shock and Helgeson 1990) was considerably higher than the value recently calculated by Sadowsky et al. (1.7 kcal/mol; 7.1 kJ/mol) (2013).
Conclusions
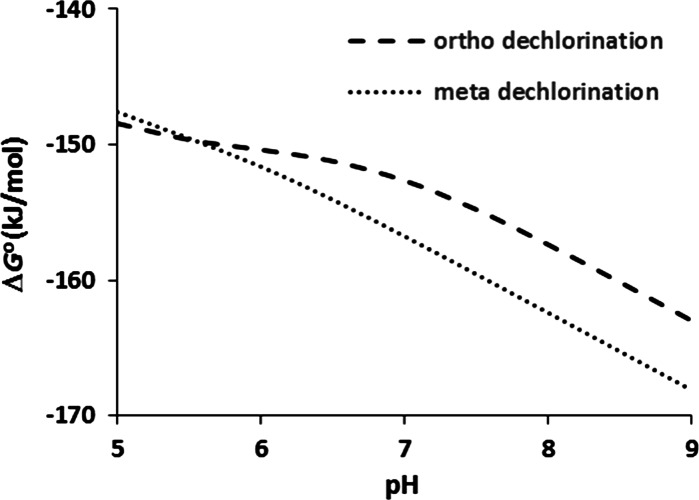
The present comprehensive state-of-the-art dataset of enthalpy and Gibbs free energy of formation values of all chlorinated and brominated phenols makes it possible to calculate change in Gibbs free energy values and redox potentials for reductive dehalogenation of halogenated phenols in the aqueous phase at temperatures other than the standard temperature of 298.15 K, something that was not possible with the previously published dataset, which lacked the required enthalpy values. Other improvements include the incorporation of brominated phenols in the data set, and data for the speciation of halogenated phenols at pH 7. The effect of pH on speciation noticed above may affect which dechlorination reaction is energetically most favorable. Figure 7 shows an example where dechlorination of 2,3,5,6-tetrachlorophenol at the ortho position is more favorable than dechlorination at the meta position at pH 5 but not at pH 7.
Fig. 7.
Effect of pH on the change in Gibbs free energy for the reductive dechlorination of 2,3,5,6-tetrachlorophenol to 2,3,5- and 2,3,6-trichlorophenol
The data presented here illustrate that halogenated aromatics are excellent electron acceptors: the carbon-halogen bond represents a considerable source of energy. Developing technologies to harness the energy involved seems a worthwhile challenge, for example in microbial fuel cells (Huang et al. 2012). Microorganisms per se have already developed this ability (Leys et al. 2013). The classical example of microbial energy generation by organohalide respiration was with 3-chlorobenzoate as electron acceptor (Dolfing and Tiedje 1987; Dolfing 1990; Mohn and Tiedje 1990). Since then a wide variety of organohalide respiring bacteria have been identified, including organisms that can grow with halogenated benzenes, benzoates and phenols as electron acceptor (Hug et al. 2013). The present data can be used to calculate the amount of energy that is potentially available to these organisms under in situ conditions. Another potential use of the present data-set is in evaluating the dehalogenation pattern of polyhalogenated aromatics. It has been observed for various classes of halogenated compounds, including chlorophenols, that the change in Gibbs free energy values can be used to rationalize dechlorination patterns, with the energetically most favorable reactions the most likely to occur (Dolfing and Harrison 1993; Dolfing 1995, 2003), although here some restrictions apply: microorganisms and their metabolic machinery do not necessarily follow the thermodynamically predicted pathways, steric and other chemical factors may also play a role (Dolfing 2003). A case in point is the often demonstrated preferred microbial ortho-dehalogenation of chlorophenols (e.g. Adrian et al. 2003; Utkin et al. 1995), which is contrary to what would be expected if the organisms would preferentially use the thermodynamically most favorable pathway. Thus dehalogenation of chlorophenols by dehalogenases in Dehalococcoides strain DCB1 and 195 and Desulfitobacterium dehalogenans JW/IU-DC1 is under kinetic control, in contrast to dehalogenation of chlorophenols by vitamin B12s which appears to be under thermodynamic control. The latter conclusion was drawn in 1995 based on the thermodynamic data available at that time (Dolfing 1995) and still holds when the data presented in Table 4 are used for the evaluation.
Electronic supplementary material
Below is the link to the electronic supplementary material.
Acknowledgments
This study was supported by the Biotechnology and Biological Sciences Research Council UK (BB/K003240/2) and a Faculty Research Fellowship from Newcastle University. Parts of the results were presented at 246th National Meeting of the American Chemical Society in Indianapolis; constructive comments from the audience and three anonymous reviewers are gratefully acknowledged.
References
- Adrian L, Hansen SK, Fung JM, Görisch H, Zinder SH. Growth of Dehalococcoides strains with chlorophenols as electron acceptors. Environ Sci Technol. 2003;41:2318–2323. doi: 10.1021/es062076m. [DOI] [PubMed] [Google Scholar]
- Baboul AG, Curtiss LA, Redfern PC, Raghavachari K. Gaussian-3 theory using density functional geometries and zero-point energies. J Chem Phys. 1999;110:7650–7657. doi: 10.1063/1.478676. [DOI] [Google Scholar]
- Cox JD, Wagman DD, Medvedev VA. CODATA key values for thermodynamics. New York: Hemipshere Publishing; 1989. [Google Scholar]
- Dolfing J. Reductive dechlorination of 3-chlorobenzoate is coupled to ATP production and growth in an anaerobic bacterium, strain DCB-1. Arch Microbiol. 1990;153:264–266. doi: 10.1007/BF00249079. [DOI] [PubMed] [Google Scholar]
- Dolfing J. Regiospecificity of chlorophenol reductive dechlorination by vitamin B12s. Appl Environ Microbiol. 1995;61:2450–2451. doi: 10.1128/aem.61.6.2450-2451.1995. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Dolfing J. Thermodynamic considerations for dehalogenation. In: Häggblom MM, Bossert ID, editors. Dehalogenation: Microbial processes and environmental applications. Boston: Kluwer; 2003. pp. 89–114. [Google Scholar]
- Dolfing J, Harrison BK. The Gibbs free energy of formation of halogenated aromatic compounds and their potential role as electron acceptors in anaerobic environments. Environ Sci Technol. 1992;26:2213–2218. doi: 10.1021/es00035a021. [DOI] [Google Scholar]
- Dolfing J, Harrison BK. Redox and reduction parameters to predict the degradation pathway of chlorinated benzenes in anaerobic environments. FEMS Microbiol Ecol. 1993;13:23–30. doi: 10.1111/j.1574-6941.1993.tb00047.x. [DOI] [Google Scholar]
- Dolfing J, Tiedje JM. Growth yield increase linked to reductive dechlorination in a defined 3-chlorobenzoate degrading methanogenic coculture. Arch Microbiol. 1987;149:102–105. doi: 10.1007/BF00425073. [DOI] [PubMed] [Google Scholar]
- Dolfing J, Xu A, Head IM. Anomalous energy yields in thermodynamic calculations: importance of accounting for pH-dependent organic acid speciation. ISME J. 2010;4:463–464. doi: 10.1038/ismej.2010.14. [DOI] [PubMed] [Google Scholar]
- Farai M, de Vos WM, Smidt H. Exploiting the ecogenomics toolbox for environmental diagnostics of organohalide-respiring bacteria. Trends Biotechnol. 2010;28:308–316. doi: 10.1016/j.tibtech.2010.03.005. [DOI] [PubMed] [Google Scholar]
- Field JA, Sierra-Alvarez R. Microbial degradation of chlorinated phenols. Rev Environ Sci Bio/Technol. 2008;7:211–241. doi: 10.1007/s11157-007-9124-5. [DOI] [Google Scholar]
- Frisch MJ, Trucks GW, Schlegel HB, Scuseria GE, Robb MA, Cheeseman JR, Zakrzewski VG, Montgomery JA Jr, Stratmann RE, Burant JC, Dapprich S, Millam JM, Daniels AD, Kudin KN, Strain MC, Farkas O, Tomasi J, Barone V, Cossi M, Cammi R, Mennucci B, Pomelli C, Adamo C, Clifford S, Ochterski J, Petersson GA, Ayala PY, Cui Q, Morokuma K, Malick DK, Rabuck AD, Raghavachari K, Foresman JB, Cioslowski J, Ortiz JV, Stefanov BB, Liu G, Liashenko A, Piskorz P, Komaromi I, Gomperts R, Martin RL, Fox DJ, Keith T, Al–Laham MA, Peng CY, Nanayakkara A, Gonzalez C, Challacombe M, Gill PMW, Johnson B, Chen W, Wong MW, Andres JL, Gonzalez C, Head-Gordon M, Replogle ES, Pople JA (2003) Gaussian 03, Revision E1. Gaussian Inc., Pittsburgh, PA, USA
- Han J, Tao F-M. Correlations and predictions of pKa values of fluorophenols and bromophenols using hydrogen-bonded complexes with ammonia. J Phys Chem A. 2006;110:257–263. doi: 10.1021/jp052824e. [DOI] [PubMed] [Google Scholar]
- Han J, Deming RL, Tao F-M. Theoretical study of molecular structures and properties of the complete series of chlorophenols. J Phys Chem A. 2004;108:7736–7743. doi: 10.1021/jp047923r. [DOI] [Google Scholar]
- Heidrich ES, Curtis TP, Dolfing J. Determination of the internal chemical energy of wastewater. Environ Sci Technol. 2011;45:827–832. doi: 10.1021/es103058w. [DOI] [PubMed] [Google Scholar]
- Huang L, Chai X, Quan X, Logan BE, Chen G. Reductive dechlorination and mineralization of pentachlorophenol in biocathode microbial fuel cells. Bioresour Technol. 2012;111:167–174. doi: 10.1016/j.biortech.2012.01.171. [DOI] [PubMed] [Google Scholar]
- Huang L, Shi Y, Wang N, Dong Y. Anaerobic/aerobic conditions and biostimulationfor enhanced chlorophenols degradation in biocathode microbial fuel cells. Biodegradation. 2014 doi: 10.1007/s10532-014-9686-1. [DOI] [PubMed] [Google Scholar]
- Hug LA, Maphosa F, Leys D, Löffler FE, Smidt H, Edwards EA, Adrian L. Overview of organohalide-respiring bacteria and a proposal for a classification system for reductive dehalogenases. Phil Trans R Soc B. 2013;368:20120322. doi: 10.1098/rstb.2012.0322. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Leys D, Adrian L, Smidt H. Organohalide respiration: microbes breathing chlorinated molcecules. Phil Trans R Soc B Biol Sci. 2013;368:20120316. doi: 10.1098/rstb.2012.0316. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Linstrom PJ, Mallard WG (2012) NIST Chemistry WebBook, NIST Standard Reference Database Number 69. National Institute of Standards and Technology, Gaithersburg http://webbook.nist.gov. Accessed 09 Apr 2014
- Marenich AV, Cramer CJ, Truhlar DG. Universal solvation model based on solute electron density and a continuum model of the solvent defined by the bulk dielectric constant and atomic surface tensions. J Phys Chem B. 2009;113:6378–6396. doi: 10.1021/jp810292n. [DOI] [PubMed] [Google Scholar]
- McCarty PL. Breathing with chlorinated solvents. Science. 1997;276:1521–1522. doi: 10.1126/science.276.5318.1521. [DOI] [PubMed] [Google Scholar]
- Mohn WW, Tiedje JM. Strain DCB-1 conserves energy for growth from reductive dechlorination coupled to formate oxidation. Arch Microbiol. 1990;153:267–271. doi: 10.1007/BF00249080. [DOI] [PubMed] [Google Scholar]
- Mun CH, Ng WJ, He J. Acidogenic sequencing batch reactor start-up procedures for induction of 2,4,6-trichlorophenol dechlorination. Water Res. 2008;42:1675–1683. doi: 10.1016/j.watres.2007.10.019. [DOI] [PubMed] [Google Scholar]
- Novak I. Ab initio vs molecular mechanics thermochemistry: homocubanes. J Chem Inf Comp Sci. 2004;44(3):903–906. doi: 10.1021/ci0300285. [DOI] [PubMed] [Google Scholar]
- Sadowsky D, McNeill K, Cramer CJ. Thermochemical factors affecting the dehalogenation of aromatics. Environ Sci Technol. 2013;47:14194–14203. doi: 10.1021/es404033y. [DOI] [PubMed] [Google Scholar]
- Shock EL, Helgeson HC. Calculation of the thermodynamic and transport properties of aqueous species at high pressures and temperatures: standard partial molal properties of organic species. Geochim Cosmochim Acta. 1990;54:915–945. doi: 10.1016/0016-7037(90)90429-O. [DOI] [Google Scholar]
- Strycharz SM, Gannon SM, Boles AR, Nevin KP, Franks AE, Lovley DR. Reductive dechlorination of 2-chlorophenol by Anaeromyxobacter dehalogenans with an electrode serving as the electron donor. Environ Microbiol Rep. 2010;2:289–294. doi: 10.1111/j.1758-2229.2009.00118.x. [DOI] [PubMed] [Google Scholar]
- Stumm W, Morgan JJ. Aquatic Chemistry. 3. New York: Wiley; 1996. [Google Scholar]
- Suflita JM, Horowitz A, Sheldon DR, Tiedje JM. Dehalogenation—a novel pathway for the anaerobic biodegradation of haloaromatic compounds. Science. 1982;218:1115–1117. doi: 10.1126/science.218.4577.1115. [DOI] [PubMed] [Google Scholar]
- Tang A, Wang L, Zhou R. Gibbs energies of formation of chlorinated benzoic acids and benzoates and application to their reductive dechlorination. J Mol Struct (THEOCHEM) 2010;960:31–39. doi: 10.1016/j.theochem.2010.08.021. [DOI] [Google Scholar]
- Thauer RK, Jungermann K, Decker K. Energy conservation in chemotrophic anaerobic bacteria. Bacteriol Rev. 1977;41:100–180. doi: 10.1128/br.41.1.100-180.1977. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Utkin I, Dalton DD, Wiegel J. Specificity of reductive dehalogenation of substituted ortho-chlorophenols by Desulfitobacterium dehalogenans JW/IU-DC1. Appl Environ Microbiol. 1995;61:346–351. doi: 10.1128/aem.61.1.346-351.1995. [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.