Abstract
According to recent reviews, the question of how trophic interactions may affect evolutionary responses to climate change remains unanswered. In this modelling study, we explore the evolutionary dynamics of thermal and plant–herbivore interaction traits in a warming environment. We find the herbivore usually reduces adaptation speed and persistence time of the plant by reducing biomass. However, if the plant interaction trait and thermal trait are correlated, herbivores can create different coevolutionary attractors. One attractor has a warmer plant thermal optimum, and the other a colder one compared with the environment. A warmer plant thermal strategy is given a head start under warming, the only case where herbivores can increase plant persistence under warming. Persistence time of the plant under warming is maximal at small or large thermal niche width. This study shows that considering trophic interactions is necessary and feasible for understanding how ecosystems respond to climate change.
Keywords: trophic interactions, coevolutionary response, temperature change, thermal niche, persistence, adaptation
1. Introduction
We have an urgent need to understand and predict the response of individual species as well as whole communities and ecosystems to global change. However, the most commonly employed methods for predicting the response of species to climate change do not explicitly incorporate all fundamental ecological and evolutionary processes that may be major determinants of species responses to climate change [1]. Evolutionary adaptation may be the only way for species to persist when faced with climate change [2], although it may not be rapid enough for some taxa to keep up with the current pace of environmental change [3]. Although many theoretical models have considered evolution in extinction scenarios [4,5], they have typically neglected species interactions that can be critically important to estimate extinction risk [6].
There is increasing evidence that species interactions play a role and should be taken into account in understanding responses to climate change [7,8], especially evolutionary responses. Competition can both increase and decrease the rate of adaptation depending on conditions [9,10]. According to recent reviews, we know almost nothing about how other types of species interactions (e.g. predator–prey, host–pathogen, mutualism) will affect evolutionary responses to climate change [11], but tracking a changing climate for a predator should be especially difficult because the predator must track both climate and its prey in evolutionary trait space. Warming has been shown to increase extinction of higher trophic levels [12,13]. Thus, adding even just a single trophic level to current models could lead to many unexplored and probably important effects [14].
Despite the importance of trophic interactions, we are aware of only a few modelling studies [15–17] that have included them in the context of evolutionary responses to a changing environment. One study found that predators help its prey adapt and extend the time to extinction by up to 40% owing to stronger selection pressure on maladapted individuals [15]. That study did not include variable population size. Although evidence of evolution to climate change is still scarce [18,19], we know that the rate and direction of evolution [20] and evolutionary rescue [21] depend on initial population size. In general, the addition of a herbivore to a producer-resource system can have ecological effects by changing the ecological attractor (location and stability of equilibrium, especially species abundances) of the system. The addition of a herbivore can also have eco-evolutionary effects through mechanisms such as the direction of selection (may act as a roadblock), strength of selection, rate of adaptation (mediated through ecological effects on abundance) [10], evolutionary attractor (location and stability of evolutionary equilibrium) and additional trait axes subject to selection [22].
The niches (location and width) of interacting species should determine species responses to one another (such as when adding a herbivore to a system) and to environmental changes. Intuitively, we expect a large niche width to lead to the longest persistence time for a species in a changing environment as it may buffer a species from the environmental change so it does not have to adapt through evolution or it may maintain a large enough population size to eventually allow adaptation. However, some studies show an intermediate niche width to lead to longest persistence of a single species [5,23], while a study that includes species interactions shows the highest extinction rates at intermediate niche width [10]. Furthermore, many previous studies do not consider the interaction of niche width and rate of environmental change, probably because they consider a small abrupt shift in the environment, rather than under what conditions all populations can catch a constantly moving optimum. We build on prior work incorporating evolution under climate change [24] and focus on the quantitative influence of the trophic interaction on the persistence of a plant and herbivore.
2. Material and methods
(a). Model
We use an ecosystem model similar to previous studies that included trophic interactions and coevolution [25,26]. The simple ecosystem model includes equations for quantities of inorganic nutrient resource R, plant biomass P and herbivore biomass H:
| 2.1 |
| 2.2 |
| 2.3 |
where I represents inorganic nutrient input; q, the inorganic nutrient loss rate; k, the nutrient uptake rate; l, the plant conversion efficiency of nutrients into plants; m, the loss rate of the plant; a, the grazing rate of the herbivore on the plant; b, the herbivore conversion efficiency of plant into herbivore; and d, the loss rate of the herbivore.
We incorporate mechanistic thermal optima curves that have been described for many taxa on earth [27]. Briefly, we assume each phenotype has a thermal optimum where it has the highest potential growth rate, and a range (width) of temperatures where it exhibits positive growth. This creates an implicit trade-off because at a certain temperature some phenotypes perform better than others. Across species, maximum growth rate increases with temperature [28] (i.e. ‘Hotter is Better’ [29]). We assume there exists an upper bound on performance set by the Eppley curve. Thus, we combine individual thermal niches with an increasing upper bound to mathematically describe trade-offs and constraints in phenotypic thermal niche space [30].
We model two traits for both species. One trait, z, determines the thermal optimum for a species (figure 1a). The functional form of the nutrient uptake rate is
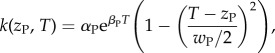 |
2.4 |
where T is the temperature of the environment; wP, the thermal niche width; αP, the Eppley curve coefficient and βP, the Eppley curve exponent for the plant [30] (see the electronic supplementary material, appendix S1 and figure S1). The more similar the trait zP is to the temperature of the environment T, the higher the uptake rate of the plant.
Figure 1.

(a) Plot of k, plant nutrient uptake rate, versus plant thermal trait, zP. The environmental temperature T = 10 and the thermal niche width wP = 8. (b) Plot of a(sP, sH, zH, T), the grazing rate, a Gaussian trait matching function so that the more similar the species trait values are, the stronger the trophic interaction. This plot assumes herbivore traits sH = zH = T = 10 and allows plant interaction trait sP to vary to determine grazing rate a(sP, sH, zH, T). Here, amax = 0.2, σ = 3.
The second trait, s, determines the interaction between the herbivore and plant species (figure 1b). We use a Gaussian-type function of trait matching [31,32] and combine it with the herbivore thermal niche to form the grazing rate [33]:
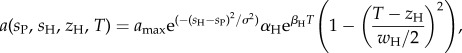 |
2.5 |
where amax is the maximum grazing rate; σ, the interaction kernel width; wH, the thermal niche width; αH, the Eppley curve coefficient; and βH, the Eppley curve exponent for the herbivore. The more similar the interaction traits (sP, sH) of the two species are to one another, the higher the grazing rate of the herbivore on the plant.
(b). Analysis
Details on evolutionary analysis methods are in the electronic supplementary material, appendix S1. Briefly, evolutionary simulations are based on the canonical equation of adaptive dynamics [34]. The evolutionary equation for the thermal trait of the plant, zP takes the form
| 2.6 |
where the mutation rate μP and population size P (proportional to biomass) control the pace of evolutionary change for the plant and 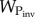 is the fitness of a mutant with trait
is the fitness of a mutant with trait  so that
so that 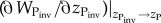 represents the local fitness gradient. We use this form for each trait (s,z) of both species (P,H).
represents the local fitness gradient. We use this form for each trait (s,z) of both species (P,H).
We use analytical methods to detail under what conditions the addition of a herbivore helps or hinders the adaptation of the plant in a warming environment (see the electronic supplementary material, appendix S1). We use numerical simulations to support our analytical results. Conducted simulations were: (i) evolution in a static environment to steady state, and then (ii) evolution from that steady state in response to environmental change. Most theoretical studies consider a single instantaneous perturbation or a fluctuating environment with a constant mean. We consider non-steady-state conditions [9], a linear change in temperature T for a fixed time period, designed to mimic some of the current climate projections for the near future [35]. We vary the amount of temperature change, ΔT, from 0 to 4° (we also consider more extreme values) to obtain qualitatively different outcomes.
The relationship between traits can be described by their genetic correlation γ [36]. Previous studies that included species interactions with evolution have considered the special case in which the genetic correlation is unity [15,32]. Calls have been made for future work that addresses cases in which the trait involved in the species interaction is distinct, but genetically correlated, with the trait responding to environmental change [15]. We consider two limiting cases regarding the relationship between traits for both species. These bracket the spectrum of possible scenarios, i.e. perfectly correlated traits and completely independent traits. Under the perfectly correlated assumption, traits s = z for both species. The traits in our model could become correlated like this if the interaction between two species is related to phenology, e.g. over certain temperature ranges, the species are more likely to be present, growing and interacting. Wild parsnips and parsnip webworms are a coevolutionary plant–herbivore system with strong genetic correlations in traits related to phenology and interaction strength [37]. For completely independent traits, s and z are allowed to evolve without a direct influence on each other. This adds complexity and additional analysis, and also allows us to be more general.
We ran evolutionary simulations to t = 108 time steps in a constant environment, and all change stopped (or a cycle had been reached). We then classified the evolutionary equilibrium, hereafter singular strategy ss, using graphical, semi-analytical and numerical methods described in section ‘Evolutionary analysis methods’ in the electronic supplementary material, appendix S1. We calculated the persistence time as the time to extinction, when the biomass was below a threshold set at 10−6.
Parameters for the temperature dependence of vital rates αj and βj were measured on phytoplankton [38] to improve the Eppley curve. Parameters for thermal niche widths were measured on diverse taxa including arthropods, amphibians, reptiles, molluscs, fishes [39], plants [40] and phytoplankton [41], and we focus on a reduced range of values to account for variability in temperature that reduces the limits of the thermal niche [42].
Parameters for resource supply, maximum grazing rate, mortality rates and conversion efficiencies were varied to match biomass ratios of natural systems. Biomass ratio is the key that determines evolutionary rates and outcomes such as persistence. Heterotrophic to autotrophic biomass ratios range from greater than 1 in very unproductive lakes to less than 4.5 × 10−4 in tropical forests [43]. For the results we present, we use herbivore to plant biomass ratio 0.07–0.4, which also depends on the evolutionary outcomes of the species. Thus, our values correspond to a typical productive plankton ecosystem and many terrestrial ecosystems with fast biomass turnover.
Evolutionary simulations were conducted over a wide range of parameter values and we present results representative of the larger parameter space (see the electronic supplementary material, appendix S1 and table S1, for presented parameter range). For each set of parameters, we repeated simulations for two different initial conditions, with values of species thermal traits both above and below the temperature of the environment, T, to check the influence of initial conditions. We changed parameters systematically to take slices through the system of important parameters wp, ΔT, μ and to explore how our system may represent aquatic and terrestrial systems. Although we see some influence of particular values of parameters on quantitative outcomes such as the exact persistence time, we consistently observe similar qualitative behaviour.
3. Results
A summary of our results on how the different assumptions affect the coevolutionary outcome in a static and changing environment is presented in table 1.
Table 1.
Summary of assumptions and results, a road map to effects of herbivore on the ecosystem. (Assumptions are: H?, with or without the herbivore in the system, and traits corr., whether the thermal and interaction traits are correlated or not. Static environment outcomes are: trait zP = T?, whether the plant thermal trait matches the temperature of the environment or not, and evol. attract. ss, when thermal traits do not match the temperature of the environment, whether they locate at the high or low evolutionary attractor (ss singular strategy). Changing environment characterizes the effect of the herbivore on plant adaptation to warming, all else being equal, the net effect on adaptation rate and persistence time of the plant.)
| assumptions |
static environment |
changing environment | ||
|---|---|---|---|---|
| H? | traits corr. | trait zP = T? | evol. attract. ss | H effect on P adaptation to warming |
| no H | yes | faster adaptation, longer persistence | ||
| +H | slower adaptation, shorter persistence | |||
| no | yes | H never helps P | ||
| yes | yes | H never helps P | ||
| no | low ss | H never helps P | ||
| high ss | H can help P by providing head start | |||
(a). Static environment
We find distinct evolutionary attractors (singular strategies, ss) in a static environment, hence we classify them into separate cases (see ‘Cases descriptions’ in the electronic supplementary material, appendix S1 for detailed analysis). For uncorrelated traits, the thermally related traits zP and zH always match the temperature T of the environment (table 1, trait zP = T) and the traits describing the interaction, sP and sH, are driven by sensitivity to initial conditions. For correlated traits, we find scenarios where the species traits do and do not match the environmental temperature (table 1, trait zP≠T). Instead, the traits of both species can be some distance from the environmental temperature.
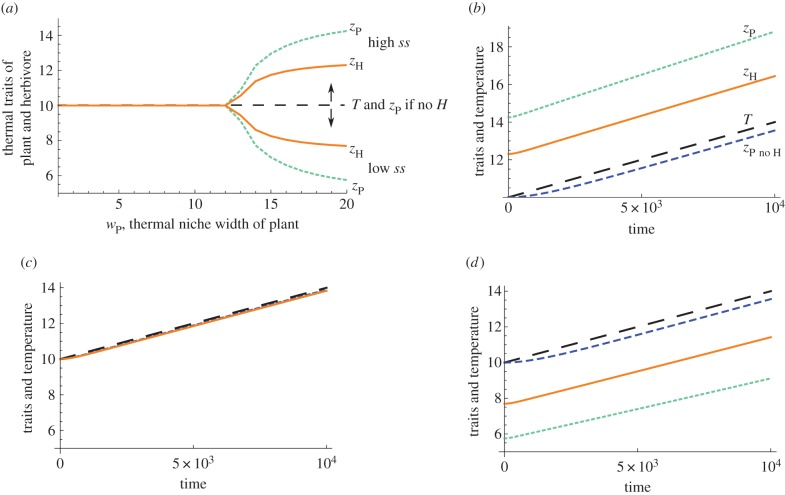
By fixing the thermal niche width of the herbivore wH at a constant value, we can examine the role of the relative thermal niche width of the plant wP on the location of the singular strategies ss in the static environment, as well as persistence time and biomass dynamics of both species in a changing environment. For all values of the plant thermal niche width wP, the thermal traits match the temperature of the environment (T = 10) if there is no herbivore or traits are not correlated (figure 2a). For correlated traits, over a large range of plant thermal niche width wP, the traits match the temperature of the environment (T = 10). However, if plant thermal niche width wP is sufficiently greater than herbivore thermal niche width wH (depending on interaction kernel width σ), then the traits do not match the temperature of the environment T and instead locate at the high or low singular strategy ss (table 1, evol. attract. ss). Which singular strategy they are attracted to depends on whether the initial plant trait value starts above (high) or below (low) the temperature of the environment T. The two different singular strategies create different responses when the environment changes.
Figure 2.
(a) Effect of plant thermal niche width wP on the ss, singular strategy thermal trait values for the plant and herbivore. If the herbivore is not in the system, the singular strategy ss plant thermal trait zP is always to match the temperature of the environment T (dashed line). For uncorrelated traits, thermal traits zP = zH = T for all values of plant thermal niche width wP (interaction traits sP and sH depend on initial conditions). For correlated traits (sP = zP and sH = zH) and high values of plant thermal niche width wP, there exists both a high-trait value singular strategy ss and a low-trait value ss for both the herbivore (solid line) and plant (dotted line). Parameters are: T = 10, wH = 8 and σ = 3. (b–d) Trait values and temperature through time in a warming environment for different values of plant thermal niche width wP for correlated traits: (b) wP = 20 high ss, (c) wP = 10 and (d) wP = 20 low ss. The large dashed line is temperature, plant trait without the herbivore is the small dashed line, herbivore trait is the solid line, plant trait with the herbivore is the dotted line. Parameters are σ = 3, wH = 8, ΔT = 4, μ = 10−1 and time interval t = 104. (Online version in colour.)
(b). Warming environment
(i). Comparing dynamics under a warming regime
Species interactions can not only create different outcomes in a constant environment (table 1, trait zP = T?, evol. attract. ss), but can also create different targets in a changing environment. A plant species in isolation or with uncorrelated traits starts at and attempts to track the environmental temperature T (figure 2c), but with the addition of a herbivore and if traits are correlated, may track targets that are displaced from T (figure 2b,d) and therefore may not be evolving to track simply their optimal temperature.
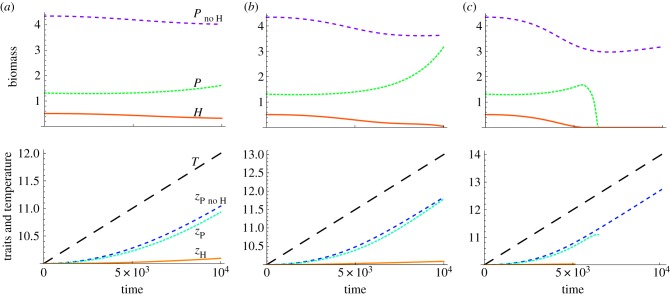
For low temperature change and when species are attempting to match their optimal temperature, the coupled plant–herbivore system and the plant without the herbivore are able to track the environmental temperature well enough to persist in the time interval (figure 3a). While difficult to discern in this figure, warming often has a positive effect on top trophic level biomass, at least initially, owing to Eppley's relationship of increasing growth rate with temperature. However, we consistently see that with increasing rate of temperature change, persistence time of both species declines owing to imperfect tracking. At the highest temperature change depicted in figure 3c, the herbivore goes extinct, followed by the plant when the herbivore is present initially in the system. Interestingly, the plant is able to track the environmental temperature T under this highest temperature change when the herbivore is not in the system. We see similar patterns when traits are not correlated. The outcome can be either eventual extinction or trait matching to the moving optimum for intermediate values of mutation rate and temperature change (electronic supplementary material, appendix S1 and figures S2 and S3). Within this interesting range of intermediate adaptation, we present the effect of thermal niche width and increasing temperature change on persistence time for both trophic levels.
Figure 3.
Top: herbivore biomass (solid line) and plant biomass with H (dotted line) and without H (small dashed line) through time in a warming environment. Bottom: trait values and temperature through time in a warming environment. Large dashed line is temperature, solid line is herbivore trait, dotted line is plant trait with H and small dashed line is plant trait without H. Temperature change rates are for (a) ΔT = 2, (b) ΔT = 3 and (c) ΔT = 4. Traits are correlated here and parameters are σ = 3, wP = 3, wH = 8, μ = 10−3.5 and time interval t = 104. (Online version in colour.)
(ii). Persistence time
Persistence time is determined by an interaction between plant thermal niche width wP and temperature change ΔT, figure 4. Persistence of the plant species with and without the herbivore in the system declines with increasing temperature change as we also observed in figure 3. Along a gradient of plant thermal niche width wP, persistence time decreases at intermediate thermal niche width of the plant, with no herbivore in the system (figure 4, small dashed line). With the herbivore in the system and for correlated traits, we can observe a multimodal relationship if we consider higher values of temperature change (figure 4b). The patterns at small plant thermal niche width wP are similar, persistence time of both species can initially decrease and then increase with thermal niche width of the plant. However, at larger plant thermal niche width wP and for correlated traits, other factors caused by the trophic interaction become important. For plant thermal niche width wP > 12, persistence time can increase or decrease depending on which singular strategy ss one examines. Paradoxically, a larger thermal niche may decrease persistence time, which leads to the non-intuitive observation that a small plant thermal niche width wP could lead to a relatively long persistence time for both species. However, the overall pattern remains: shorter persistence times for the plant at intermediate values of plant thermal niche width wP.
Figure 4.
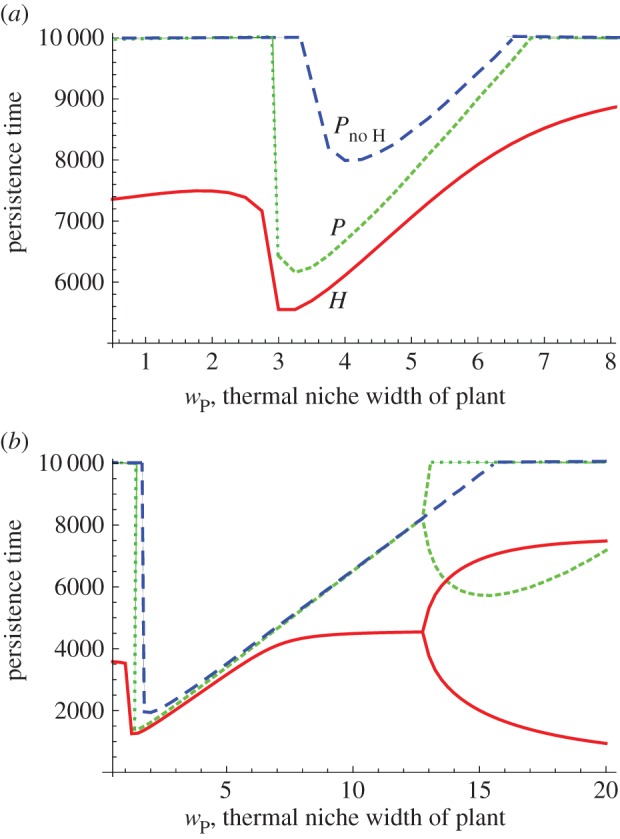
Effect of wP, thermal niche width of plant and ΔT temperature change on persistence time for (a) ΔT = 4 and (b) ΔT = 8. Persistence time plotted versus plant thermal niche width wP for the herbivore (solid line), plant with H (dotted line) and plant without H (small dashed line). Traits are correlated here, therefore, at high values of plant thermal niche width wP with H, there exists both a high-trait value singular strategy ss and a low-trait value ss for the plant and herbivore. Parameters are: σ = 3 and μ = 10−3.5. (Online version in colour.)
Biomass strongly affects adaptation and persistence time. Plant biomass at equilibrium is controlled by the herbivore (electronic supplementary material, appendix S1, equation 6) and varies with plant thermal niche width wP for correlated traits (electronic supplementary material, appendix S1 and figure S4). The plant has a decreased persistence time in the presence of the herbivore except for under strict conditions (electronic supplementary material, appendix S1 section ‘Shift from equilibrium under warming’). The conditions are: (i) the plant thermal niche width is large enough to create alternative singular strategies, and (ii) initial conditions are such that the system evolves to the high-trait value singular strategy.
4. Discussion
A central challenge in ecology is predicting how ecosystems will respond to global change. Our results show trophic interactions modify the response and speed of adaptation to a change in environmental conditions. The ecosystem architecture, controlled by the nature of the trophic interaction, values, and correlations of species traits, also affects the existence of and ability to reach different peaks on the adaptive landscape. In particular, the addition of the trophic interaction has a strong negative effect on density and rate of adaptation of the plant. The trophic interaction can create different coevolutionary attractors in a static environment. Multiple attractors occur under large thermal niche widths, create different targets and trajectories in a changing environment and increase or decrease plant and herbivore persistence. Intermediate plant thermal niche width generally leads to low-persistence time of the plant with and without the herbivore.
Empirical evidence shows that higher trophic levels can control the abundance of lower trophic levels immediately below them [44] and at levels further below them through trophic cascades [45]. Responses to climate change are highly variable between species [46]. Species interactions that are not taken into account may be one of the causes of this variability. We have shown that species interactions affect both ecological and evolutionary responses to warming, therefore, it is important to understand both in order to predict a species response to a changing climate, particularly in plant–herbivore systems [47]. Climate change affects co-occurring species differently, which may disrupt trophic interactions [8]. Even within thermal tolerance ranges of the interacting species, these temperature changes are a potent mechanism to alter trophic interactions [48]. Furthermore, the response of predators and prey to temperature can be context dependent, which means that impacts of future climate change on trophic interactions may be difficult to predict [49].
(a). Persistence
Persistence time is influenced by the amount of temperature change (figures 3 and 4). Plant–herbivore systems may appear rather stable to small amounts of temperature change, for example one to two degrees in figure 3a, but larger amounts can lead to extinction, for example four degrees in figure 3c. The amount of temperature change that is tolerated before extinction occurs is highly dependent on a number of uncertain parameters such as mutation rate, so figure 3 should be taken more as a qualitative illustration than a quantitative prediction.
We have shown that persistence time in a changing environment can critically depend on measurable physiological parameters such as species-specific thermal niche width. The thermal niche width wP that leads to the longest persistence of the species depends on mutation and temperature change rates, and is not always a large thermal niche width, multimodal patterns of species persistence time with thermal niche width exist for correlated traits. We predict an intermediate thermal niche width is worst in regards to persistence of a species, conflicting with previous studies of single species [5,23] but in agreement with a previous study including species interactions [10]: high extinction rates at intermediate width of the carrying capacity function, equivalent to the thermal niche width in our model. We and another study [10] assume monomorphic populations, differing from the assumption that at low niche widths much of the population is at low fitness owing to population variance [5,23].
Both with and without the herbivore in the system, under a realistic climate warming scenario and small plant relative thermal niche width, we expect to see high-persistence time of both species because it creates strong selection to track the environment. For an intermediate plant relative thermal niche width, we generally see low-persistence time and biomass for both species because intermediate thermal niche width relaxes selection and the population lags too far behind the temperature to adequately respond before extinction. Perhaps counterintuitively, for a large plant relative thermal niche width, we can see either low- or high-persistence time for both species, depending on initial conditions. In addition, a large thermal niche width can buffer a species from the environmental change because the species never has to adapt through evolutionary change or the species maintains a large enough population size to eventually allow adaptation. This suggests the possibility of two distinct strategies to deal with environmental change.
Higher trophic levels can be important to the adaptive capacity of the ecosystem, they may release lower trophic levels and allow faster adaptation. A large herbivore population or very adaptive herbivore (high mutation rate) reduces persistence time of a plant, adding a carnivore to the system could mediate that. Owing to differences in biomass ratios between terrestrial and aquatic ecosystems, we expect different adaptation rates and patterns of extinction depending on the balance between biomass and interaction strengths controlled by coevolution.
(b). Assumptions, predictions and future work
Evidence is increasing that some species may not be able to simply move to a better location to alleviate climate stress [50]. In this situation, species must adapt to local conditions through evolution or other mechanisms of adaptation. Many species can disperse but are also facing habitat loss and the interaction of these processes additively or synergistically with evolutionary adaptation complicates predictions [14]. We use a slightly different framework than many previous studies examining evolutionary rescue [4,5]. Specifically, we do not explicitly consider dispersal and intraspecific variation but instead consider interacting species, thus our study adds a facet to studies on evolutionary responses to a changing environment.
Our model and results are rather general allowing us to tune some parameters to an aquatic or terrestrial system. In the situation of a very unproductive lake, when all else is equal, the plant goes extinct before the herbivore owing to the high herbivore to plant biomass ratio, although the herbivore will eventually go extinct without the plant (result not shown). In more productive aquatic systems and many terrestrial systems, as our presented parameters more closely represent, the herbivore will go extinct first as we illustrate in figures 3 and 4. Low biomass turnover coupled with including dead as well as living tissue for the autotrophs skews the biomass ratio for many forests. The ratio for a forest would probably be closer to the biomass ratio we present in the results (0.07–0.4) if we consider just live tissue [43].
We assume biomass is a good proxy for the number of reproductive units. This is valid not only for plankton but also many plant species. Plants are typically modular organisms whose reproduction is proportional to number of modules, where modules represent basic demographic units of plants such as tillers, shoots and rosettes [51]. Biomass is highly correlated with module density [52].
Other fundamental differences between aquatic and terrestrial systems are the time scales for different processes. Evolutionary processes have been shown to occur on very short time scales in a variety of systems [53]. For example, plankton have a fast generation time and can be expected to adapt more quickly to temperature change. However, tree species with a small niche width and a slow adaptation rate are more likely to go extinct.
How can the predictions from our model be tested? Our results are more likely to apply to an ecosystem with strong herbivore pressure and herbivore–plant specialization or low species diversity. Data on thermal niche widths of the species is readily available [39] however, critically, one must compare if the producers have smaller or larger thermal niche widths than their herbivores and we are not aware that this comparison has been made. Additionally, data on mutation rates are available and are size, taxon and perhaps even temperature dependent [54]. The interaction of multiple global change drivers on plant–herbivore interactions has been shown to be complex and will require multiple study approaches at multiple scales [55]. We are aware of only one theoretical study on the interaction of temperature and resources in a food chain [56], therefore future work should consider the interaction of temperature and resources, with evolution.
Supplementary Material
Acknowledgements
The authors thank the Centre for Biodiversity Theory and Modelling (CBTM) laboratory members for helpful discussion, Bart Haegeman, Tomas Revilla and several anonymous reviewers for suggestions.
Funding statement
This work was supported by the TULIP Laboratory of Excellence (ANR-10-LABX-41).
References
- 1.Leroux S, Larrivee M, Boucher-Lalonde V, Hurford A, Zuloaga J, Kerr J, Lutscher F. 2013. Mechanistic models for the spatial spread of species under climate change. Ecol. Appl. 23, 815–828. ( 10.1890/12-1407.1) [DOI] [PubMed] [Google Scholar]
- 2.Hoffmann A, Sgro CM. 2011. Climate change and evolutionary adaptation. Nature 470, 479–483. ( 10.1038/nature09670) [DOI] [PubMed] [Google Scholar]
- 3.Quintero I, Wiens JJ. 2013. Rates of projected climate change dramatically exceed past rates of climatic niche evolution among vertebrate species. Ecol. Lett. 16, 1095–1103. ( 10.1111/ele.12144) [DOI] [PubMed] [Google Scholar]
- 4.Pease C, Lande R, Bull J. 1989. A model of population growth, dispersal and evolution in a changing environment. Ecology 70, 1657–1664. ( 10.2307/1938100) [DOI] [Google Scholar]
- 5.Huey RB, Kingsolver J. 1993. Evolution of resistance to high temperature in ectotherms. Am. Nat. 142, S21–S46. ( 10.1086/285521) [DOI] [Google Scholar]
- 6.Palamara G, Delius G, Smith M, Petchey O. 2013. Predation effects on mean time to extinction under demographic stochasticity. J. Theor. Biol. 334, 61–70. ( 10.1016/j.jtbi.2013.06.007) [DOI] [PubMed] [Google Scholar]
- 7.Tylianakis J, Didham R, Bascompte J, Wardle D. 2008. Global change and species interactions in terrrestrial ecosystems. Ecol. Lett. 11, 1351–1363. ( 10.1111/j.1461-0248.2008.01250.x) [DOI] [PubMed] [Google Scholar]
- 8.Post E. 2013. Ecology of climate change: the importance of biotic interactions. Princeton, NJ: Princeton University Press. [Google Scholar]
- 9.Johansson J. 2008. Evolutionary responses to environmental changes: how does competition affect adaptation. Evolution 62, 421–435. ( 10.1111/j.1558-5646.2007.00301.x) [DOI] [PubMed] [Google Scholar]
- 10.Osmond M, de Mazancourt C. 2012. How competition affects evolutionary rescue. Phil. Trans. R. Soc. B 368, 1–13. ( 10.1098/rstb.2012.0085) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Lavergne S, Mouquet N, Thuiller W, Ronce O. 2010. Biodiversity and climate change: integrating evolutionary and ecological responses of species and communities. Annu. Rev. Ecol. Evol. Syst. 41, 321–350. ( 10.1146/annurev-ecolsys-102209-144628) [DOI] [Google Scholar]
- 12.Petchey O, McPhearson P, Casey T, Morin PJ. 1999. Environmental warming alters food-web structure and ecosystem function. Nature 402, 69–72. ( 10.1038/47023) [DOI] [Google Scholar]
- 13.Fussman K, Schwarzmuller F, Brose U, Jousset A, Rall B. 2014. Ecological stability in response to warming. Nat. Clim. Change 4, 206–210. ( 10.1038/nclimate2134) [DOI] [Google Scholar]
- 14.Urban M, de Meester L, Velland M, Stoks R, Vanoverbeke J. 2011. A crucial step toward realism: responses to climate change from an evolving metacommunity perspective. Evol. Appl. 5, 154–167. ( 10.1111/j.1752-4571.2011.00208.x) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Jones A. 2008. A theoretical quantitative genetic study of negative ecological interactions and extinction times in changing environments. BMC Evol. Biol. 8, 119 ( 10.1186/1471-2148-8-119) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Moya-Laraño J, Verdeny-Vilalta O, Rowntree J, Melguizo-Ruiz N, Montserrat M, Laiolo P. 2012. Climate change and eco-evolutionary dynamics in food webs. Adv. Ecol. Res. 47, 1–80. ( 10.1016/B978-0-12-398315-2.00001-6) [DOI] [Google Scholar]
- 17.Northfield T, Ives A. 2013. Coevolution and the effects of climate change on interacting species. PLOS Biol. 11, e1001685 ( 10.1371/journal.pbio.1001685) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Gienapp P, Teplitsky C, Alho J, Mills J, Merila J. 2008. Climate change and evolution: disentangling environmental and genetic responses. Mol. Ecol. 17, 167–178. ( 10.1111/j.1365-294X.2007.03413.x) [DOI] [PubMed] [Google Scholar]
- 19.Merila J. 2012. Evolution in response to climate change: in pursuit of the missing evidence. Bioessays 34, 811–818. ( 10.1002/bies.201200054) [DOI] [PubMed] [Google Scholar]
- 20.Turcotte M, Reznick DN, Hare J. 2013. Experimental test of an eco-evolutionary dynamic feedback loop between evolution and populaiton density in the green peach aphid. Am. Nat. 181, S46–S57. ( 10.1086/668078) [DOI] [PubMed] [Google Scholar]
- 21.Bell G, Gonzalez A. 2011. Adaptation and evolutionary rescue in metapopulations experiencing environmental deterioration. Science 332, 1327–1330. ( 10.1126/science.1203105) [DOI] [PubMed] [Google Scholar]
- 22.Etterson J, Shaw R. 2001. Constraint to adaptive evolution in response to global warming. Science 294, 151–154. ( 10.1126/science.1063656) [DOI] [PubMed] [Google Scholar]
- 23.Burger R, Lynch M. 1995. Evolution and extinction in a changing environment: a quantitative-genetic analysis. Evolution 49, 151–163. ( 10.2307/2410301) [DOI] [PubMed] [Google Scholar]
- 24.Norberg J, Urban MC, Velland M, Klausmeier CA, Loeuille N. 2012. Eco-evolutionary responses of biodiversity to climate change. Nat. Clim. Change 2, 747–751. ( 10.1038/nclimate1588) [DOI] [Google Scholar]
- 25.Loeuille N, Loreau M, Ferriere R. 2002. Consequences of plant-herbivore coevolution on the dynamics and functioning of ecosystems. J. Theor. Biol. 217, 369–381. ( 10.1006/jtbi.2002.3032) [DOI] [PubMed] [Google Scholar]
- 26.Mellard JP, Ballantyne IVF. 2014. Conflict between dynamical and evolutionary stability in simple ecosystems. Theor. Ecol. 7, 273–288. ( 10.1007/s12080-014-0217-9) [DOI] [Google Scholar]
- 27.Angilletta M. 2009. Thermal adaptation: a theoretical and empirical synthesis. New York, NY: Oxford University Press. [Google Scholar]
- 28.Eppley RW. 1972. Temperature and phytoplankton growth in the sea. Fishery Bull. 70, 1063–1085. [Google Scholar]
- 29.Angilletta M, Huey RB, Frazier M. 2010. Thermodynamic effects on organismal performance: is hotter better? Physiol. Biochem. Zool. 83, 197–206. ( 10.1086/648567) [DOI] [PubMed] [Google Scholar]
- 30.Norberg J. 2004. Biodiversity and ecosystem functioning: a complex adaptive systems approach. Limnol. Oceanogr. 49, 1269–1277. ( 10.4319/lo.2004.49.4_part_2.1269) [DOI] [Google Scholar]
- 31.Gavrilets S. 1997. Coevolutionary chase in exploiter-victim systems with polygenic characters. J. Theor. Biol. 186, 527–534. ( 10.1006/jtbi.1997.0426) [DOI] [PubMed] [Google Scholar]
- 32.Calcagno V, Dubosclard M, de Mazancourt C. 2010. Rapid exploiter-victim coevolution: the race is not always to the swift. Am. Nat. 176, 198–211. ( 10.1086/653665) [DOI] [PubMed] [Google Scholar]
- 33.Rall B, Brose U, Hartvig M, Kalinkat G, Schwarzmuller F, Vucic-Pestic O, Petchey O. 2012. Universal temperature and body-mass scaling of feeding rates. Phil. Trans. R. Soc. B 367, 2923–2934. ( 10.1098/rstb.2012.0242) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Dieckmann U, Law R. 1996. The dynamical theory of coevolution: a derivation from stochastic ecological processes. J. Math. Biol. 34, 579–612. ( 10.1007/BF02409751) [DOI] [PubMed] [Google Scholar]
- 35.IPCC. 2007. Climate change 2007: synthesis report. An assessment of the intergovernmental panel on climate change. Technical report.
- 36.Lande R. 1979. Quantitative genetic analysis of multivariate evolution, applied to brain: body size allometry. Evolution 33, 402–416. ( 10.2307/2407630) [DOI] [PubMed] [Google Scholar]
- 37.Berenbaum M, Zangerl A, Nitao J. 1986. Constraints on chemical coevolution: wild parsnips and the parsnip webworm. Evolution 40, 1215–1228. ( 10.2307/2408949) [DOI] [PubMed] [Google Scholar]
- 38.Bissinger JE, Montagnes D, Sharples J, Atkinson D. 2008. Predicting marine phytoplankton maximum growth rates from temperature: improving on the eppley curve using quantile regression. Limnol. Oceanogr. 53, 487–493. ( 10.4319/lo.2008.53.2.0487) [DOI] [Google Scholar]
- 39.Sunday J, Bates A, Dulvy N. 2011. Global analysis of thermal tolerance and latitude in ectotherms. Proc. R. Soc. B 278, 1823–1830. ( 10.1098/rspb.2010.1295) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Honek A, Martinkova Z, Lukas J, Dixon A. 2014. Plasticity of the thermal requirements of exotherms and adaptation to environmental conditions. Ecol. Evol. 4, 3103–3112. ( 10.1002/ece3.1170) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Thomas M, Kremer C, Klausmeier CA, Litchman E. 2012. A global pattern of thermal adaptation in marine phytoplankton. Science 338, 1085–1088. ( 10.1126/science.1224836) [DOI] [PubMed] [Google Scholar]
- 42.Estay SA, Lima M, Bozinovic F. 2014. The role of temperature variability on insect performance and population dynamics in a warming world. Oikos 123, 131–140. ( 10.1111/j.1600-0706.2013.00607.x) [DOI] [Google Scholar]
- 43.del Giorgio PA, Gasol JM. 1995. Biomass distribution in freshwater plankton communities. Am. Nat. 146, 135–152. ( 10.1086/285790) [DOI] [Google Scholar]
- 44.Sih A, Crowly P, McPeek MA, Petranka J, Strohmeier K. 1985. Predation, competition, and prey communities: a review of field experiments. Annu. Rev. Ecol. Syst. 16, 269–311. ( 10.1146/annurev.es.16.110185.001413) [DOI] [Google Scholar]
- 45.Pace ML, Cole JJ, Carpenter SR, Kitchell JF. 1999. Trophic cascades revealed in diverse ecosystems. Trends Ecol. Evol. 14, 483–488. ( 10.1016/S0169-5347(99)01723-1) [DOI] [PubMed] [Google Scholar]
- 46.Moritz C, Agudo R. 2013. The future of species under climate change: resilience or decline? Science 341, 504–508. ( 10.1126/science.1237190) [DOI] [PubMed] [Google Scholar]
- 47.Louthan A, Doak D, Goheen J, Palmer T, Pringle R. 2013. Climatic stress mediates the impacts of herbivory on plant population structure and components of individual fitness. J. Ecol. 101, 1074–1083. ( 10.1111/1365-2745.12090) [DOI] [Google Scholar]
- 48.Grigaltchik V, Ward A, Seebacher F. 2012. Thermal acclimation of interactions: differential responses to temperature change alter predator-prey relationship. Proc. R. Soc. B 279, 4058–4064. ( 10.1098/rspb.2012.1277) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 49.Ewald NC, Hartley SE, Steward AJA. 2013. Climate change and trophic interactions in model temporary pond systems: the effects of high temperature on predation rate depend on prey size and density. Freshw. Biol. 58, 2481–2493. ( 10.1111/fwb.12224) [DOI] [Google Scholar]
- 50.Buckley L, Tewksbury J, Deutsch C. 2013. Can terrestrial ectotherms escape the heat of climate change by moving?. Proc. R. Soc. B 280, 20131149 ( 10.1098/rspb.2013.1149) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 51.Schmid B. 1990. Some ecological and evolutionary consequences of modular organization and clonal growth in plants. Evol. Trends Plants 4, 25–34. [Google Scholar]
- 52.Marquard E, Weigelt A, Roscher C, Gubsch M, Lipowsky A, Schmid B. 2009. Positive biodiversity-productivity relationship due to increased plant diversity. J. Ecol. 97, 696–704. ( 10.1111/j.1365-2745.2009.01521.x) [DOI] [Google Scholar]
- 53.Hairston NG, Jr, Ellner SP, Geber MA, Yoshida T, Fox JA. 2005. Rapid evolution and the convergence of ecological and evolutionary time. Ecol. Lett. 8, 1114–1127. ( 10.1111/j.1461-0248.2005.00812.x) [DOI] [Google Scholar]
- 54.Stegen J, Ferrière R, Enquist B. 2011. Evolving ecological networks and the emergence of biodiversity patterns across temperature gradients. Proc. R. Soc. B 279, 1051–1060. ( 10.1098/rspb.2011.1733) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 55.de Sassi C, Lewis O, Tylianakis J. 2012. Plant-mediated and nonadditve effects of two global change drivers on an insect herbivore community. Ecology 93, 1892–1901. ( 10.1890/11-1839.1) [DOI] [PubMed] [Google Scholar]
- 56.Binzer A, Guill C, Brose U, Rall B. 2012. The dynamics of food chains under climate change and nutrient enrichment. Phil. Trans. R. Soc. B 367, 2935–2944. ( 10.1098/rstb.2012.0230) [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.