Figure 2.
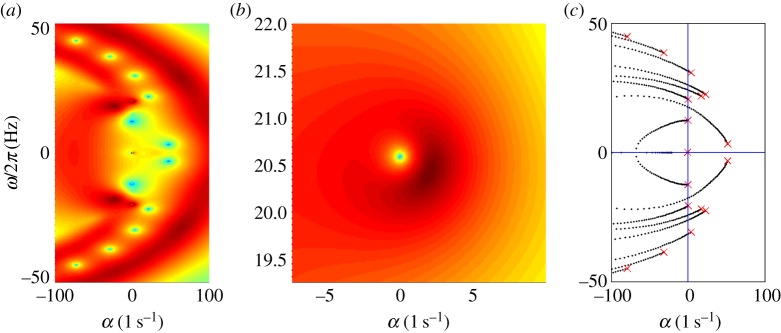
(a) Eigenvalues from the assumed exponential solution of the sliding-controlled flagellar model (equation (3.14)) with sliding permitted at the base (case 1; parameters in table 1). Images of the log magnitude of the determinant, ln|D(σ)| (arb. units), are shown as a function of σ = α + iω. Eigenvalues (characteristic exponents) are found at local minima (blue) of |D(σ)|. Unstable modes have α = Re(σ) > 0. (b) Expanded view of panel (a) shows the eigenvalue at σ = i2π · 20.6 corresponding to a 20.6 Hz periodic mode. (c) Eigenvalues from the weighted-residuals method: paths of eigenvalues σ = α + iω are shown in the complex plane as 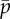 is varied (
is varied (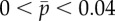 ). Other parameters are as in table 1. The red ‘x’ symbols denote the eigenvalues at the final value
). Other parameters are as in table 1. The red ‘x’ symbols denote the eigenvalues at the final value 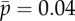 . The eigenvalues in panel (c) closely match the minima of |D(σ)| in panel (a). (Online version in colour.)
. The eigenvalues in panel (c) closely match the minima of |D(σ)| in panel (a). (Online version in colour.)
