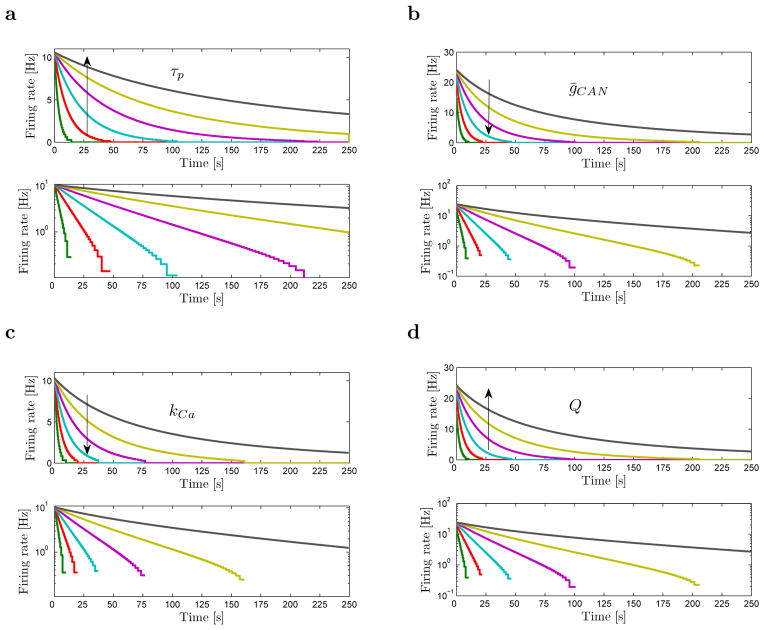
Figure 4.

Simulated firing rate decays exponentially with various time constant for different values of calcium clearance time constant τ_p, conductivity of the CAN current channels ḡ_CAN, calcium influx during spikes k_Ca and the charge required for spike generation Q. Top plot on each of the four figures shows firing rate in linear scale, while bottom plots show firing rate in log scale. Approximately straight lines in the log-plots confirm that the overall decays can be well approximated as exponential. For low firing rates (< 1Hz), the decay deviates from exponential due to increased inaccuracy in the time constant estimation. In each plot, the parameter manipulated is displayed. The arrow shows the ordering corresponding to increasing values of the parameter. a. Influence of τ_p on the firing rate. Following values of τ_p were used: [1.4, 1.9, 2.1, 2.2, 2.25, 2.28]s. b. Influence of ḡ_CAN on the firing rate. The following values of ḡ_CAN were used: $[1.4,1.9,2.1,2.2,2.25,2.28]{10}^{-4}\frac{\mathit{mho}}{{cm}^2}$. c. Influence of k_Ca on the firing rate. Following values of k_Ca were used: [0.5, 1.4, 1.9, 2.1, 2.2, 2.25, 2.28]/25. Calcium concentration is given as unitless such that initial calcium concentration is normalized to value 1. d. Influence of Q on the firing rate. To change Q we changed specific membrane capacitance c_m, so it took the following values: 1/[0.5, 1.4, 1.9, 2.1, 2.2, 2.25, 2.28]μF/cm².