Figure 2.

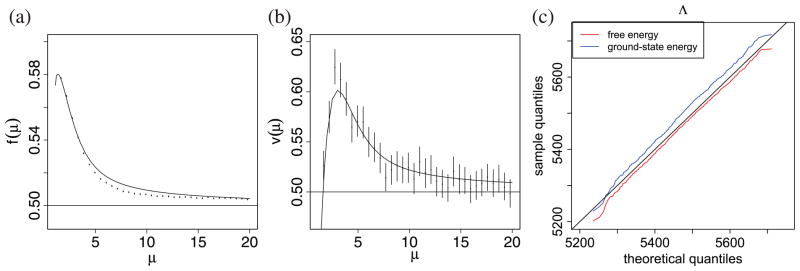
Comparison of asymptotic theory to finite-n simulations. We generated networks from the stochastic block model with varying average degree μ (n = 10⁴, k = 2, γ₁ = γ₂ = 1/2, ω₁₁ = ω₂₂, and ω₁₂/ω₁₁ = ω₂₁/ω₂₂ = 0.15) and computed Λ (the log-likelihood ratio) for each graph. Parts (a) and (b) show n⁻¹E(Λ) and n⁻¹var(Λ), comparing 95% bootstrap confidence intervals (over 10³ replicates) to the asymptotic formulas (respectively f(μ) from (15) and v(μ) from (17)). Part (c) compares the distribution of Λ from 10⁴ replicates, all with μ = 3, to a Gaussian with the theoretical mean and variance.