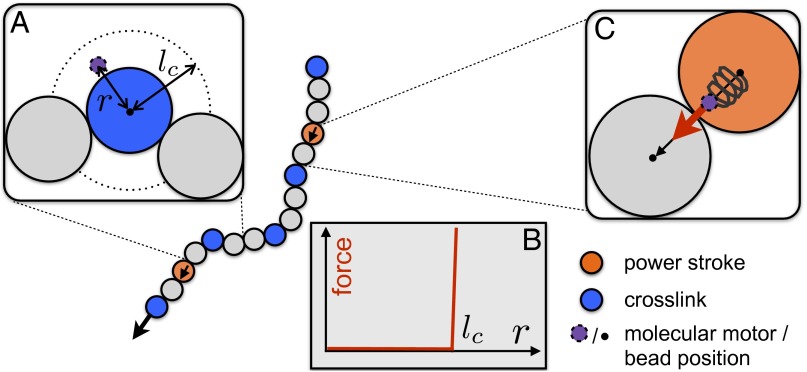
Fig. 2.

Computational model for actin filaments propelled by molecular motors. We consider a bead-spring model in which each bead is bound to a molecular motor that either forms a cross-link (blue beads) or is engaged in executing a power stroke (orange beads). In addition, the filament is subject to thermal forces. (A) During some fraction ${\tau }_{\times }$ of its chemo-mechanical cycle, the molecular motor acts as a cross-link. We assume a highly nonlinear force-extension relation for the linker domain as illustrated in B. The linker domain acts as a rigid link if the distance r between the bead position and the motor anchoring point exceeds a certain value $l_c$ (referred to as capturing length); otherwise, the force exerted on the bead is zero. (C) During the power stroke phase, the linker domain of the motor acts like a spring, releasing stored elastic energy as it snaps forward parallel to the local tangent of the filament’s contour. For simplicity, we assume that the total number of motors attached to a filament, $m_b$, stays constant. For a typical experiment of motor density, $c=1.5\cdot {10}^3{\mu \text{m}}^{-2}$, we estimate $m_b\approx 20$ (SI Materials and Methods).