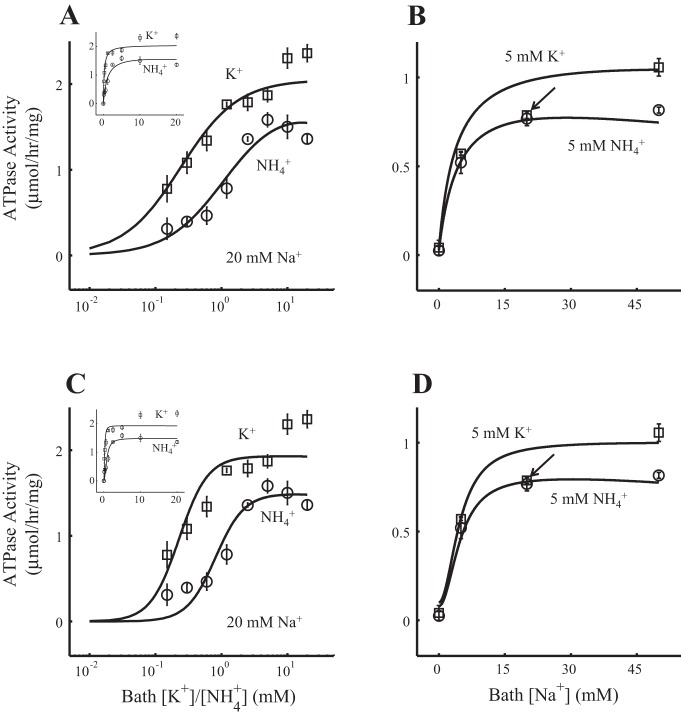
Fig. 2.

H⁺(Na⁺)-K⁺(NH₄⁺)-ATPase model fit to the experimental data. Each flux is multiplied by the corresponding J_max^exp shown in Table 4. A and B: the 1H⁺(1Na⁺):1K⁺(1NH₄⁺)-per-ATP stoichiometry model. C and D: the 2H⁺(2Na⁺):2K⁺(2NH₄⁺)-per-ATP stoichiometry model. A and C: ATPase activity as a function of bath K⁺ concentration ([K⁺]; squares, Experiment 1 in Table 1) or NH₄⁺ concentration ([NH₄⁺]; circles, Experiment 2 in Table 1), both with bath Na⁺ concentration ([Na⁺]) fixed to 20 mM. For a better appreciation of the fittings, the x-axis is presented in the log₁₀ scale and does not include the data corresponding to [K⁺] and [NH₄⁺] equal to zero. The fittings in the linear scale are shown in the top left corner and include the data corresponding to [K⁺] and [NH₄⁺] equal to zero. B and D: ATPase activity as a function of bath [Na⁺] with [K⁺] fixed to 5 mM (squares, Experiment 3 in Table 1) or [NH₄⁺] fixed to 5 mM (circles, Experiment 4 in Table 1). The arrow identifies the square that corresponds to [Na⁺] at 20 mM (see results). The R² value of the model fit to the experimental data was 0.95 for the 1H⁺(1Na⁺)-1K⁺(1NH₄⁺)-ATPase model and 0.93 for the 2H⁺(2Na⁺)-2K⁺(2NH₄⁺)-ATPase model. The resulting H⁺-to-K⁺ net flux ratio under the conditions in H⁺-to-K⁺ net flux ratio in Table 1 was 0.0099 for the 1H⁺(1Na⁺)-1K⁺(1NH₄⁺)-ATPase model and 0.011 for the 2H⁺(2Na⁺)-2K⁺(2NH₄⁺)-ATPase model.