Fig. 4.

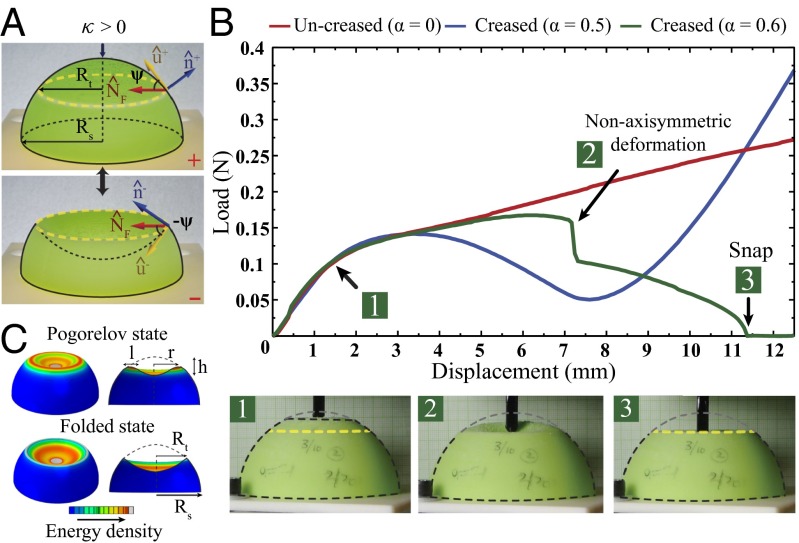
Folding of spheres ($K>0$). (A) Spherical shell of thickness t, radius $R_s$, and a crease radius $R_t$ in bistable states (+, −). Vectors (${\widehat{\mathbf{u}}}^{\pm },{\widehat{\mathbf{n}}}^{\pm },{\widehat{\mathbf{N}}}_{\mathbf{F}}$, and $\widehat{\mathbf{t}}$) and parameters ($R_t,R_s$, and ψ) used in this study are overlaid on the experimental sample. (B) Spherical shells with crease radius $\alpha =R_t/R_s=0$ (red), 0.5 (blue), and 0.6 (green), and over all radius $R_s=35\hspace{0.5em}\text{mm}$. For the smaller value of α, no stable snap is observed indicating monostability, whereas for larger value of α a nonaxisymmetric deformation, followed by a stable snap, occurs under indentation (Movie S3). (C) Schematics for the Pogorelov state of a deformed spherical shell, with representative ridge (of size $\sim l$) at a radius r; and the folded state of a creased spherical shell (radius $R_s$), with a crease radius $R_t$.