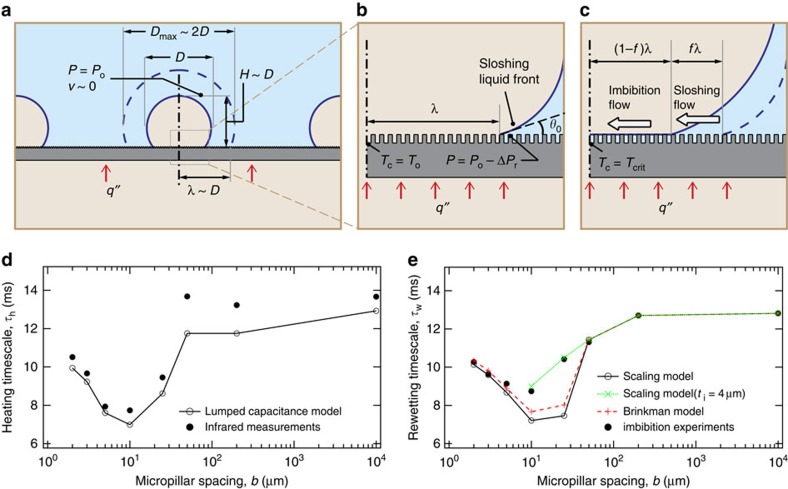
Figure 6. Characteristic timescales for the CHF scaling model.

(a) An idealized spherical vapour mass formed on the boiling surface at CHF. Corresponding to an average vapour mass diameter D, a dry spot of maximum radius λ∼D can form on the boiling surface. (b) At maximum dry spot radius, the initial substrate temperature at the centre of the dry spot is T_c=T_o. Assuming uniform bubble curvature for θ₀=0, the pressure at the sloshing liquid front is equal to the pressure of the comparatively static liquid near the top of the bubble P_o minus a surface wetting pressure reduction term (ΔP_r) for partially wetting surfaces (θ₀>0). (c) The dry spot is rewetted under the combined effect of gravity-induced inward sloshing motion of the surrounding liquid and imbibition of liquid into the surface micro-textures, where f is the fractional length of the dry spot rewetted as a result of the sloshing motion. At CHF, the substrate temperature T_c exceeds the critical dry spot temperature T_crit before the rewetting liquid front reaches the centre of the dry spot. The heating timescale τ_h corresponds to the increase in T_c from T_o to T_crit, whereas the rewetting timescale τ_w denotes the time for complete rewetting of the dry spot. (d) Plot of the dry spot heating timescale (τ_h) versus micropillar spacing b for the micro-textured surfaces at 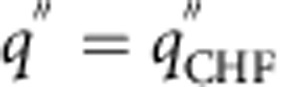. The calculated timescale is based on a thermal lumped capacitance model, whereas the experimental timescale is based on the maximum substrate temperature ramp rate obtained from infrared measurements. The value of the critical dry spot superheat is assumed to be T_crit—T_o∼12 °C. (e) Plot of the dry spot rewetting timescale (τ_w) versus micropillar spacing b for the micro-textured surfaces. The calculated timescale is based on the imbibition scaling model, whereas the measured timescale is based on the imbibition experiments (Supplementary Note 5). The contact angle of water on flat silicon was measured to be θ∼30° (see Methods).