Fig. 3.

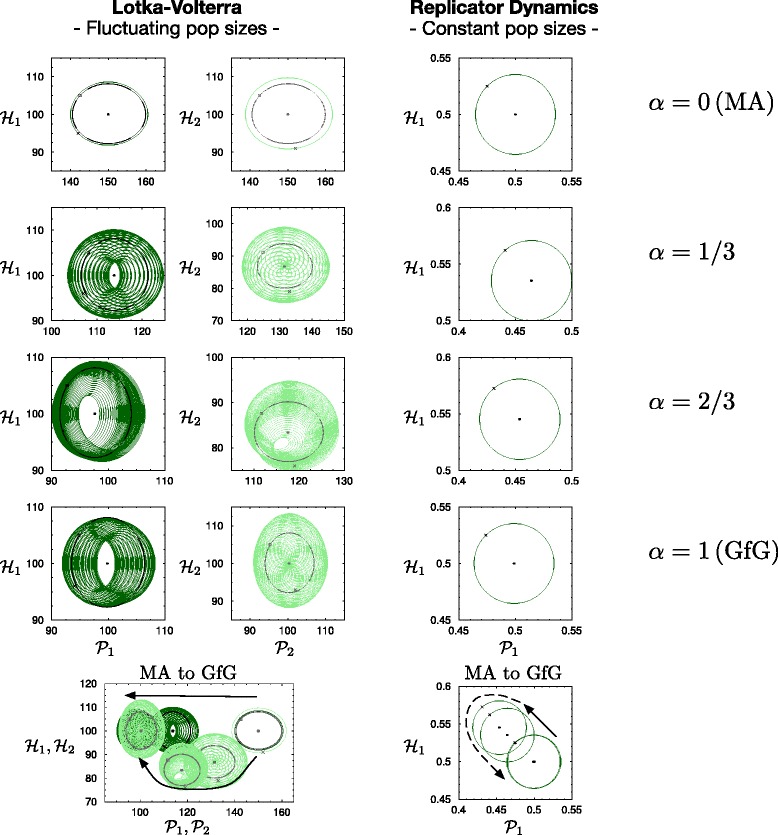
Trajectories close to the interior fixed points (black points) on the h ₁−p ₁ plane (dark green solid lines both for LV and RD equations) and the h ₂−p ₂ plane (light green dashed lines LV only). The black crosses mark the initial conditions. The black rectangle represent a special set of initial condition while the black solid/dashed lines show the corresponding trajectories. With Replicator Dynamics the h ₁−p ₁ trajectory is a closed circle. With Lotka-Volterra dynamics, the trajectories are closed circle when the initial conditions fulfill Eq. (25) (black lines). For the closed circles (black in LV and green in RD) the initial host population densities, h ₁ and h ₂ are 5 % above the corresponding fixed point, while the parasite population densities are 5 % beneath the fixed point. Except for α=0 (MA) the green trajectories with LV resemble tori instead of closed circles, an implication for two oscillation frequencies. To show the shift of the interior fixed point as α increases from 0 to 1, the trajectories are plotted all in the same coordinate system at the bottom