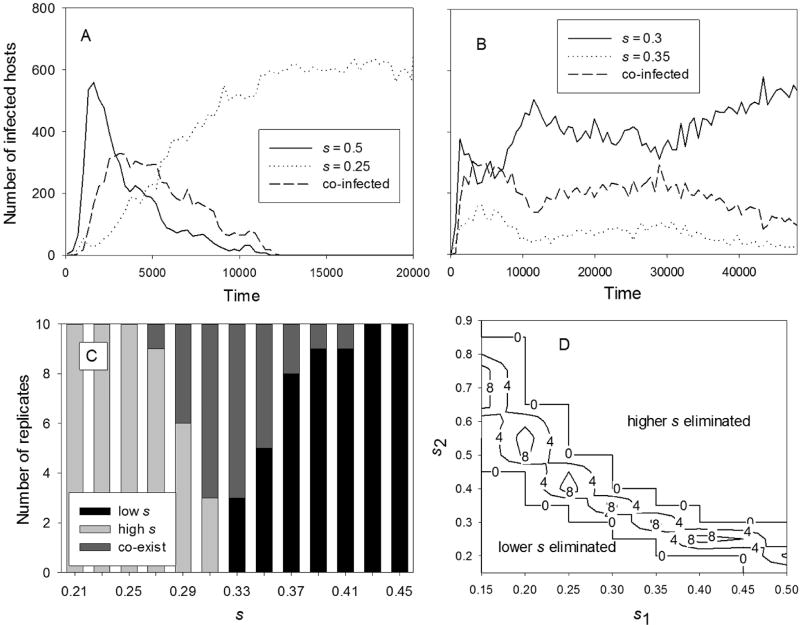
Fig. 3.

Competition and coexistence between clones competing within hosts. Within-host dynamics follow Eqs. (4). There are 2 clones, differing in s, introduced at the same time. Virus model parameters and mortality function are as in Fig. 1. Other parameters are θ = 10⁻¹⁵, and N = 1000, with 5 hosts each initially infected with one virion of each clone for (A) and (B), and 100 hosts for (C) and (D). There is co-infection and within-host competition. A. The s = 0.5 day⁻¹ clone initially infects more hosts, but is eventually displaced by the s = 0.25 day⁻¹ clone. B. With s = 0.3 day⁻¹ and s = 0.35 day⁻¹ clones, sometimes there is coexistence for a long time. C. Number of replicates (out of 10) for which the higher-s clone eliminated the lower-s clone (high s), the reverse (low s) and the two clones coexisted (coexist) at 50,000 time units. The abscissa is labeled with the average s of the two competing clones, which were separated by 0.02 units. D. Number of runs with coexistence (out of 10), for clones with s being a multiple of 0.05.