Fig. 2. Device tests.
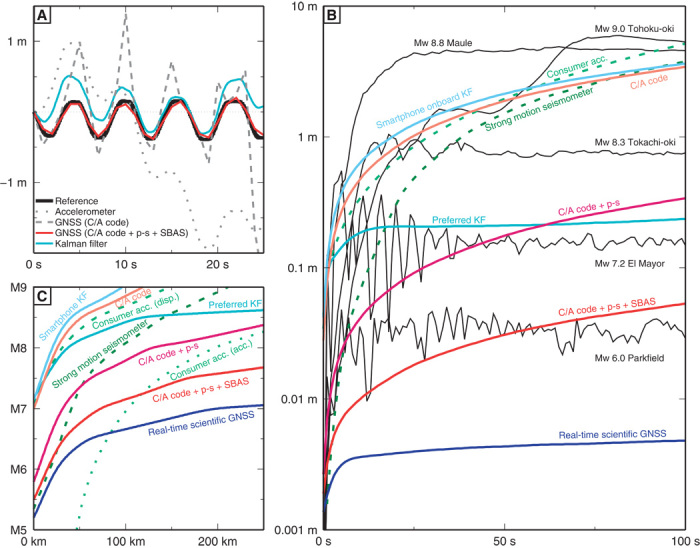
(A) Comparison of displacements obtained from consumer GNSS receivers with and without phase smoothing (p-s) and SBAS, by twice integrating smartphone acceleration and by Kalman filtering acceleration and GNSS data. Almost any smartphone or similar consumer device would generate the displacement and acceleration data shown with gray lines. Although these time series individually do a poor job of reproducing the true time history of motion (shown in black), they can be combined using a Kalman filter. This process produces one unified estimate of displacement (cyan) that is much less noisy than the original acceleration and displacement data used as inputs to the filter. However, the best GNSS hardware found in consumer devices (shown in red) is such high quality that there is no need to supplement the data with acceleration observations, and in fact, the displacement time series could be degraded by doing so. (B) Drift of position obtained from various devices (GNSS, double-integrated accelerometers, and Kalman filtering thereof) compared to observed earthquake displacements (7). Neither GNSS displacement observations nor acceleration data double integrated to displacement are stable over long periods. For GNSS data, this is because the inherent noise in the observations is not white noise. For acceleration data, this is because small tilts or steps in the observed acceleration cause large drifts when integrated. Thus, over time, the apparent position of sensors drifts, obscuring the true displacement of the instrument. The color curves show the apparent drift expected for each sensor and data type based on controlled tests. The black lines are observed displacement time series for earthquakes of different magnitudes. Thus, anywhere that a colored line is below a black line, the signal-to-noise ratio for that data type is greater than 1. In a crowdsourced setting, we expect to obtain data ranging in quality from a Kalman filter of C/A code data with acceleration data (cyan line created by combining data from light blue line with light green line), to C/A code data that have been phase-smoothed (“C/A code + p-s,” magenta line), to C/A code data that have been phase-smoothed and supplemented with SBAS (“C/A code + p-s + SBAS,” red line). Although all of these data types are significantly noisier than scientific-quality GNSS data (blue line), they are sensitive enough to record M6-7 earthquakes. (C) Using the drift curves shown in (B) and the peak ground displacement expected as function of magnitude and distance from the source (27), we can calculate the minimum magnitude earthquake observable with a signal-to-noise ratio of 10. Dotted line shows sensitivity of acceleration recorded on a smartphone. Dashed lines show sensitivity of displacement data obtained by twice integrating consumer and scientific acceleration data. At very close distances, the highest-quality consumer devices can observe earthquakes as small as M6 with a signal-to-noise ratio of at least 10.
