Fig. 2.

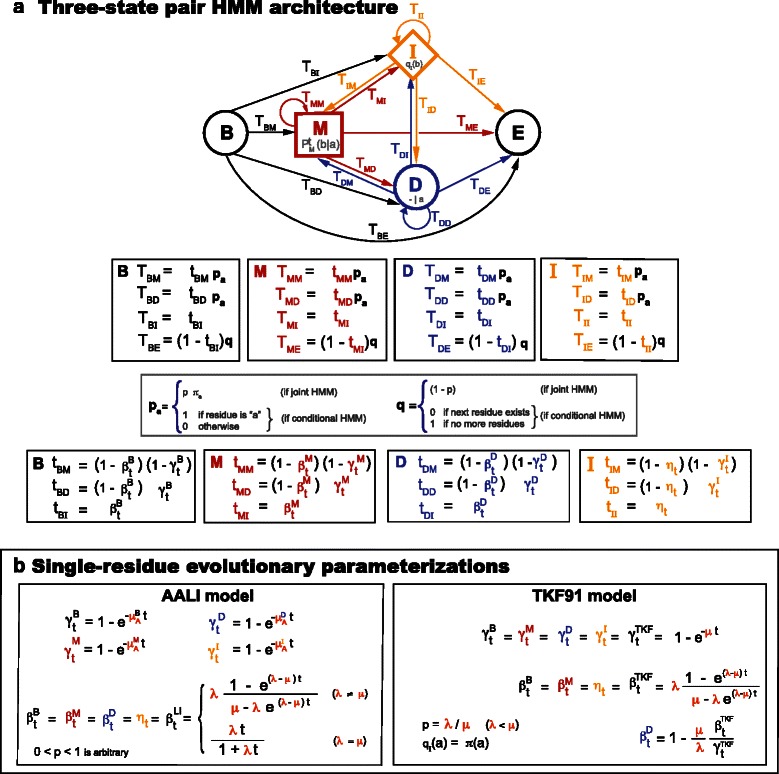
Standard affine three-state pair HMM with two explicit evolutionary parameterizations. a Standard probabilistic three-state M/D/I HMM (plus the customary B and E states) to describe homologous sequences under an affine gap cost. We simultaneously describe the joint and conditional versions of the HMM. For the joint version, we assume one of the sequences is generated with a length according to a geometric distribution of Bernoulli parameter p, and residues drawn from a probability distribution π. Joint residue match emissions occur with probability P _t(a,b)=π(a)P _t(b∣a), and conditional match emissions occur with probability P _t(b∣a). Inserted residues follow a probability distribution q _I which in principle could be different from π. The transition parameters t_XM,t_XD,t_XI are probabilities valued between zero and one. The HMM is normalized in its two versions (joint or conditional) as long as t_XM+t_XD+t_XI=1 for all states X={B,M,D,I}. b We write the transition probabilities of the HMM in terms of several elementary probability functions γ ^{B,M,D,I}(t) (the probability of deleting an ancestral residue), β ^{B,M,D,I}(t) (the probability of opening an insert), and η(t) (the probability of extending an insert). The HMM transition probabilities are automatically normalized for arbitrary elementary probability functions. Here we provide explicit time-dependent descriptions of the probabilistic elementary functions of the pair HMM according to the AALI and the TKF91 evolutionary models