Fig. 5.

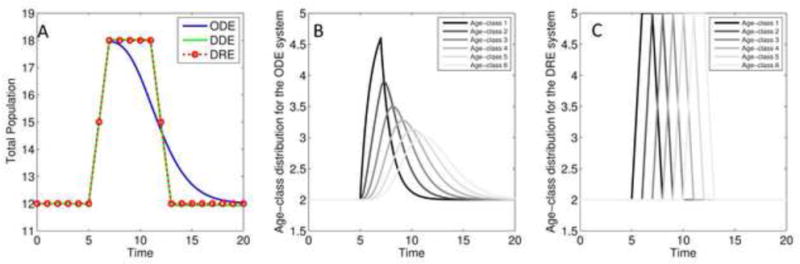
Comparison of the dynamical responses to a transient increase in cell influx by three different frameworks capable of modeling cells with fixed lifespan: ODE with age classes (ODE; ); DDE (DDE; ); and discrete recursive equation (DRE; ) with age classes. The models were set up with as much internal and external equivalence as possible (see Text). Panel A shows the simulation results for the total numbers of cells for the three frameworks. Panels B and C present the numbers of cells obtained for each age class of the ODE and DRE frameworks, respectively. These are shown with a color gradient from black (first age class) to light gray (sixth age class). The DDE and DRE frameworks behave equally well, as additional cells enter the pools between 5 < t ≤ 7. These cells are assumed to remain in the pool for τ = 6 time units. Thus, τ is the value of the delay in the DDE and also the number of age classes in the DRE and ODE frameworks. The ODE does not capture the sustained increase in cell number and instead immediately starts losing cells.