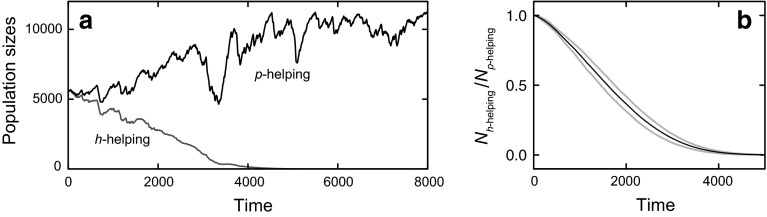
Fig. 4.

a Two populations with different forms of fitness are made to compete (implicitly through the Malthusian factor in Eq. 4, depending on combined population size), starting with equal number of individuals in each population. The graph shows how the populations change over time, as dependent on a time-varying environment E. The grey curve marked ‘h-helping’ implements a population that incorporates inclusive fitness (direct fitness as well as the indirect part of inclusive fitness). Helping depends on the size of h-subpopulations through the nonlinearity shown in Fig. 2b. The black curve marked ‘p-helping’ implements a population that incorporates direct fitness as well as the phenotypic part of extensive fitness (thus without h-helping). Helping depends on the size of p-subpopulations through the same nonlinearity as used for h-helping. Time is given in discrete time steps of the simulation; the typical organism’s lifetime equals 100 time steps. b Result of 100 simulations like in a for different realizations of E. The lines shows the ratio of the number of organisms in a population with h-helping (N _h-helping) and one with p-helping (N _p-helping). Black line: mean, grey lines: mean ± SD