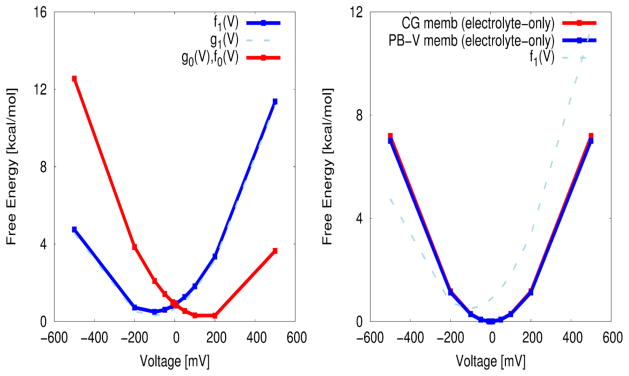
Figure 4.

The voltage dependent Marcus-Warshel parabolas, obtained using the CG model of voltage coupling (the KDW model). (A) The free energy of a unit charge movement as a function of external potentials using the CG energetics of voltage coupling, which is approximately quadratic, as predicted by linear response approximation applied to the voltage coupling of charge displacement. Notice that f_λ(V) {λ=0,1} interest at V=0 along the reaction coordinate of voltage coupling, which is a natural consequence of the equilibrium fluctuation relation of Eq. (15) that any system of two equilibrium states described by a reaction coordinate of energy gap (voltage coupling in the present study) should satisfy. g₁(V) (dashed light blue) is obtained by shifting f₁(V) (blue) by the conformational free energy of −ΔG_conf. (B) The free energy of charging the membrane between two electrolytes (i.e., the model system of (A) but in the absence of any charge within the membrane). The free energy parabola obtained by our KDW model shows an excellent agreement with the capacitor formula (Eq. (55)) obtained from the macroscopic continuum approach by solving a modified Poisson-Boltzmann equation (PB-V), yielding a capacitance of ~2.34e/V (in comparison with 2.33e/V from the capacitor formula of Eq. (55)).