Table 1.
Simulation results. Evolution in a finite ILGR population simulation with 106 groups was simulated for 1100 time periods. Initially, frequency of type A was p0 and groups were formed at random. Population regulation was given by the logistic 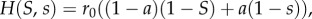
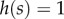 and δ = 0.01. There were three pay-off functions that gave the incremental effect of social behaviour on the expected number of offspring of types A and N in a group in which As had frequency x. Linear:
and δ = 0.01. There were three pay-off functions that gave the incremental effect of social behaviour on the expected number of offspring of types A and N in a group in which As had frequency x. Linear: 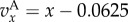 and
and 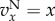 . Threshold: for
. Threshold: for 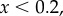
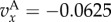 and
and 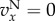 , and for
, and for 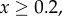
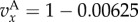 and
and 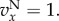 Iterated Public Goods Game (IPG): for
Iterated Public Goods Game (IPG): for 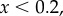
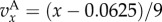 and
and 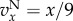 , and for
, and for 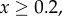
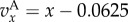 and
and 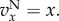 The observed value of Q is the average of values in the interval [100,1100] calculated using (33) in the electronic supplementary material. The first 100 time periods were ignored to allow the population to reach demographic quasi-equilibrium. The predicted value of Q is given by (3.4). The observed moments of the distribution of x were calculated using the empirical distribution of x at t = 1100 and the predicted ones were calculated using the beta(lp,lq) distribution for the values of p and
The observed value of Q is the average of values in the interval [100,1100] calculated using (33) in the electronic supplementary material. The first 100 time periods were ignored to allow the population to reach demographic quasi-equilibrium. The predicted value of Q is given by (3.4). The observed moments of the distribution of x were calculated using the empirical distribution of x at t = 1100 and the predicted ones were calculated using the beta(lp,lq) distribution for the values of p and 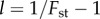 calculated from the empirical distribution of x at t = 1100. This is the beta distribution that has the first two moments identical to the observed ones, and for these reason those first two moments are omitted from the table. The observed value of p1100 was calculated from the distribution of x at time t = 1100, and the predicted value was calculated by iterating the recursion for pt given in (3.2) and (3.3), where the betas are calculated using
calculated from the empirical distribution of x at t = 1100. This is the beta distribution that has the first two moments identical to the observed ones, and for these reason those first two moments are omitted from the table. The observed value of p1100 was calculated from the distribution of x at time t = 1100, and the predicted value was calculated by iterating the recursion for pt given in (3.2) and (3.3), where the betas are calculated using 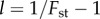 for period t, and Q is given by (3.4), beginning at time 100 with the value of p100 calculated from the simulation at time 100.
for period t, and Q is given by (3.4), beginning at time 100 with the value of p100 calculated from the simulation at time 100.
| population parameters |
moments of distribution of x |
||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| n0 | m | r0 | a | p0 | pay-off function | Q | 3rd | 4th | 5th | 6th | 7th | p1100 | |
| 80 | 0.05 | 1 | 0.5 | 0.1 | linear | pred. | −0.822 | 0.00313 | 0.00101 | 0.000388 | 0.000167 | 7.90 × 10−5 | 0.0697 |
| obs. | −0.816 | 0.00319 | 0.00104 | 0.000402 | 0.000174 | 8.30 × 10−5 | 0.0700 | ||||||
| thresh. | pred. | −0.822 | 0.0439 | 0.0211 | 0.0111 | 0.00621 | 0.00368 | 0.283 | |||||
| obs. | −0.821 | 0.0444 | 0.0214 | 0.0113 | 0.00641 | 0.00383 | 0.283 | ||||||
| IPG | pred. | −0.822 | 0.00327 | 0.00106 | 0.000404 | 0.000173 | 8.12 × 10−5 | 0.0714 | |||||
| obs. | −0.819 | 0.00338 | 0.00111 | 0.000427 | 0.000186 | 8.93 × 10−5 | 0.0730 | ||||||
| 320 | 0.05 | 1 | 0.5 | 0.1 | linear | pred. | −0.822 | 0.00535 | 7.31 × 10−5 | 1.17 × 10−5 | 2.13 × 10−6 | 4.40 × 10−7 | 0.0570 |
| obs. | −0.826 | 0.00553 | 7.66 × 10−5 | 1.24 × 10−5 | 2.31 × 10−6 | 4.77 × 10−7 | 0.0568 | ||||||
| thresh. | pred. | −0.822 | 0.0145 | 0.00416 | 0.00128 | 0.00417 | 0.000144 | 0.221 | |||||
| obs. | −0.822 | 0.0146 | 0.00428 | 0.00131 | 0.00428 | 0.000148 | 0.221 | ||||||
| IPG | pred. | −0.822 | 0.000885 | 0.000132 | 2.27 × 10−5 | 4.39 × 10−6 | 9.44 × 10−7 | 0.0710 | |||||
| obs. | −0.822 | 0.000911 | 0.000137 | 2.39 × 10−5 | 4.71 × 10−6 | 1.03 × 10−6 | 0.0717 | ||||||
| 20 | 0.05 | 1 | 0.5 | 0.1 | linear | pred. | −0.822 | 0.0345 | 0.0234 | 0.0172 | 0.0133 | 0.0107 | 0.133 |
| obs. | −0.798 | 0.0352 | 0.0241 | 0.0179 | 0.0140 | 0.0114 | 0.131 | ||||||
| thresh. | pred. | −0.822 | 0.170 | 0.131 | 0.107 | 0.0901 | 0.0771 | 0.366 | |||||
| obs. | −0.801 | 0.171 | 0.133 | 0.109 | 0.0923 | 0.0803 | 0.397 | ||||||
| IPG | pred. | −0.822 | 0.0180 | 0.0118 | 0.00846 | 0.00641 | 0.00510 | 0.0740 | |||||
| obs. | −0.797 | 0.0184 | 0.0121 | 0.00881 | 0.00681 | 0.00543 | 0.0787 | ||||||
| 40 | 0.05 | 1 | 1 | 0.1 | linear | pred. | −0.903 | 0.00784 | 0.00381 | 0.00211 | 0.00127 | 0.000822 | 0.0771 |
| obs. | −0.903 | 0.00808 | 0.00396 | 0.00221 | 0.00135 | 0.000885 | 0.0740 | ||||||
| thresh. | pred. | −0.903 | 0.0515 | 0.0300 | 0.0191 | 0.0130 | 0.00926 | 0.261 | |||||
| obs. | −0.902 | 0.0525 | 0.0308 | 0.0198 | 0.0136 | 0.00978 | 0.250 | ||||||
| 40 | 0.1 | 1 | 0.5 | 0.1 | linear | pred. | −0.681 | 0.00480 | 0.00170 | 0.000700 | 0.000320 | 0.000160 | 0.0842 |
| obs. | −0.664 | 0.00475 | 0.00165 | 0.000670 | 0.000305 | 0.000152 | 0.0861 | ||||||
| thresh. | pred. | −0.681 | 0.0908 | 0.0512 | 0.0308 | 0.0196 | 0.0129 | 0.380 | |||||
| obs. | −0.673 | 0.0908 | 0.0511 | 0.0308 | 0.0196 | 0.0131 | 0.384 | ||||||
| 80 | 0.05 | 0.5 | 0.5 | 0.1 | linear | pred. | −0.698 | 0.00393 | 0.00132 | 0.000513 | 0.000225 | 0.000108 | 0.0802 |
| obs. | −0.686 | 0.00408 | 0.00138 | 0.000546 | 0.000243 | 0.000118 | 0.0805 | ||||||
| thresh. | pred. | −0.698 | 0.0794 | 0.0431 | 0.0249 | 0.0152 | 0.00978 | 0.361 | |||||
| obs. | −0.700 | 0.0801 | 0.0435 | 0.0254 | 0.0156 | 0.0101 | 0.367 | ||||||
| 80 | 0.05 | 1 | 0.05 | 0.1 | linear | pred. | −0.316 | 0.00774 | 0.00279 | 0.00116 | 0.00531 | 0.000265 | 0.120 |
| obs. | −0.315 | 0.00800 | 0.00291 | 0.00122 | 0.00566 | 0.000285 | 0.120 | ||||||
| thresh. | pred. | −0.316 | 0.172 | 0.110 | 0.0740 | 0.0515 | 0.0414 | 0.504 | |||||
| obs. | −0.318 | 0.174 | 0.112 | 0.0755 | 0.0529 | 0.0383 | 0.507 | ||||||
| 80 | 0.05 | 1 | 0.5 | 0.9 | linear | pred. | −0.822 | 0.668 | 0.600 | 0.544 | 0.497 | 0.458 | 0.858 |
| obs. | −0.818 | 0.669 | 0.602 | 0.547 | 0.501 | 0.462 | 0.860 | ||||||
| thresh. | pred. | −0.822 | 0.856 | 0.821 | 0.790 | 0.762 | 0.738 | 0.942 | |||||
| obs. | −0.780 | 0.856 | 0.822 | 0.791 | 0.763 | 0.740 | 0.943 | ||||||
