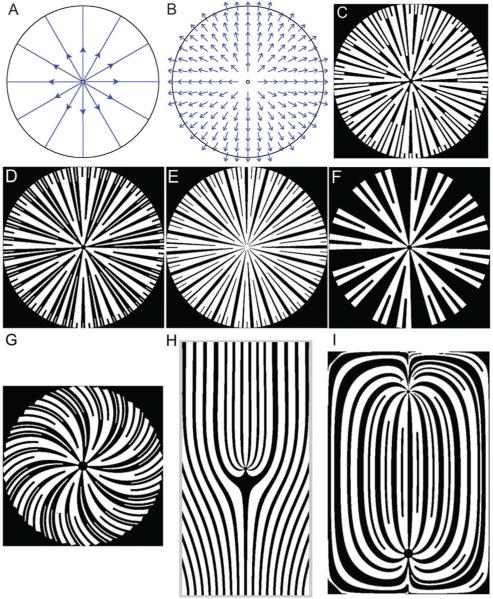
Fig. 2.

Varying the seed pattern and the rules of seed pattern propagation can result in distinct patterns that are based on the same vector field, with further diversity in patterns introduced by generating different underlying vector fields. (A) Direction of simulated fluid flow in a circular region resulting from the initial condition specification, i.e., a source at the center of the circle and a sink at the circumference. (B) The vector field defined by this flow can be used to generate several different patterns. Branching is allowed for both bands and gaps in (C) and (D), but only allowed for the bands in (E) and (F). In (E), the gaps are additionally constrained to not grow beyond a certain maximum width, while in (F) the gaps can grow in an unconstrained fashion. Additionally, varying the relative widths of the bands and gaps in the initial conditions, and the fractional widths of newly generated bands or gaps following a branching, are also conditions that give rise to a diverse range of patterns in (C–F). Changing the boundary conditions during the generation of the vector field, e.g., the direction of the flow to be non-normal to the perimeter of the patterned region, can result in a spiral pattern (G). By defining more than one source, e.g., a line source at the bottom edge of the rectangle and a point source in the center of the rectangle in (H), or by defining a source and a sink as two singularities within the rectangle (I), it is possible to further increase the pattern diversity. As shown in (I), one can define both the emergence and the fusion of adjoining bands (or gaps) depending on whether the local flow lines diverge or converge. See additional information in the ESI.†