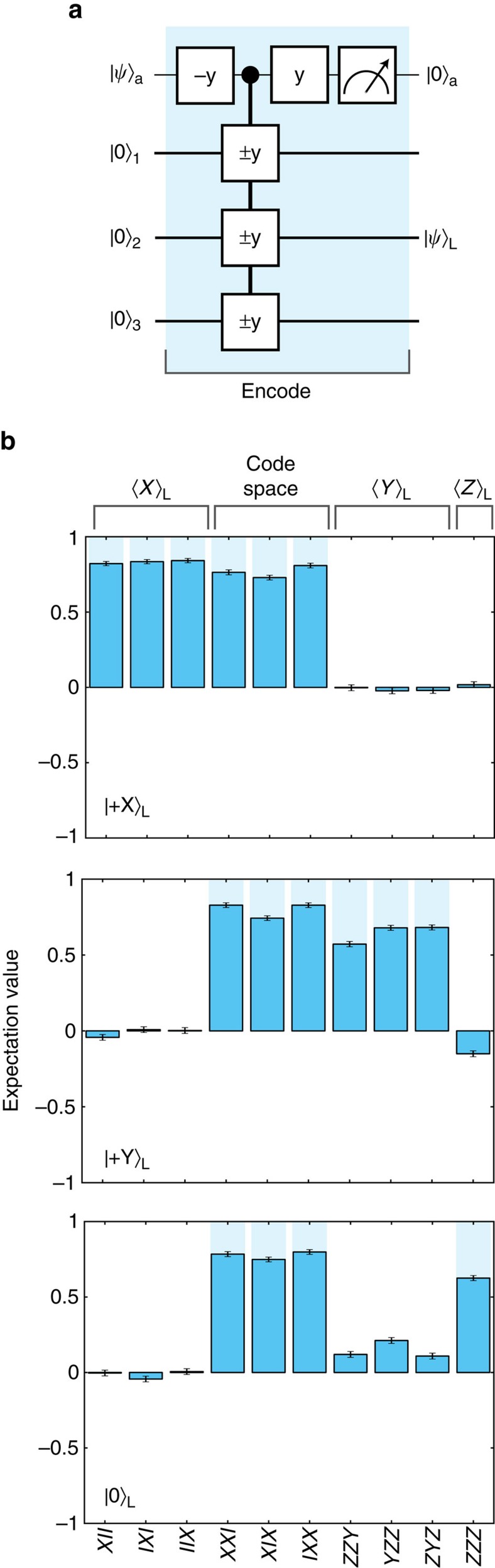
Figure 2. Encoding of the logical qubit.

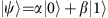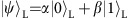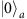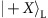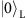(a) Encoding an arbitrary quantum state prepared on the ancilla into . Successful encoding is heralded by outcome . (b) Characterization of the logical states , and . Only the logical qubit operators and stabilizers are shown (see Supplementary Fig. 7 for complete tomography of all 6 logical basis states). The fidelities with the ideal three-qubit states are F=0.810(5), 0.759(5)and 0.739(5), respectively, demonstrating three-qubit entanglement¹⁰. The logical state fidelities are 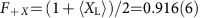, 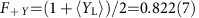and 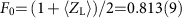. Ideally, all the encoded states are +1 eigenstates of the stabilizers X₁X₂I₃ and I₁X₂X₃. The fidelity to this code space, 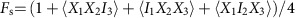, is 0.839(3) averaged over all states and gives the probability that the starting state is free of detectable errors. All error bars are one statistical s.d.