Skip to main content
PMC home page
Journal of Vision logoLink to Journal of Vision
. 2016 Jun 1;16(8):2. doi: 10.1167/16.8.2

The absolute disparity anomaly and the mechanism of relative disparities

Adrien Chopin 1,5,6,7, Dennis Levi 2,7, David Knill 3,8, Daphne Bavelier 4,6,8
PMCID: PMC4898198  PMID: 27248566

Abstract

There has been a long-standing debate about the mechanisms underlying the perception of stereoscopic depth and the computation of the relative disparities that it relies on. Relative disparities between visual objects could be computed in two ways: (a) using the difference in the object's absolute disparities (Hypothesis 1) or (b) using relative disparities based on the differences in the monocular separations between objects (Hypothesis 2). To differentiate between these hypotheses, we measured stereoscopic discrimination thresholds for lines with different absolute and relative disparities. Participants were asked to judge the depth of two lines presented at the same distance from the fixation plane (absolute disparity) or the depth between two lines presented at different distances (relative disparity). We used a single stimulus method involving a unique memory component for both conditions, and no extraneous references were available. We also measured vergence noise using Nonius lines. Stereo thresholds were substantially worse for absolute disparities than for relative disparities, and the difference could not be explained by vergence noise. We attribute this difference to an absence of conscious readout of absolute disparities, termed the absolute disparity anomaly. We further show that the pattern of correlations between vergence noise and absolute and relative disparity acuities can be explained jointly by the existence of the absolute disparity anomaly and by the assumption that relative disparity information is computed from absolute disparities (Hypothesis 1).

Keywords: stereoscopic vision, stereopsis, depth vision, psychophysics, stereoblindness, absolute disparity, relative disparity

Introduction

Most humans have a very keen ability to discern the distance of objects in the visual field based on a variety of visual depth cues, including occlusion, motion parallax, and perspective or shadows (Howard & Rogers, 2012b; Mamassian, Knill, & Kersten, 1998). However, the most precise cue is usually the stereoscopic cue that arises from the difference of viewpoints between the two eyes (Howard & Rogers, 2012a; Wheatstone, 1838). Interestingly, at least 4% of the population is totally blind to this stereoscopic cue (Patterson & Fox, 1984) and is functionally impaired because of this lack (Caziot & Backus, 2015; McKee & Taylor, 2010). Given the critical role of stereoscopic vision in depth perception, it is important to understand the mechanisms behind binocular depth vision.

Stereoscopic vision relies on binocular disparity. When one fixates at a given point in depth, an object located behind that point will produce an image horizontally shifted to the left of the left eye's fixation point and to the right of the right eye's fixation point. That is, each eye receives an opposite signed visual angle between the object and the fixation point (Figure 1a). The difference of these signed monocular angles is called the absolute disparity of an object. For a specific fixation point, the larger the absolute disparity, the larger the depth distance to the fixation point.

Figure 1.

Figure 1

Open in a new tab

Schematic illustration defining (a) absolute and (b) relative disparities. Viewed from above the scene: The two eyes belong to an observer looking at the fixation plane (dotted line) while an object is present at P1 (red dot). In each panel, the two frames depict the left-eye and right-eye images before projection through the pupil. (a) Inline graphic is the absolute disparity of P1. The visual angle between fixation and P1 is called φL in the left eye and φR in the right eye. By convention, the angles are signed positively when they fall on the left of the fixation point and signed negatively otherwise. (b) A second dot P2 is present (green square). The visual angle between P2 and P1 is called ηL in the left eye and ηR in the right eye. Inline graphic is the relative disparity between P1 and P2. Geometrically, it can be expressed as the difference between the absolute disparities of P1 (Inline graphic ) and P2 (Inline graphic ) or as the difference of the visual angles between P2 and P1 in each eye. We illustrate one putative mechanism to compute the absolute disparity of an object (computing the difference of signed monocular distances between the object and fixation). Note that this mechanism is formally equivalent to computing directly the dichoptic distance between the monocular images of the object. Whether one prefers one formulation or the other does not change the interpretation of our findings.

When a second object is located at a different depth, it produces an image with a relative disparity to the first object. To calculate the relative disparity of two objects, one can estimate each of the signed monocular angles between the objects' images and subtract them (Figure 1b). Thus, relative disparities are independent of the fixation point, while absolute disparities are defined relative to the fixation point.

Humans are especially good at discerning relative disparities (Badcock & Schor, 1985; Farell, Li, & McKee, 2004; Westheimer, 1979), raising the important issue of how the human visual system computes relative disparities. There has been a long-standing debate about the mechanisms underlying the perception of relative disparities (Westheimer, 1979).

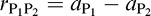Relative disparities between two visual objects (P1 and P2) could be recovered in two different ways, each of them being a two-step process. In the first way, relative disparities could be computed by first computing the absolute disparities of each object (Inline graphic and Inline graphic in Figure 1) and then subtracting these absolute disparities, following the formula Inline graphic (Figure 1). This implies that absolute disparities are the inputs to the relative disparity system (Schor, 2000). Therefore, we refer to this as the hypothesis of the feeding systems. It is identical to the direct disparity processing hypothesis (Westheimer & McKee, 1979), close to the differencing mechanism (Westheimer, 1979), and supported by the adapted energy model of Thomas, Cumming, and Parker (2002).

Each eye has an image of the two objects. In the second way, the visual system could first compute the image separations between objects formed in each eye individually (ηL and ηR in Figure 1) and then perform the subtraction between these two values (Schor, 2000). In that case, we have Inline graphic = ηL – ηR, and the relative disparity system does not rely on absolute disparities (Julesz, 1971, pp. 78–80; Stratton, 1900; Westheimer & McKee, 1979). We refer to this as the hypothesis of the independent systems, which is similar to Stigmar's (1970) measuring mechanism or to the prior uniocular hyperacuity processing hypothesis (Westheimer & McKee, 1979).

Surprisingly, while considerable efforts have been undertaken recently to understand the neural bases of absolute and relative disparities (Cottereau, McKee, Ales, & Norcia, 2012; Cottereau, McKee, & Norcia, 2012; Neri, Bridge, & Heeger, 2004; Parker, 2007; Roe, Parker, Born, & DeAngelis, 2007; Thomas et al., 2002), the debate over the relative disparity mechanism has never been completely settled (DeAngelis, 2000; Parker, 2007). Neurons tuned to absolute (Cumming & Parker, 1999) and relative (Thomas et al., 2002) disparities have been isolated. Although this could suggest independent systems, it is actually compatible with relative disparity neurons taking their input from absolute disparity neurons, as proposed by energy models (Thomas et al., 2002). Thus, more research is required to answer the question (Parker, 2007).

Comparing stereo and monocular position acuities

One way to tackle the question is to compare relative disparity detection thresholds and monocular position thresholds under similar conditions. If the hypothesis of independent systems is correct and the relative disparity between two objects is determined from the difference in the monocular separations between the objects, then stereo thresholds should be a function of monocular position detection thresholds. Geometrically, stereo thresholds should be a factor of two worse when the two objects are aligned in depth (McKee, Welch, Taylor, & Bowne, 1990; Walls, 1943). If the objects' retinal separation is just detectable in one eye, the liminal binocular disparity should be the sum of the two liminal separations in each eye; therefore, it would be two times larger. Indeed, early studies (Stigmar, 1970) found better vernier detection thresholds than stereo thresholds, but several later carefully-controlled studies (Berry, 1948; Patel, Bedell, Tsang, & Ukwade, 2009; Westheimer & McKee, 1979) contradicted the prediction. They discovered that the ratios between positional and stereo thresholds vary depending on the conditions, such as the separation between objects (Westheimer & McKee, 1979) or the pedestal (McKee, Levi, & Bowne, 1990). However, stereo and positional thresholds vary similarly with eccentricity (McKee, Welch, et al., 1990), which makes it difficult to reach a definitive conclusion.

Comparing absolute and relative disparity acuities

Another way to probe how relative disparity is computed is to directly compare absolute and relative disparity acuities. The literature reports better relative disparity thresholds than absolute ones (Westheimer, 1979), suggesting independence between the absolute and relative disparity systems. However, both theoretical and methodological considerations potentially weaken such a conclusion.

First, it may be wrong to assume that the absolute disparity is directly accessible by the observer for judging depth. Electrophysiological studies of V1 neurons in the monkey cortex were found to be highly selective for absolute disparities but not for relative disparities (Cumming & Parker, 1999). Importantly, V1 neurons can also be driven by anticorrelated dots (Cumming & Parker, 1997)—that is, in the absence of depth perception. Thus, physiological data in macaques suggest that absolute disparity is coded in V1 neurons but is not accessible for judging depth (Prince, Pointon, Cumming, & Parker, 2000). Specifically, the authors found that some V1 neurons outperformed the monkey's psychophysical behavior for random-dot depth discriminations when the random-dot background was removed (absolute disparities) but not when the background was present (relative disparities).

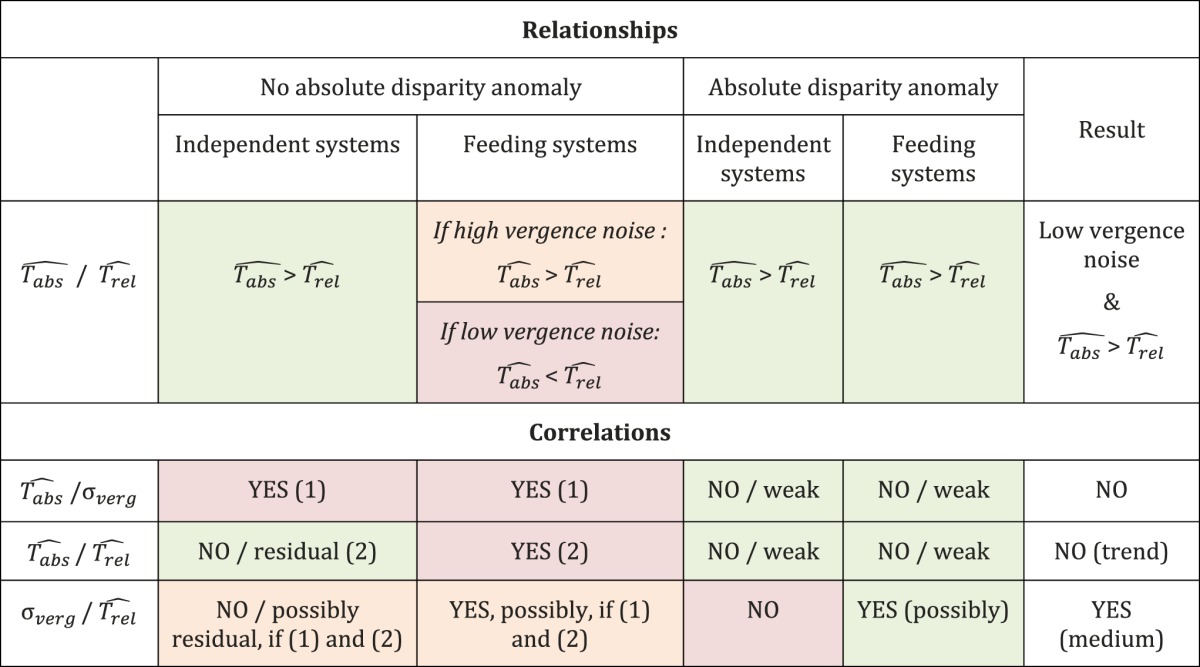
Consistent with this view, absolute disparity changes can drive large vergence changes while producing only a limited percept of motion in depth as opposed to relative disparity changes (Regan, Erkelens, & Collewijn, 1986), and vertical disparities can drive significant vergence movements with no participant's control or awareness (Stevenson, Lott, & Yang, 1997). Based on these studies, we consider the possibility that better relative disparity acuity does not necessarily mean independent systems; it could just be that the absolute disparity signals are not directly accessible for judging depth. We call this the absolute disparity anomaly (i.e., there is no conscious readout of absolute disparities). Interestingly, the anomaly could explain why the absolute disparity thresholds are so high compared with the relative disparity thresholds, independent of the way relative disparities are calculated (Table 1).

Table 1.

Predictions for the two hypotheses concerning the mechanism of relative disparities, depending on the presence or absence of the absolute disparity anomaly and on the size of the vergence noise. T̂abs is the measured absolute disparity threshold, T̂rel is the measured relative disparity threshold, and σverg is the measured vergence noise using the Nonius line technique. The results of our experiments are indicated in the last column. The satisfied expectations are highlighted in green, the unsatisfied ones are shown in red, and the conditionally unsatisfied ones are shown in orange (i.e., the result would be like expected if another expectation was satisfied but is not).

graphic file with name i1534-7362-16-8-2-t01.jpg

Open in a new tab

Second, measuring relative disparity acuity typically involves discrimination of simultaneously presented targets at different depths, whereas absolute disparity acuity usually is measured via a two-interval forced-choice discrimination task between successively presented targets, a necessary step to prevent participants from simply using relative disparity information. The relative impact of simultaneous versus sequential presentation remains largely unexplored. Nevertheless, the simultaneous (relative disparity) and successive (absolute disparity) tasks have different memory loads inherent to the task. Indeed, for the successive task, the depth perceived in the first interval needs to be memorized before being compared with the second interval. Our study addressed this memory load issue directly.

We now consider the predictions of the feeding system hypothesis (assuming that the task memory load is equal for the two tasks) in the absence of the absolute disparity anomaly. Interestingly, relative disparity acuities should be worse than absolute disparity acuities (see Supplementary Appendix A) given that relative disparities are then extracted by subtracting absolute disparities. However, the prediction holds only when the vergence noise is low enough. We have calculated in Supplementary Appendix A that the opposite is expected when the vergence noise exceeds the value

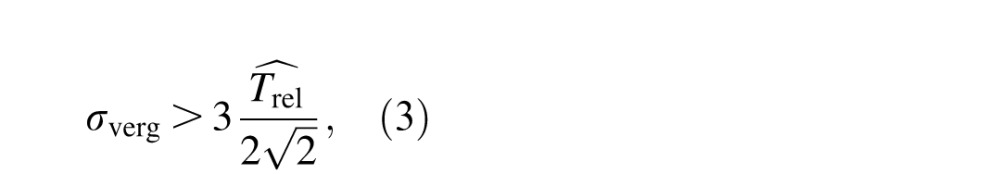graphic file with name i1534-7362-16-8-2-e01.jpg

where σverg is the measured vergence noise using the Nonius line technique (see Method) and Inline graphic is the threshold measured in a relative disparity task.

Studying the correlation pattern

Another way to uncover the mechanism of computation for relative disparities is to consider the correlations between absolute and relative disparity thresholds on one side and their respective correlation with vergence noise. Methods for studying individual differences efficiently have been clearly formulated specifically to investigate stereopsis (Wilmer, 2008). However, this approach has not been fully explored in the past because of the limited size of the samples used in previous studies. Recently, correlations on large groups have been successfully used to study the independence of binocular mechanisms (Nefs, O'Hare, & Harris, 2010). Below we review the predictions of each hypothesis on the pattern of correlations between absolute and relative disparity thresholds and vergence noise.

Predictions in the absence of the absolute disparity anomaly

If the hypothesis of feeding systems is true, absolute and relative disparity thresholds should be highly correlated because relative disparities are calculated from absolute disparities. Similarly, it is known that vergence eye movements rely on absolute disparities (Cumming & Parker, 1997; Erkelens & Collewijn, 1985a, 1985b; Masson, Busettini, & Miles, 1997) and that absolute disparities are corrupted by vergence noise. Therefore, vergence thresholds and absolute disparity thresholds should be correlated too. Finally, relative disparity and vergence acuities could also be correlated (but not necessarily) to a lesser extent because both depend on absolute disparities (Table 1). We emphasize that the absence of this latter correlation is not a falsification of expectations because A can be correlated with B and C while B and C are not correlated with each other.

Assuming that the hypothesis of independent systems is true, one might expect no correlation between absolute and relative disparity thresholds because they would be computed from distinct systems that do not share the same processes (see Discussion). A strong correlation between vergence noise and absolute disparity thresholds is still expected (Table 1) as explained above. If there is no correlation between absolute and relative disparity acuities, then no correlation between relative disparity and vergence thresholds is expected because such a correlation only reflects the double relation between absolute disparity and relative disparity acuities and between absolute disparity and vergence acuities (see Discussion).

Predictions in the presence of the absolute disparity anomaly

The absolute disparity anomaly modifies the predictions discussed above. First, in all cases, absolute disparity thresholds will be high compared with relative disparity thresholds. Second, the expected pattern of correlations will be modified. Absolute disparity remains an input to vergence and, in the case of the hypothesis of the feeding systems, to the relative disparity. However, the anomaly precludes the measurement of the true absolute disparity thresholds because they are not available for judging depth. As a result, we expect all direct correlations with the absolute disparity sensitivities to be weak. Only an indirect correlation between relative disparity thresholds and vergence noise is expected in the case of the hypothesis of the feeding systems (but not necessarily) because both systems depend on absolute disparities. In contrast, if the independent system hypothesis is true, then relative disparities do not depend on the absolute disparities, and, therefore, no correlation is expected (we argue about that expectation in the Discussion). Finally, all expected correlations are predicted to be positive. We summarize all these predictions in Table 1.

Our study

In the experiment described here, we control for task memory load and vergence noise and we study the different correlations discussed above. The memory load issue is addressed by using the method of single stimuli (Farell et al., 2004; Morgan, 1992; Morgan, Watamaniuk, & McKee, 2000). In this method, the participant is always presented with a unique stimulus. The task requires comparison of the stimulus with a memorized reference, which is the average of all previously presented stimuli. Therefore, the memory load inherent to the task is identical when measuring the absolute or the relative disparity acuities.

In order to minimize vergence eye movements, we used brief presentations (200 ms). We separately measured vergence noise with the Nonius line technique. This measure allows us to derive the absolute disparity thresholds expected under the feeding system hypothesis (and no absolute disparity anomaly).

Finally, in the literature, mostly small samples of experts (i.e., highly experienced psychophysical observers) have been tested; these are usually researchers or lab students who have been trained extensively on the tasks. It is unclear whether their performance reflects that of naive observers and whether the specific tasks they have been trained on have biased their acuity to favor one type of disparity over another. Therefore, we tested a large sample of naive observers (not working in vision labs) to obtain a measure unbiased by training.

To anticipate, our results show that absolute disparities cannot be accessed directly in line with the absolute disparity anomaly and that vergence thresholds are significantly correlated with relative disparity thresholds only, suggesting that relative disparity is calculated from the difference of absolute disparities (feeding system hypothesis).

Method

Overview

An experimental session started with clinical acuity and clinical stereoacuity tests, after which a stereoscope was adjusted individually for each participant using Nonius lines to ensure proper alignment. Observers then underwent separate practice blocks (15 trials each) for each of the two stereo tasks with unlimited presentation time (with a fixation point), followed by additional practice blocks with short presentation times (200 ms). Both the practice and experimental blocks provided auditory feedback. The actual experiment consisted of three blocks of 175 trials/block (each one preceded by 15 more practice trials without fixation point)—one for the relative disparity task and two for the absolute disparity task. The order of blocks was counterbalanced across participants. Following the three blocks of stereo tests, observers performed the vergence task.

Observers

Twenty-one naive observers (four females, 17 males; age range = 19–35 years, average age = 24.1 years) were recruited with an ad posted at the University of Geneva. None was working in a vision lab.

All participants had normal or corrected-to-normal vision when tested with the Sloan chart at 3 m (clinical acuity test) and were naive to the goals of the study. All observers had a crossed stereoacuity better than or equal to 70 arcsec on the circles part of the Randot and Butterfly clinical stereo tests and passed the random-dot stereogram part of each test. Consistent with Heron and Lages (2012), no participant was excluded at the clinical stereo test stage; however, normal vision was a criterion in the recruitment process, which means that self-selection of participants with good vision likely occurred. As a result, our sample is representative only of the population with normal vision acuity. All subjects signed an informed consent and received monetary compensation.

Materials

Stimuli were presented on a ViewPixx screen (VPixx Technologies, Saint-Bruno, Québec, Canada) in a dark room and were viewed through a custom-designed four-mirror stereoscope at a distance of 2.1 m (through the mirrors). The minimal luminance of the screen (black) was slightly above zero (<1 cd/m2) so that the edge of the screen was just barely visible. Therefore, we masked the screen edges with an additional black panel in a chevron shape so that it was in binocular rivalry when viewed through the stereoscope (Figure 2b). Observers had their head stabilized with a head-and-chin rest and responded via the keyboard.

Figure 2.

Figure 2

Open in a new tab

(a) Schematic depiction of the two different tasks: the absolute disparity task to measure absolute disparity thresholds (left) and the relative disparity task to measure relative disparity thresholds (right). (b) Schematic timeline depicting a trial of the stereo tasks. For the absolute disparity task, the two lines shown after the mask had the same depth, while they had a different depth for the relative disparity task. The gray fields depict a black panel that was used to hide the screen edge and to create a different edge shape with no binocular part.

Stereo task stimuli

Thresholds for absolute and relative disparities were measured using two stereo tasks with nearly identical stimuli. In this article, we consider only horizontal disparities. A trial started with the fusion screen (Figure 2b) consisting of a fixation point (90-arcsec diameter), white Nonius lines, a large frame, and small fusion frames (seen by both eyes). All white stimuli in the experiment had a luminance of 20 cd/m2, and all lines were 1 arcmin wide except when otherwise stated. The Nonius lines, designed to ensure appropriate vergence at the fixation point, consisted of two dichoptic vertical lines aligned with the fixation point, one above (in one eye) and the other below (in the other eye). Each line was 20 arcmin long and 4 arcmin above or below fixation. Two smaller binocular horizontal lines (5 arcmin long) flanked the fixation point 4 arcmin away from it. When seen together, the two binocular lines with the two Nonius lines completed a cross. The lines were white and were presented on a black background (<1 cd/m2). The cross was surrounded by a binocular white square frame (1° on each side), and this was surrounded by a series of binocular small gray square frames (8.8 arcmin on each side and 3 cd/m2). These squares were randomly distributed in two rows outside of a virtual 2°-wide square centered on fixation. The role of these surrounding items was to help maintain fusion and to lock vergence.

Procedure

Observers were instructed to fixate the fixation point and maintain precise vergence. The perceived horizontal alignment of the Nonius lines served as feedback for vergence. When properly aligned, and thus appearing as a fixation cross in a white box, the observer's key press initiated the disappearance of all visible items, simultaneously with the presentation of a mask (uniform uncorrelated white noise, with dots between 0 and 10 cd/m2), for 10 ms. It was followed by the brief appearance (200 ms) of the stereoacuity stimulus: two white vertical lines 4 arcmin above and below the extinguished fixation point. The brief presentation time precluded vergence eye movements. The disparity of the lines depended on the task (see below). The lines were 20 arcmin long and 26 arcsec wide. Subpixel precision was achieved using a simple variant of the ribbon and quadrant techniques (Georgeson, Freeman, & Scott-Samuel, 1996; Westheimer & McKee, 1977, 1979): Each stereo line consisted of three adjacent-pixel vertical lines whose light distribution determined the subpixel position of the stereo line. The horizontal position of both lines was jittered by adding a random shift from a uniform distribution between −15 and +15 arcmin in order to avoid monocular cues. Following the two lines, a totally black screen was shown until the observer responded.

Absolute disparity task

The general task design described above was adapted to measure absolute disparity thresholds. The fusion frame was yellow during this task to distinguish it from the relative disparity task described below. For this absolute disparity measure, observers were presented with the two vertical lines at the same depth and were instructed to respond according to the distance in depth between the lines and the (extinguished) fixation point—specifically, whether the depth between the lines and the (extinguished) fixation point on the trial was larger or smaller than the average depth of all trials in the block so far (Figure 2a). This method of single stimuli with an implicit reference (Farell et al., 2004; Morgan, 1992; Morgan et al., 2000) allows for minimizing the differences in memory demand that are inherent to the task of measuring absolute and relative disparity acuities.

Five depth values, all crossed disparities, were chosen symmetrically around the implicit reference that was a 5-arcmin depth interval. Observers began each block with 15 additional practice trials (allowing them to estimate the reference), followed by a subblock of 50 trials with depth values chosen to span a large range. The lines were presented at 0.5, 2.75, 5, 7.25, and 9.5 arcmin. An initial threshold estimate based on this subblock was used to determine the stimulus depths for the second subblock of 125 trials, in which the five depth values were the reference depth ±2 SD, if that resulted in a range of depths smaller than the initial one. In those cases when it wasn't smaller, the initial depth values were used.

The relative disparity task described below features two lines, one located at an average 5-arcmin depth and the other at an average 10-arcmin depth. For comparison with that task, one of the two blocks of the absolute disparity task had a reference of 10-arcmin depth. For this block, lines were presented at 5.5, 7.75, 10, 12.25, and 14.5 arcmin.

Relative disparity task

The task described above was adapted to measure relative disparity thresholds. The fusion frame was blue during this task. Following the Nonius lines and the mask, observers were presented with two vertical lines identical to those in the absolute disparity task, but each line had a different depth. They were instructed to respond according to the distance in depth between the lines and whether it was larger or smaller than the implicit reference, defined as the average of all the depth distances between the lines for all trials in the block so far (Figure 2a). All the line pairs had crossed disparities, and their relative depth distance was chosen symmetrically around the depth distance reference of 5 arcmin. Observers began each block with 15 practice trials (to estimate the reference) followed by a subblock of 50 trials, with distances between lines first chosen to span a large range (0.5, 2.75, 5, 7.25, and 9.5 arcmin). A threshold estimate based on this subblock was used to determine the range of depth values for the second subblock of 125 trials in which the five depth values were the reference depth ±2 SD, if that resulted in a range of depths smaller than the initial one. The two lines were always presented symmetrically around 7.5 arcmin, which means that the depth average of the lines closer to the fixation point was 5 arcmin and the depth average of those farther from fixation was 10 arcmin.

Vergence task

In order to measure their vergence noise, observers were first presented with the fusion screen and instructed to align the Nonius lines. After a key press, the Nonius lines disappeared with all fusion lock items and the mask appeared (10 ms). It was quickly replaced by two new Nonius lines—the flashed lines—for 200 ms. A horizontal gap existed between the flashed lines, so they were misaligned. Observers reported whether the upper flashed line was presented to the left or the right of the lower flashed line. We varied the gap between the flashed lines with a staircase procedure designed to converge at a performance of approximately 75% correct. We used four randomly interleaved staircases with initial values of 1.2, 4.7, 8.2, and 11.7 arcmin. The staircases followed an accelerated stochastic approximation (Kesten, 1958), with an initial step of 4.5 arcmin and a stopping criterion of 1 arcmin. In addition, the flashed lines were displayed with a random horizontal jitter (before applying the gap), drawn from a uniform distribution between −15 and +15 arcmin.

Perceptual learning experiment

To test whether participants improved with practice, we retested six observers five times for the 5-arcmin absolute disparity condition.

Data analysis

For the stereo tasks, data were expressed as a probability of responding behind the reference (a smaller distance to fixation) as a function of the distance to the fixation plane (for the absolute disparity task; Figure 3a) or smaller than reference as a function of the distance between lines (for the relative disparity task; Figure 3b). The data were fitted with a cumulative normal distribution function from which we extracted the 75% correct stereo threshold (proportional to the standard deviation of the distribution).

Figure 3.

Figure 3

Open in a new tab

Example of psychometric functions for (a) the absolute disparity task and (b) the relative disparity task for a typical participant. (a) Probability of responding that the line is behind the reference (indicated by a purple dashed line) for the absolute disparity condition. The two subblocks described in Method are pooled together. (b) Probability of responding that the depth between the lines is larger than the reference (indicated by a black dashed line) for the relative disparity condition. The psychometric functions are fit with a normal cumulative distribution function.

For the vergence task, the data were expressed as a probability of responding left as a function of the distance between the flashed Nonius lines. The data were fitted with a cumulative normal distribution function from which we extracted the 75% correct vergence threshold (proportional to the vergence noise). We estimated vergence thresholds in two conditions (when the upper Nonius was presented in the left eye and when it was presented in the right eye) and averaged the two measures. This procedure gave lower noise estimates than simply averaging the two curves. The vergence bias was calculated as the absolute value of the difference between the points of subjective equality (estimate of the mean parameter of the function) of the two curves. When a participant fixates, on average, on the fixation plane, her bias is null. If fixation on all trials was exactly on the fixation plane, her vergence noise would be null too.

Results

We measured observers' thresholds for localizing lines in depth in two conditions: when only absolute disparities are available (absolute disparity condition) and when relative disparities are added (relative disparity condition). Typical psychometric functions can be seen in Figure 3.

For most analyses, we used raw data and nonparametric tests based on the median values. For some analyses, we also added parametric statistics on thresholds, with thresholds above 3000 arcsec considered to be 3000 arcsec. In addition, for the following analyses, we excluded three participants who did not reach a relative disparity threshold better than 3000 arcsec. We refer to these participants as stereo impaired (despite their good clinical stereopsis). However, we included them when analyzing stereo impairment proportions and correlations.

First, we used nonparametric statistics to test the difference in raw acuity between the two absolute disparity blocks (references at 5- and 10-arcmin depth); they did not differ significantly, Wilcoxon signed rank test T(18) = 109, p = 0.12. We confirmed this using parametric statistics on the data, with acuities above 3000 arcsec “ceilinged” at 3000 arcsec, t test T(17) = 0.99, p = 0.33. Parametric statistics can be used because no distribution could be shown to be different from normal distributions using Kolmogorov–Smirnov tests (p > 0.05). As a result of this analysis, we merged together the data from the two absolute disparity blocks for the rest of the analyses.

Our main result is that raw thresholds in the absolute disparity task were significantly higher (worse) than those in the relative disparity task by almost a log unit (Figure 4), Wilcoxon signed rank test T(18) = 171, p < 10−3. We also confirmed this using parametric statistics on the data when limiting the values above 3000 arcsec to 3000 arcsec, t test T(17) = 8.77, p < 10−6, Cohen's d = 2.98.

Figure 4.

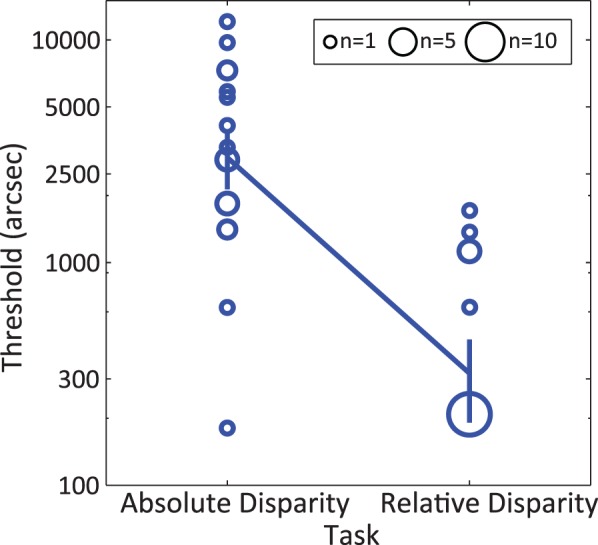
Figure 4

Open in a new tab

Stereo thresholds for the relative disparity task and the absolute disparity task. Central values (solid line) show the medians for each task. For visualization purposes, the surface of each data circle is proportional to the number of observers with thresholds within a 4-arcmin window centered on the circle. Error bars are standard errors.

Vergence noise was, on average, 225 ± 61 arcsec—too small to explain the large difference between absolute and relative disparity acuities if the feeding system hypothesis was true in the absence of an absolute disparity anomaly. According to Equation 3, the vergence noise should be larger than the relative disparity threshold divided by two thirds of the square root of 2, which is equal to 564 arcsec on average; we found the opposite.

To illustrate this, we calculate the absolute disparity thresholds that are expected from the feeding system hypothesis, and in the absence of the absolute disparity anomaly, by combining relative disparity thresholds and vergence noise for each observer following Equation 4:

graphic file with name i1534-7362-16-8-2-e02.jpg

from Equations 1 and 2 (see Supplementary material), where Inline graphic is the expected absolute disparity threshold, σrel is the standard deviation of the psychometric function for the relative disparity task, and 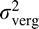Inline graphic is the variance of the vergence psychometric function.

Measured absolute disparity thresholds were always higher than the predicted thresholds based on the feeding system hypothesis in the absence of an absolute disparity anomaly using nonparametric tests on the raw data (Figure 5), Wilcoxon signed rank test T(18) = 188, p < 10−5, and parametric tests on the data under a 3000-arcsec ceiling, T(17) = 4.9, p < 10−3.

Figure 5.

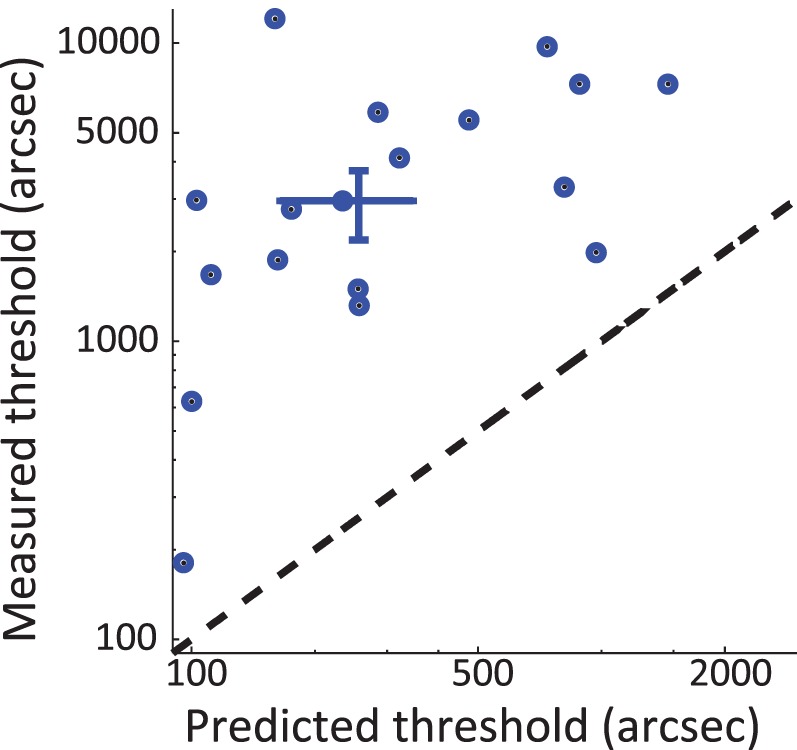
Figure 5

Open in a new tab

Predicted threshold for absolute disparities, as derived from the measured vergence noise and relative disparity thresholds, under the feeding system hypothesis and assuming there is no absolute disparity anomaly. Each dot shows a participant threshold (raw data), and the central cross shows medians and standard errors. All dots fall above the unity line (black dotted line), showing an actual threshold worse than predicted.

For the correlation analysis, we always use sensitivities (inverse of raw data thresholds, even when thresholds are greater than 3000 arcsec). We were concerned that a few outliers could have an exaggerated influence and inflate the correlations. Therefore, we first investigated the relationships between sensitivities using least-squares linear regression analysis. This analysis can control for outlier impact in two ways: (a) robust fitting with bisquare weighting and (b) identification and removal of outliers with large Cook's distances. The robust linear regression of relative disparity sensitivities using vergence sensitivities as a predictor generated one outlier using Cook's distance, which was removed. Intercept α1 was not significantly different from zero (p = 0.90), but slope β1 was (one outlier), β1 = 0.36, t test T(18) = 2.68, p < 0.05, R2 = 0.32 (Figure 6). The same analysis issued neither a significant regression of relative disparity sensitivities over absolute disparity sensitivities (one outlier), β2 = 0.018, t test T(18) = 1.31, p = 0.20, nor a significant regression of vergence sensitivities over absolute disparity sensitivities (one outlier), β3 = 1.61, t test T(18) = 0.45, p = 0.66.

Figure 6.

Figure 6

Open in a new tab

Relative disparity sensitivities as a function of vergence sensitivities (blue dots) for each observer, regression line (solid red line), and 95% confidence interval bounds (dashed red line).

Using Spearman correlations (with the same outliers excluded), vergence sensitivities were significantly correlated (Figure 7) with relative disparity sensitivities (r = 0.53, p < 0.01; one tailed) but not with absolute disparity sensitivities (r = 0.08, p = 0.35; one tailed). The correlation between absolute disparity and relative disparity sensitivities was not significant (r = 0.35, p = 0.06; one tailed). This pattern of correlations is compatible only with the hypothesis of the feeding systems in combination with the absolute disparity anomaly (Table 1, column 5).

Figure 7.

Figure 7

Open in a new tab

Correlations between the measured sensitivities for absolute disparities, relative disparities, and vergence (left), and suggested descriptive relations between the corresponding cognitive information (right). Absolute disparities feed both the relative disparity system and the vergence system, but the absolute disparities cannot be accessed directly for judging depth (the absolute disparity anomaly), explaining the correlation pattern. Significant correlations are indicated by asterisks (*p < 0.05; n.s. = p > 0.05).

While a larger number of participants might have resulted in a significant correlation between absolute and relative disparity sensitivities, our conclusions rest on the fact that only the hypothesis of the feeding systems in combination with the absolute disparity anomaly predicts a positive correlation between vergence and relative disparity sensitivities.

Average absolute disparity thresholds were coarse (2138 arcsec) because many participants had performances above 3000 arcsec. This finding is in line with the presence of the absolute disparity anomaly. To further characterize the finding, we calculated the proportions of stereo-impaired participants and participants with no fine stereopsis.

Stereo impairment

We define a stereo-impaired observer as an observer with a threshold equal to or larger (worse) than 3000 arcsec (Figure 8). The proportion of stereo impairment was significantly different between disparity tasks (χ2 = 5.90, p < 0.05) and was higher in the absolute disparity task (45.2%) compared with the relative disparity task (14.2%). For that comparison and the next, proportions were calculated separately for the blocks of the absolute disparity task with the 5- and 10-arcmin references, before being averaged, to avoid decreasing the proportions artificially through probability summation.

Figure 8.

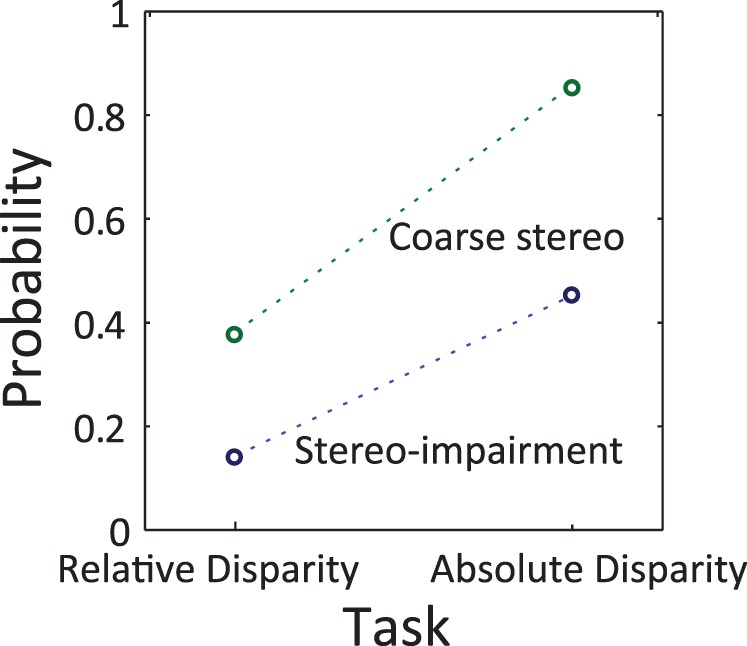
Figure 8

Open in a new tab

Probabilities of stereo impairment (threshold ≥ 3000 arcsec; blue circles) and coarse stereopsis only (threshold > 900 arcsec; green circles) for each task (relative disparity, absolute disparity).

Coarse stereopsis only

We also calculated the proportion of observers with coarse stereopsis only for each task. We define an observer with coarse stereopsis only as an observer with a threshold larger (worse) than 900 arcsec, which is the diplopia limit (Palmer, 1961). The “coarse stereopsis only” proportion was different between the two disparity tasks (81% vs. 38.1%; χ2 = 11.57, p < 0.001) and was higher for the absolute disparity task compared with the relative disparity task (Figure 8).

Perceptual learning

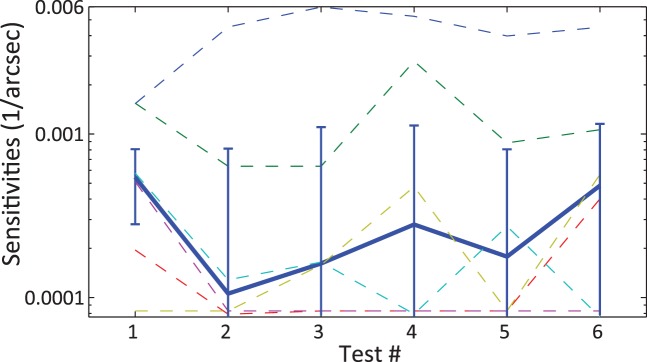
With the exception of one observer (with the best starting sensitivity), we found no evidence for learning over the six blocks of repeated testing on the absolute disparity task with a reference at 5 arcmin (Figure 9). Sensitivities (inverse of raw thresholds) were not significantly different across test repeats: Friedman's analysis of variance, χ2(5, 25) = 4.45, p = 0.49; repeated measures analysis of variance, F(5, 25) = 0.72, p = 0.62.

Figure 9.

Figure 9

Open in a new tab

Perceptual learning test: Stereo sensitivities as a function of test repetition. Each colored dashed line corresponds to a participant. The blue plain line is the median with standard error bars. No evidence of learning can be seen except for one participant out of six.

Discussion

We compared depth judgments for absolute and relative disparities when (a) equating the memory burden inherent in the two tasks, (b) measuring vergence noise under similar conditions, and (c) using a relatively large sample (N = 21) of nonexpert and naive observers. We took great care to measure absolute disparity thresholds under conditions that eliminate all relative disparity cues. In the past, when measuring absolute disparity acuity, the successive task almost always included landmarks that could have been used for computing a relative disparity with the object, such as a visible fixation point, or the screen edge (Cottereau, McKee, Ales, et al., 2012; McKee, Welch, et al., 1990; Westheimer, 1979). This casts doubt on the true absolute disparity acuity. An elegant study (Cottereau, McKee, Ales, et al., 2012) isolated absolute disparity acuities by having a center disk with a correlated random-dot stereogram and a surround with an uncorrelated random-dot stereogram. However, the screen edge theoretically still could have served as a landmark for judging relative rather than absolute disparities (McKee, Welch, et al., 1990; Parker, 2007).

Therefore, we removed all potential landmarks for the absolute disparity condition by extinguishing the fixation point and the fusion locks upon stimulus presentation, measuring acuity in a dark room, and having different screen-edge shapes between eyes so that the edges are in binocular rivalry and cannot serve as a binocular reference. Monocular cues were available, but their use was compromised by adding a large horizontal random jitter on each trial. One study (McKee, Welch, et al., 1990) compared depth estimates in the presence and absence of a fixation point using short presentations; however, they did not mask the screen edge or control for monocular cues. In their case, the relative disparity target was always on the right in the relative disparity condition, with no horizontal random jitter.

We found that, on average, acuity was approximately four times better for relative disparities than for absolute disparities. This falls within the large range reported in previous studies. For example, relative disparity acuity has been reported to be better than absolute disparity acuity by as much as a factor of 30 (Cottereau, McKee, Ales, et al., 2012) and as little as a factor of three (McKee, Welch, et al., 1990; Westheimer, 1979) in the most relevant conditions.

Importantly, vergence noise was too low to reconcile the difference between absolute and relative disparity thresholds with the feeding system hypothesis in its pure form (no anomaly). This is the main reason to exclude that option, but we emphasize that the pattern of correlations was also incompatible because of the absence of a strong correlation between absolute and relative disparity sensitivities and between absolute disparity and vergence sensitivities.

However, the pattern of correlations was also not compatible with the independent system hypothesis in its pure form (no anomaly): Vergence and absolute disparity thresholds were uncorrelated, whereas vergence and relative disparity thresholds were correlated. It is difficult to theorize a direct link between vergence and relative disparity that could explain this correlation. It would make little sense for an efficient cognitive agent to base vergence on relative disparities or to use vergence noise in the calculation of relative disparities. It is more likely that vergence is based on absolute disparities and that relative disparities are also computed from absolute disparities. Indeed, the pattern of correlation can be explained by the joint existence of the absolute disparity anomaly and the hypothesis of the feeding systems, as discussed below.

We found that vergence noise was not correlated with absolute disparity thresholds (Figure 7). This observation is paradoxical given that vergence relies on absolute disparities and, therefore, the two should be correlated. We also uncovered a significant correlation between vergence noise and relative disparity thresholds. These two findings are difficult, if not impossible, to interpret without postulating the presence of the absolute disparity anomaly—that is, an absence of conscious readout of absolute disparities for judging depth (Table 1)—conjointly with the feeding system hypothesis. Specifically, we hypothesize that the true absolute disparity threshold is actually lower than the vergence noise and that the vergence system can access that absolute disparity information (Figure 7). However, the observer cannot consciously access the disparity information for judging depth when in an absolute disparity format but rather only when in a relative disparity format (either directly or calculated from the absolute disparities). The absolute disparity anomaly explains why the absolute disparity thresholds are higher than the relative ones despite the fact that the relative disparity system is fed by the absolute disparities. Further evidence for the absolute disparity anomaly includes the fact that the proportion of observers with stereo impairment or no fine stereopsis was much higher in the absolute disparity task than in the relative disparity task. Assuming that absolute disparities feed the relative disparity system predicts the correlation between relative disparity thresholds and vergence noise but not the correlation between absolute disparity thresholds and vergence noise because of the absolute disparity anomaly. This pattern of results cannot be explained by the hypothesis of independent systems.

It is important to note that our interpretation of the correlation pattern (absolute disparity anomaly and existence of feeding systems) might appear to be based partially on the absence of two correlations (correlation between relative disparity thresholds and absolute disparity thresholds and correlation between vergence noise and absolute disparity thresholds). Absences of correlation are controversial to interpret, especially given the number of participants, which is small by correlation study standards (Wilmer, 2008). However, we emphasize that our arguments are based solely on the presence of significant correlations and differences. Specifically, the presence of the significant relationship between vergence and relative disparity acuities is difficult to reconcile with the hypothesis of independent systems, while the sign of the difference between absolute disparity and relative disparity thresholds and the low vergence noise excludes the simple version of the hypothesis of feeding systems (feeding systems in the absence of the anomaly; Table 1). Thus, we are left to conclude that the most parsimonious explanation for the pattern of results is that relative disparity is calculated from the difference of absolute disparities and that there is an absolute disparity anomaly.

One may ask whether our pattern of data could be accounted for by the hypothesis of independent systems under the absolute disparity anomaly. Although we cannot firmly rule out this conjecture, it seems unlikely. First, a relatively strong relationship between absolute disparity and relative disparity acuities is needed to produce the indirect correlation between relative disparity and vergence thresholds that we measured. The former correlation could occur on the basis that the two systems share some common visual inputs, but it would then be a residual correlation. A residual correlation is unlikely to drive a strong indirect correlation of r = 0.53. One could object that the correlation between the absolute and relative disparity sensitivities should be expected to be more than residual because participants have varying positional acuities and visual acuities, which are limiting factors for good stereo acuities. However, we attempted to reduce this limiting variability by selecting only participants with visual acuity better than 20/20 in both eyes. We have not tested positional sensitivity because positional acuity is not the limiting factor of stereo acuity for participants with normal visual acuities (Westheimer & McKee, 1979). Furthermore, there is a trend in our data for a possible correlation between absolute disparity and relative disparity acuities (Spearman r = 0.35, p = 0.06; one tailed). Given that we had only 21 participants and that 23 participants are required to detect a correlation r = 0.5 at p = 0.05 (one tailed) 80% of the time (Wilmer, 2008), we may have found that correlation significant with more participants. Such a result would not be in line with an independent system view. Taken together, our pattern of results does not align well with the assumption that the two systems are only residually correlated but rather seems better captured under the assumption that the absolute system feeds the relative one in the presence of the absolute disparity anomaly.

Therefore, using a different approach, our study adds evidence to converging data supporting the feeding system hypothesis. These data include the study of Westheimer and McKee (1979), which compared binocular bisection with equivalent stereo discrimination, and another study (McKee, Welch, et al., 1990), which compared monocular small-interval discriminations with stereo discrimination. Comparing relative disparity acuities and positional acuities under similar conditions is theoretically a good way to address the question of relative disparity mechanisms. However, it is challenging to compare positional and stereo acuities directly. The first complication concerns the distance between the lines to compare: Should it be matched to the monocular distance between stereo lines or to the dichoptic distance between stereo lines (McKee, Welch, et al., 1990)? As noted by Howard and Rogers (2002, pp. 197–198), it is also unclear whether stereo thresholds should be compared with binocular (Westheimer & McKee, 1979), monocular (McKee, Levi, et al., 1990; McKee, Welch, et al., 1990), or dichoptic (McKee & Levi, 1987) positional thresholds.

We tested naive nonexpert observers because we believe that it is difficult to extend the conclusions from a small sample of expert psychophysical observers to the general population. As a result, our relative disparity thresholds are worse than previously reported (McKee, Levi, et al., 1990; Shortess & Krauskopf, 1961; Westheimer, 1979). However, the proportion of relative disparity stereo impairment was 14.2%, which is lower than previously found in the literature for brief presentations. For example, with a 200-ms presentation time, the proportion was reported to be greater than 30% (Patterson et al., 1995), and greater than 20% at 300 ms (Patterson et al., 1995; Tam & Stelmach, 1998). Nevertheless, it is difficult to compare those studies with ours because of the numerous differences in paradigms and stimuli. However, our low proportion (compared with the literature) of stereo impairment for relative disparities provides evidence that the higher stereoblind proportion for absolute disparities (compared with relative disparities) is not an artefact of our experimental procedures. Our estimate of vergence noise was also worse than previously estimated—McKee and Levi (1987) estimated approximately 45 arcsec using the Nonius line technique in highly trained observers—which reflects the absence of expertise of our observers. However, note that if we had measured lower vergence noise, it would have strengthened our conclusions.

It may be worth considering whether the absolute disparity anomaly could be explained by an artefact in the measurement of absolute disparities. We argue that it is unlikely for the following three reasons. First, the tasks for absolute and relative disparities were almost identical. In both conditions, two lines (one above and one below fixation) are presented. In the absolute condition, observers had to judge the depth distance of the lines to the fixation plane—a depth distance that averages to 5 arcmin in the first absolute disparity block. In the relative condition, one has to compare a line at a depth of approximately 5 arcmin with a line at a depth of approximately 10 arcmin, which is also a 5-arcmin average depth distance. The difference between the two tasks is therefore the presence of a 5-arcmin pedestal (depth distance between the fixation plane and the line closest to fixation plane) in the relative disparity condition. The pedestal actually played against our result because increasing the pedestal to 5 arcmin increases thresholds (Badcock & Schor, 1985; Blakemore, 1970; McKee, Welch, et al., 1990; Siderov & Harwerth, 1995). While one study using gratings (Farell et al., 2004) found a small dip at a 5-arcmin pedestal in the thresholds as a function of pedestal, the effect seems to be specific to gratings and is not evident with lines (Westheimer, 1979).

Second, it could be argued that the single stimulus method was too difficult for the participants. However, the method has often been tested and been shown to result in acuities similar to those found with methods using an explicit reference for many tasks, even with naive participants (Morgan et al., 2000; Ross & Burr, 2010). Indeed, for line length estimation, performance was sometimes better with the method of single stimuli than with two-interval forced choices, with young and even elderly adults (Norman, Holmin, & Bartholomew, 2011). Another study (Morgan et al., 2000) suggested that observers average only over the last 15 trials, which is the reason we began each block of trials with 15 practice trials. To ensure that our observers could make optimal use of the depth information, we also provided feedback, although it has not been proven to give better results (Norman et al., 2011). Even if we assume that the single stimulus method was more challenging for the observers than the usual successive or simultaneous tasks, it cannot account for the difference between the absolute and the relative disparity conditions.

Third, it has been shown that observers have a very steep perceptual learning curve for the first few hundreds of trials (Fahle, Edelman, & Poggio, 1995), particularly when starting a task involving seeing depth in random-dot stereograms (Fendick & Westheimer, 1983; Sagi, 2011; Westheimer & Truong, 1988). However, at least in part, the very rapid learning can be interpreted as procedural learning (i.e., the participants are learning the procedures involved in the task) rather than perceptual learning. To be sure that our participants were not just slower to learn the task for the absolute disparity condition, five retests for that condition were administered to six observers, and all but one participant failed to learn.

Conclusions

In this study, we controlled for four potential issues in the long-standing debate over the relationship between absolute and relative disparities: unequal task memory load inherent to the task, high vergence noise, the use of landmarks, and the inclusion of expert observers only. We found that the fourfold difference between absolute and relative disparity thresholds is unlikely to be due to high vergence noise, lack of experience, or unequal memory load. We attribute this difference to an absence of conscious readout of absolute disparities for judging depth: the absolute disparity anomaly. Accordingly, the pattern of correlations between vergence noise, absolute disparity thresholds, and relative disparity thresholds can be explained by the joint existence of feeding systems and the absolute disparity anomaly. Altogether, our data suggest that relative disparity information is extracted from absolute disparities.

Given that the visual system is capable of extracting absolute disparity information for computing vergence, why would it discard it for judging depth? We discard absolute disparities for depth because they are corrupted by vergence noise, whereas relative disparities are not, independently of how they are calculated.

Supplementary Material

Supplement 1
Click here for additional data file. (18.9KB, docx)

Acknowledgments

We dedicate this work to David Knill, who passed away during the preparation of the article. We also acknowledge Pedro Cardoso, Simon Barthelmé, and Indu Vedamurthy for their help and Chloé de Senarclens, Suzanne McKee, Martin Banks, Marina Zannoli, Clifton Schor, Julie Harris, Ben Backus, and Anu Devi for their very helpful advice on this project. This work was supported by grants from the NEI (RO1EY020976 to Dennis Levi, Daphne Bavelier, and Dave Knill) and the Swiss National Foundation (100014_140676 to Daphne Bavelier).

Commercial relationships: none.

Corresponding author: Adrien Chopin.

Email: adrien.chopin@gmail.com.

Address: École Normale Supérieure, Département d'Études Cognitives, Paris, France.

Contributor Information

Adrien Chopin, adrien.chopin@gmail.com, http://adrien-chopin.weebly.com.

Dennis Levi, Email: dlevi@berkeley.edu.

David Knill, Email: david.knill@rochester.edu.

Daphne Bavelier, Email: Daphne.Bavelier@unige.ch.

References

  1. Badcock D. R.,, Schor C. M. (1985). Depth-increment detection function for individual spatial channels. Journal of the Optical Society of America, 2, 1211–1215. [DOI] [PubMed] [Google Scholar]
  2. Barthelmé S.,, Mamassian P. (2008). A flexible Bayesian method for adaptive measurement in psychophysics (Application). Retrieved from http://arxiv.org/abs/0809.0387
  3. Berry R. N. (1948). Quantitative relations among vernier, real depth, and stereoscopic depth acuities. Journal of Experimental Psychology, 38, 708–721. [DOI] [PubMed] [Google Scholar]
  4. Blakemore C. (1970). The range and scope of binocular depth discrimination in man. The Journal of Physiology, 211, 599–622. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Caziot B.,, Backus B. T. (2015). Stereoscopic offset makes objects easier to recognize. PLoS One, 10 (6), e0129101, doi:10.1371/journal.pone.0129101. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Cottereau B. R.,, McKee S. P.,, Ales J. M.,, Norcia A. M. (2012). Disparity-specific spatial interactions: Evidence from EEG source imaging. The Journal of Neuroscience, 32, 826–840, doi:10.1523/JNEUROSCI.2709-11.2012. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Cottereau B. R.,, McKee S. P.,, Norcia A. M. (2012). Bridging the gap: Global disparity processing in the human visual cortex. Journal of Neurophysiology, 107, 2421–2429. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Cumming B. G.,, Parker A. J. (1997). Responses of primary visual cortical neurons to binocular disparity without depth perception. Nature, 389, 280–283, doi:10.1038/38487. [DOI] [PubMed] [Google Scholar]
  9. Cumming B. G.,, Parker A. J. (1999). Binocular neurons in V1 of awake monkeys are selective for absolute, not relative, disparity. The Journal of Neuroscience, 19, 5602–5618. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. DeAngelis G. C. (2000). Seeing in three dimensions: The neurophysiology of stereopsis. Trends in Cognitive Sciences, 4, 80–90, doi:10.1016/S1364-6613(99)01443-6. [DOI] [PubMed] [Google Scholar]
  11. Erkelens C. J.,, Collewijn H. (1985a). Eye movements and stereopsis during dichoptic viewing of moving random-dot stereograms. Vision Research, 25, 1689–1700, doi:10.1016/0042-6989(85)90141-5. [DOI] [PubMed] [Google Scholar]
  12. Erkelens C. J.,, Collewijn H. (1985b). Motion perception during dichoptic viewing of moving random-dot stereograms. Vision Research, 25, 583–588, doi:10.1016/0042-6989(85)90164-6. [DOI] [PubMed] [Google Scholar]
  13. Fahle M.,, Edelman S.,, Poggio T. (1995). Fast perceptual learning in hyperacuity. Vision Research, 35, 3003–3013, doi:10.1016/0042-6989(95)00044-Z. [DOI] [PubMed] [Google Scholar]
  14. Farell B.,, Li S.,, McKee S. P. (2004). Disparity increment thresholds for gratings. Journal of Vision, 4 (3): 2 156–168, doi:10.1167/4.3.3. [PubMed] [Article] [DOI] [PubMed] [Google Scholar]
  15. Fendick M.,, Westheimer G. (1983). Effects of practice and the separation of test targets on foveal and peripheral stereoacuity. Vision Research, 23, 145–150, doi:10.1016/0042-6989(83)90137-2. [DOI] [PubMed] [Google Scholar]
  16. Georgeson M. A.,, Freeman T. C. A.,, Scott-Samuel N. E. (1996). Sub-pixel accuracy: Psychophysical validation of an algorithm for fine positioning and movement of dots on visual displays. Vision Research, 36, 605–612. [DOI] [PubMed] [Google Scholar]
  17. Heron S.,, Lages M. (2012). Screening and sampling in studies of binocular vision. Vision Research, 62, 228–234, doi:10.1016/j.visres.2012.04.012. [DOI] [PubMed] [Google Scholar]
  18. Howard I. P.,, Rogers B. J. (2002). Seeing in depth: Vol. 2. Depth perception. Toronto, Canada: I Porteous. [Google Scholar]
  19. Howard I. P.,, Rogers B. J. (2012a). Perceiving in depth: Vol. 2. Stereoscopic vision. New York, NY: Oxford University Press. [Google Scholar]
  20. Howard I. P.,, Rogers B. J. (2012b). Perceiving in depth: Vol. 3: Other mechanisms of depth perception. New York, NY: Oxford University Press. [Google Scholar]
  21. Julesz B. (1971). Foundations of cyclopean perception. Chicago, IL: University of Chicago Press. [Google Scholar]
  22. Kesten H. (1958). Accelerated stochastic approximation. The Annals of Mathematical Statistics, 29, 41–59. [Google Scholar]
  23. Mamassian P.,, Knill D. C.,, Kersten D. (1998). The perception of cast shadows. Trends in Cognitive Science, 2, 288–295. [DOI] [PubMed] [Google Scholar]
  24. Masson G. S.,, Busettini C.,, Miles F. A. (1997). Vergence eye movements in response to binocular disparity without depth perception. Nature, 389, 283–286, doi:10.1038/38496. [DOI] [PubMed] [Google Scholar]
  25. McKee S. P.,, Levi D. M. (1987). Dichoptic hyperacuity: The precision of Nonius alignment. Journal of the Optical Society of America, 4, 1104–1108. [DOI] [PubMed] [Google Scholar]
  26. McKee S. P.,, Levi D. M.,, Bowne S. F. (1990). The imprecision of stereopsis. Vision Research, 30, 1763–1779, doi:10.1016/0042-6989(90)90158-H. [DOI] [PubMed] [Google Scholar]
  27. McKee S. P.,, Taylor D. G. (2010). The precision of binocular and monocular depth judgments in natural settings. Journal of Vision, 10 (10): 2 1–13, doi:10.1167/10.10.5. [PubMed] [Article] [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. McKee S. P.,, Welch L.,, Taylor D. G.,, Bowne S. F. (1990). Finding the common bond: Stereoacuity and the other hyperacuities. Vision Research, 30, 879–891. [DOI] [PubMed] [Google Scholar]
  29. Morgan M. J. (1992). On the scaling of size judgements by orientational cues. Vision Research, 32, 1433–1445. [DOI] [PubMed] [Google Scholar]
  30. Morgan M. J.,, Watamaniuk S. N. J.,, McKee S. P. (2000). The use of an implicit standard for measuring discrimination thresholds. Vision Research, 40, 2341–2349. [DOI] [PubMed] [Google Scholar]
  31. Nefs H. T.,, O'Hare L.,, Harris J. M. (2010). Two independent mechanisms for motion-in-depth perception: Evidence from individual differences. Frontiers in Psychology, 1, 155, doi:10.3389/fpsyg.2010.00155. [DOI] [PMC free article] [PubMed] [Google Scholar]
  32. Neri P.,, Bridge H.,, Heeger D. J. (2004). Stereoscopic processing of absolute and relative disparity in human visual cortex. Journal of Neurophysiology, 92, 1880–1891. [DOI] [PubMed] [Google Scholar]
  33. Norman J. F.,, Holmin J. S.,, Bartholomew A. N. (2011). Visual memories for perceived length are well preserved in older adults. Vision Research, 51, 2057–2062, doi:10.1016/j.visres.2011.07.022. [DOI] [PubMed] [Google Scholar]
  34. Palmer D. A. (1961). Measurement of the horizontal extent of Panum's area by a method of constant stimuli. International Journal of Optics, 8, 151–159, doi:10.1080/713826374. [Google Scholar]
  35. Parker A. J. (2007). Binocular depth perception and the cerebral cortex. Nature Reviews Neuroscience, 8, 379–391. [DOI] [PubMed] [Google Scholar]
  36. Patel S. S.,, Bedell H. E.,, Tsang D. K.,, Ukwade M. T. (2009). Relationship between threshold and suprathreshold perception of position and stereoscopic depth. Journal of the Optical Society of America A, 26, 847–861, doi:10.1364/JOSAA.26.000847. [DOI] [PMC free article] [PubMed] [Google Scholar]
  37. Patterson R.,, Cayko R.,, Short G. L.,, Flanagan R.,, Moe L.,, Taylor E.,, Day P. (1995). Temporal integration differences between crossed and uncrossed stereoscopic mechanisms. Perception & Psychophysics, 57, 891–897, doi:10.3758/BF03206803. [DOI] [PubMed] [Google Scholar]
  38. Patterson R.,, Fox R. (1984). The effect of testing method on stereoanomaly. Vision Research, 24, 403–408, doi:10.1016/0042-6989(84)90038-5. [DOI] [PubMed] [Google Scholar]
  39. Prince S. J. D.,, Pointon A. D.,, Cumming B. G.,, Parker A. J. (2000). The precision of single neuron responses in cortical area V1 during stereoscopic depth judgments. Journal of Neuroscience, 20, 3387–3400. [DOI] [PMC free article] [PubMed] [Google Scholar]
  40. Regan D.,, Erkelens C. J.,, Collewijn H. (1986). Necessary conditions for the perception of motion in depth. Investigative Ophthalmology and Visual Science, 27, 584–597. [PubMed] [Article] [PubMed] [Google Scholar]
  41. Roe A. W.,, Parker A. J.,, Born R. T.,, DeAngelis G. C. (2007). Disparity channels in early vision. The Journal of Neuroscience, 27, 11820–11831, doi:10.1523/JNEUROSCI.4164-07.2007. [DOI] [PMC free article] [PubMed] [Google Scholar]
  42. Ross J.,, Burr D. C. (2010). Vision senses number directly. Journal of Vision, 10 (2): 2 1–8, doi:10.1167/10.2.10. [PubMed] [Article] [DOI] [PubMed] [Google Scholar]
  43. Sagi D. (2011). Perceptual learning in Vision Research. Vision Research, 51, 1552–1566, doi:10.1016/j.visres.2010.10.019. [DOI] [PubMed] [Google Scholar]
  44. Schor C. M. (2000). Binocular vision. DeValois K. K. (Ed.) Seeing: Handbook of perception and cognition (pp 177–258). San Diego, CA: Academic Press. [Google Scholar]
  45. Shortess, G. K.,, Krauskopf J. (1961). Role of involuntary eye movements in stereoscopic acuity. Journal of the Optical Society of America, 51, 555–559. [Google Scholar]
  46. Siderov J.,, Harwerth R. S. (1995). Stereopsis, spatial frequency and retinal eccentricity. Vision Research, 35, 2329–2337, doi:10.1016/0042-6989(94)00307-8. [DOI] [PubMed] [Google Scholar]
  47. Stevenson S. B.,, Lott L. A.,, Yang J. (1997). The influence of subject instruction on horizontal and vertical vergence tracking. Vision Research, 37, 2891–2898, doi:10.1016/S0042-6989(97)00109-0. [DOI] [PubMed] [Google Scholar]
  48. Stigmar G. (1970). Observations on vernier and stereo acuity with special reference to their relationship. Acta Ophthalmologica, 48, 979–998. [DOI] [PubMed] [Google Scholar]
  49. Stratton G. M. (1900). A new determination of the minimum visible and its bearing on localization and binocular depth. Psychological Review, 7, 429–435, doi:10.1037/h0064590. [Google Scholar]
  50. Tam W. J.,, Stelmach L. B. (1998). Display duration and stereoscopic depth discrimination. Canadian Journal of Experimental Psychology, 52, 56–61. [DOI] [PubMed] [Google Scholar]
  51. Thomas O. M.,, Cumming B. G.,, Parker A. J. (2002). A specialization for relative disparity in V2. Nature Neuroscience, 5, 472–478, doi:10.1038/nn837. [DOI] [PubMed] [Google Scholar]
  52. Walls G. L. (1943). Factors in human visual resolution. Journal of the Optical Society of America, 33, 487–503, doi:10.1364/JOSA.33.000487. [Google Scholar]
  53. Westheimer G. (1979). Cooperative neural processes involved in stereoscopic acuity. Experimental Brain Research, 36, 585–597. [DOI] [PubMed] [Google Scholar]
  54. Westheimer G.,, McKee S. P. (1977). Integration regions for visual hyperacuity. Vision Research, 17, 89–93. [DOI] [PubMed] [Google Scholar]
  55. Westheimer G.,, McKee S. P. (1979). What prior uniocular processing is necessary for stereopsis? Investigate Ophthalmology and Visual Science, 18, 614–621. [PubMed] [Article] [PubMed] [Google Scholar]
  56. Westheimer G.,, Truong T. T. (1988). Target crowding in foveal and peripheral stereoacuity. American Journal of Optometry and Physiological Optics, 65, 395–399. [DOI] [PubMed] [Google Scholar]
  57. Wheatstone C. (1838). Contributions to the physiology of vision. Part the first. On some remarkable, and hitherto unobserved, phenomena of binocular vision. Philosophical Transactions of the Royal Society of London, 128, 371–394. [Google Scholar]
  58. Wichmann F. A.,, Hill N. J. (2001). The psychometric function: I. Fitting, sampling, and goodness of fit. Perception & Psychophysics, 63, 1293–1313, doi:10.3758/BF03194544. [DOI] [PubMed] [Google Scholar]
  59. Wilmer J. B. (2008). How to use individual differences to isolate functional organization, biology, and utility of visual functions: With illustrative proposals for stereopsis. Spatial Vision, 21, 561–579, doi:10.1163/156856808786451408. [DOI] [PMC free article] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

i1534-7362-16-8-2-icon.jpg (26.8KB, jpg)
Supplement 1
Click here for additional data file. (18.9KB, docx)

Articles from Journal of Vision are provided here courtesy of Association for Research in Vision and Ophthalmology

RESOURCES