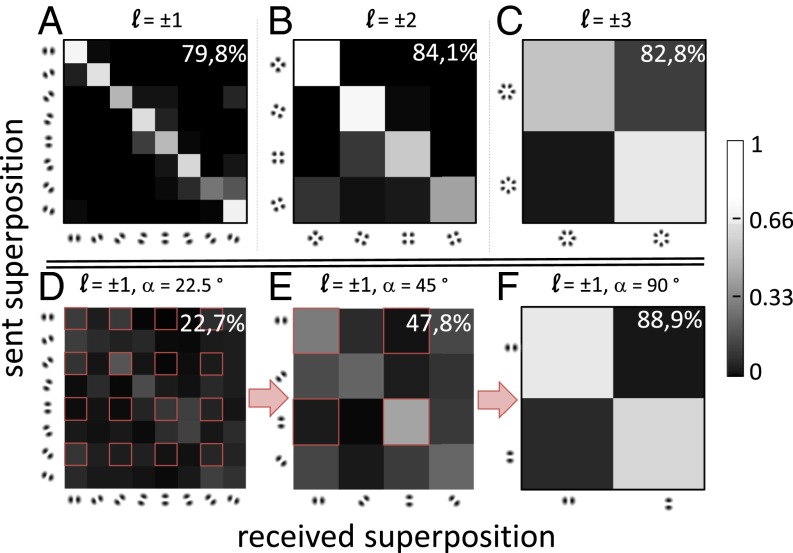
Fig. 3.

Cross-talk matrices showing the success probability with which the transmitted OAM mode superpositions were correctly identified at the receiver. (A–C) Results for superpositions of ℓ = ±1, ±2, and ±3 modes with relative phases of $\mathrm{\Delta }\alpha =\pi /4,\hspace{0.17em}\pi /2,\hspace{0.17em}\text{and}\hspace{0.17em}\pi$, respectively. The different relative phases correspond to different rotations of the superposition structure. The received modes were correctly identified by our detection algorithm with an average success probability of 82%. (D) During a turbulent night, eight modes consisting of superpositions of ℓ = ±1 were identified with a success probability of only 22.7%. When we restrict ourselves to a subset of these modes with $\mathrm{\Delta }\alpha =\pi /2$ or $\pi$ (highlighted with red squares and reanalyzed by the neural network each time), the success probability increases significantly (E and F). The ability to resolve all eight of these modes is required for device-independent quantum key distribution (violation of a Bell inequality), four modes are necessary for entanglement-based quantum key distribution (violation of an entanglement witness), and two modes can be used for classical communication with 1 bit per mode.