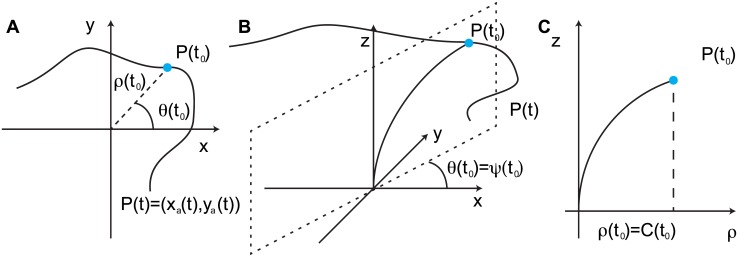
Fig 5.

A. The trajectory of the apical tip projected onto the (x, y) plane produces a parametric curve P(t) = (x_a(t), y_a(t)). Under the assumptions H1–H3, the position of the apical projection P(t), gives information on the amplitude and the direction of the plane of curvature, in polar coordinates (ρ(t), θ(t₀)). B. At a given time t₀ the organ is considered to be curved with a constant curvature C(t₀) along the organ in a single plane defined by the angle ψ(t₀) = θ(t₀). The measurement of θ(t), the angle of the projected apical curve, is then equivalent to ψ_c(t) C. Inside the plane perpendicular to the horizontal plane that contains the organ, the curvature can be measured directly as the value of ρ(t₀) (see S1 Text section 2–4 for a mathematical justification and a description of the approximations).