Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2017 Jan 12;7:40643. doi: 10.1038/srep40643

Micro-Structured Two-Component 3D Metamaterials with Negative Thermal-Expansion Coefficient from Positive Constituents

Jingyuan Qu 1,2, Muamer Kadic 1,2,a, Andreas Naber 1, Martin Wegener 1,2
PMCID: PMC5228033  PMID: 28079161

Abstract

Controlling the thermal expansion of materials is of great technological importance. Uncontrolled thermal expansion can lead to failure or irreversible destruction of structures and devices. In ordinary crystals, thermal expansion is governed by the asymmetry of the microscopic binding potential, which cannot be adjusted easily. In artificial crystals called metamaterials, thermal expansion can be controlled by structure. Here, following previous theoretical work, we fabricate three-dimensional (3D) two-component polymer micro-lattices by using gray-tone laser lithography. We perform cross-correlation analysis of optical microscopy images taken at different sample temperatures. The derived displacement-vector field reveals that the thermal expansion and resulting bending of the bi-material beams leads to a rotation of the 3D chiral crosses arranged onto a 3D checkerboard pattern within one metamaterial unit cell. These rotations can compensate the expansion of the all positive constituents, leading to an effectively near-zero thermal length-expansion coefficient, or over-compensate the expansion, leading to an effectively negative thermal length-expansion coefficient. This evidences a striking level of thermal-expansion control.

Three-dimensional (3D) printing of materials is a huge trend. It allows for individualizing products and for fabricating architectures that are very difficult if not impossible to make otherwise. Ultimately, one would like to 3D print any functional structure or device at the push of a button. Apart from boosting spatial resolution and printing speed, achieving this goal requires the ability to obtain hundreds or thousands of different material properties with one 3D printer. Today’s 2D graphical printers realize thousands of colors from only three cartridges (cyan, magenta, yellow). By analogy, future 3D material printers might be able to print thousands of different effective materials from only a few constituent-material “cartridges”.

Physics is on our side: Upon 3D printing two constituent materials A and B to obtain a composite or metamaterial, one might naively think that its effective properties will always be in between those of A and B. Fortunately, this is not the case1,2,3,4. In some cases, the behavior is even conceptually unbounded, i.e., an effective material parameter can assume any value from minus infinity to plus infinity even if those of the constituents are all finite and, e.g., positive. Examples are the electric permittivity and the magnetic permeability in electromagnetism or the compressibility and the mass density in mechanics5,6,7,8,9,10,11,12,13. However, for the mentioned examples, sign reversal and unbounded effective parameters are only possible near resonances at finite frequency and not in the truly static regime for reasons of stability in mechanics and non-negative energy density in electromagnetism5,13.

Static examples are rare. Theoretically, the thermal length-expansion coefficient and the Hall coefficient have been discussed1,2,3,14,15,16,17,18,19,20,21. Regarding the Hall coefficient, even one constituent material A and voids within suffice20. The situation is distinct for the thermal length-expansion coefficient. Within the range of validity of the continuum approximation, any connected structure composed of one constituent material A and voids within will show exactly the same thermal length-expansion coefficient as the bulk constituent material A. In contrast, the work of Lakes and others has shown that the behavior of composites containing two components A and B plus voids within is principally unbounded1. These two-dimensional structures were reviewed in Milton’s textbook2. Concrete blueprints for three-dimensional structures showing isotropic behavior were suggested later14. Refinements and two-dimensional macroscopic model structures composed of bimetallic beams were published as well15,16,17,18,22. Discussed theoretically a related two-dimensional structure composed of bimetallic strips showing a negative effective compressibility (at fixed temperature).

In regard to applications, thermal length-expansion is a small effect with huge consequences. A relative thermal length-expansion around 10−4 to 10−3 can lead to severe misalignment, failure, or cracks. Atomic-scale composites can provide near-zero or negative thermal-length expansion by changing the microscopic binding potential23,24,25. More macroscopic composites with near-zero length expansion are based on one constituent material with positive and another one with negative thermal expansion. For example, CERAN® glass cooking fields are made like that and have led to considerable markets.

Results

In this work, by using 3D gray-tone two-photon laser lithography, we fabricate micro-structured two-component metamaterials using a single photoresist, leading to an effectively negative thermal length-expansion coefficient from all-positive constituents. Applying image cross-correlation analysis, we directly measure the temperature-induced displacement-vector field in different layers of the micro-lattice with sub-pixel precision and thereby visualize the underlying microscopic mechanism.

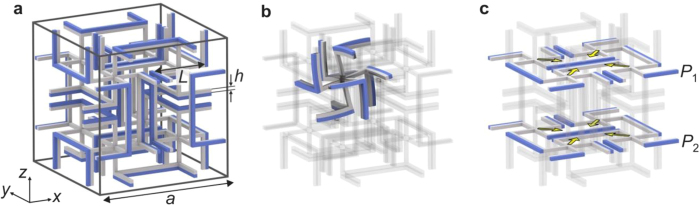
We have considered different blueprints from the literature14,16. For implementation using 3D laser printing, it is of utmost importance that the structure is robust against variations of structural and material parameters. Based on this consideration and on preliminary tests, we have focused our experimental work on one approach16. Figure 1a exhibits a single lattice constant of the micro-lattice blueprint we start from. This unit cell is placed onto a three-dimensional simple-cubic translational lattice. Apart from minor modifications, this blueprint has been taken from the literature16. The two components A and B shown in different colors have different positive thermal length-expansion coefficients. A mathematical discussion closely following ref. 1 is given in Supplementary Fig. S1. Intuitively, the operation principle is as follows (see Fig. 1b): The bi-material beams expand and bend upon heating. The bending leads to a rotation of the 3D crosses, the arms of which make them chiral. The chirality and hence the sense of rotation alternates between clockwise and counter-clockwise from one 3D cross to its neighbors, forming a 3D checkerboard pattern. The rotations counteract the length expansion of the beams. The amplitude of the rotations can be adjusted by the ratio of the thermal length-expansion coefficients of the constituent materials A and B. On this basis, one can tune the effective metamaterial thermal length-expansion coefficient (see Supplementary Figs S1 and S3). For the geometrical parameters used, the sign of the effective metamaterial thermal length-expansion coefficient changes sign at around 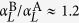Inline graphic. The absolute values of the Young’s moduli of A and B do not influence the effective metamaterial thermal length-expansion coefficient at all. The ratio of the Young’s moduli, i.e., EA/EB, does have an influence. However, even when changing this ratio by factors, the overall qualitative behavior remains the same (see Supplementary Figs S1 and S3). If component B is left away, i.e., replaced by vacuum, the effective thermal length-expansion coefficient of the micro-lattice is identical to that of bulk A. The same is true if B equals A.

Figure 1. Illustration of the micro-lattice blueprint underlying our experiments.

Figure 1

Open in a new tab

(a) One unit cell of the three-dimensional lattice composed of two different constituent materials. The one shown in gray has a smaller positive thermal length-expansion coefficient than the one shown in blue. This unit cell is repeated on a simple-cubic translation lattice with lattice constant a. The distance between the 3D crosses is a/2. All beams have quadratic cross section with width h. Geometrical parameters: a = 100 μm, h = 2.5 μm, and L = 40 μm. (b) In one eighth of the unit cell, the calculated structure is exhibited for an increased temperature, assuming a linear response. For clarity, all changes are largely exaggerated. One obtains an expansion and bending of the bi-material beams. The bending leads to a rotation of the three-dimensional cross, resulting in an inward movement of this cross (negative thermal length-expansion coefficient). The other seven eighths of the unit cell behave as mirror images (with respect to the three principal Cartesian planes) of the eighth shown. (c) Same as panel a, but two planes P1 and P2 parallel to the xy-plane cutting through the three-dimensional crosses are highlighted. These planes are imaged by optical microscopy in the experiments shown in Fig. 3.

We mention in passing that the structure shown in Fig. 1a has an effective Poisson’s ratio of v = −0.41. However, in general, the sign of the thermal-length expansion coefficient is not necessarily the same as the sign of the Poisson’s ratio15.

3D laser printing

How can we fabricate such complex 3D two-component metamaterials? Three-dimensional two-photon laser printing of a single polymer component is a well-established technology. Here, we use a commercial instrument (Photonics Professional, Nanoscribe GmbH). While characterizing the thermal length-expansion coefficients of various polymers in bulk cuboid form, we noticed that the thermal length-expansion coefficient does not only depend on the type of monomer we start from. For a given monomer (we use IP-Dip, Nanoscribe GmbH), it also depends on the light exposure dose during photo-polymerization. For example, when writing a bulk cube at a power scaling factor (see Methods) of 65% at a scan speed of 2 cm/s, we find a thermal length-expansion coefficient of αL = (+5 ± 0.5) × 10−5 K−1. At a power scaling factor of 35% and the same speed, this value increases to αL = (+8 ± 0.5) × 10−5 K−1. In between, the behavior is approximately linear (see Fig. S2). The measurement procedure shall be described below. We interpret this behavior in that the exposure dose influences the polymer cross-linking density. A correlation between cross-linking density and thermal expansion has been established previously26. The bottom line of this finding is that we can realize two different components A and B plus voids within by using only a single photoresist and gray-tone optical lithography.

For the temperature-dependent measurements, the samples are fixed to a Peltier-element heater within a small encapsulated chamber. In this fashion, the air within the chamber is heated as well, such that, after a certain waiting time (we chose several hours here), we can safely assume that the polymer micro-lattice temperature is actually equal to the temperature measured by a calibrated thermo-resistor at the Peltier-element location (as well as by a second one at the upper end of the cell). The chamber has a glass window to allow for optical access via a home-built wide-field optical microscope. It is based on a single microscope lens (Zeiss LD Achroplan 20× with a numerical aperture NA = 0.4) which images a sample plane directly onto the chip of a silicon-based charge-coupled-device (CCD) camera. We illuminate the sample by diffuse white light, for which we obtain the best image contrast for our micro-lattices. The entire chamber can be moved with respect to the microscope by using a 3D piezoelectric translation stage (Piezosystem Jena 1469), which is controlled by a computer.

Cross-correlation analysis

How can we directly characterize the thermal length-expansion and the operation principle underlying the micro-lattices? For example, for temperature differences on the order of ΔT = 20 K, we expect relative length changes of the samples on the order of |ΔL/L| = 10−3. If one images the entire sample onto a camera chip with 1000 pixels in one direction, this relative change corresponds to a movement of merely 1 pixel. Obviously, the measurement accuracy must be yet better than that. Image cross-correlation analysis can provide such sub-pixel sensitivity27. Our analysis, which is illustrated in Fig. S4a, starts from two optical images of a sample taken at two different temperatures. The images can refer to the sample’s surface. For transparent samples, they can alternatively correspond to a plane below the surface within the volume of the sample (e.g., plane P1 or P2 in Fig. 1c). We take a first image, the reference Iref(x, y), at room temperature. A second image is taken at a temperature elevated by ΔT with respect to room temperature. In this image, regions of interest (ROI) are defined. These small regions (we choose 33 × 33 pixels) can be centered around characteristic points (x, y) of the sample, e.g., onto the lattice points of a micro-lattice (compare Fig. 1c). Outside of the ROI, the image points are set to zero, leading to the function IROI(x, y). Next, the two-dimensional cross-correlation function C(Δx, Δy) of the reference image and the ROI is computed, i.e.,

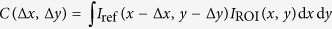graphic file with name srep40643-m2.jpg

we normalize according to C(x, y) → C(x, y)/C(0, 0). If both images are identical, the cross correlation peaks at displacement vector (0, 0); if they are shifted with respect to each other, it peaks at the corresponding displacement vector 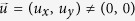Inline graphic. Thus, by identifying the position of the maximum of the cross-correlation function in two dimensions, the local displacement vector can be identified. We average over the results obtained from ten different images at the elevated temperature. By repeating the procedure for many ROI at different coordinates (x, y) in the image, we obtain the displacement-vector field 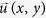Inline graphic. Figure S4b shows the result for a copper surface. The derived average length-expansion coefficient of αL = (+1.6 ± 0.1) × 10−5 K−1 is consistent with the literature value of αL = +1.65 × 10−5 K−1 within the error bars. Generally, upon changing the temperature of the sample and its holder, the sample as a whole can also move or drift across the image and/or through the focal plane of the microscope. The former effect can be eliminated by subtracting the average displacement vector of all ROI, i.e., 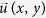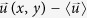Inline graphic → Inline graphic. This subtraction is unproblematic if the camera chip is perfectly homogeneous. To avoid possible artifacts from inhomogeneities, we rather compensate most of this average displacement by the piezoelectric translation stage, such that the sample’s image on the camera chip does not change with respect to the camera chip. Remaining small average shifts are subtracted in the processing of the images. Likewise, we compensate a possible thermally induced defocus (i.e., movement in z-direction) by an autofocus function. It is based on acquiring images while moving the sample along the z-axis using the piezoelectric translation stage. The sharpest image is selected in the post-processing.

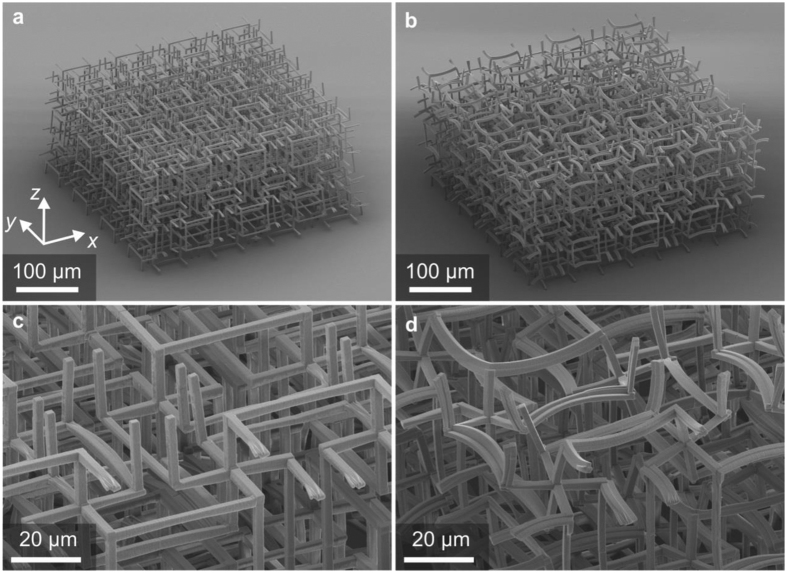
Electron micrographs of selected fabricated samples on glass substrates are shown in Fig. 2. The structure in Fig. 2b is composed of two different components, corresponding to different exposure doses during the printing process (see above). Note that the beams are bent, whereas they are straight for the control sample (depicted in Fig. 2a), in which exclusively the first component has been written. The bending originates from the different cross-linking densities resulting from different exposure doses, leading to different levels of volume shrinkage of the constituents during development. Notably, this shrinkage leads to an overall expansion of the metamaterial sample – in agreement with the operation principle28. This pre-bending also directly evidences that the two components actually have different properties. Within the linear regime, the pre-bending does not change the operation principle compared to that of the blueprint (compare Fig. 1b). The samples shown in Fig. 2 contain 4 × 4 × 2 = 32 complex three-dimensional unit cells following the blueprint shown in Fig. 1a.

Figure 2. Electron micrographs of three-dimensional polymer micro-lattices fabricated by gray-tone laser lithography.

Figure 2

Open in a new tab

These follow the blueprint shown in Fig. 1a and contain 4 × 4 × 2 unit cells. (a) Control sample composed of only a single constituent component (gray in Fig. 1a). (b) Two-component sample as shown in Fig. 1a. The different shrinkage of the two components during development leads to a pre-bending of the bi-material beams. Within the linear regime, this pre-bending does not change the operation principle. Conveniently, it directly evidences that the properties of the two components are actually different. (c,d) Are magnified views of the samples shown in panels a and b, respectively.

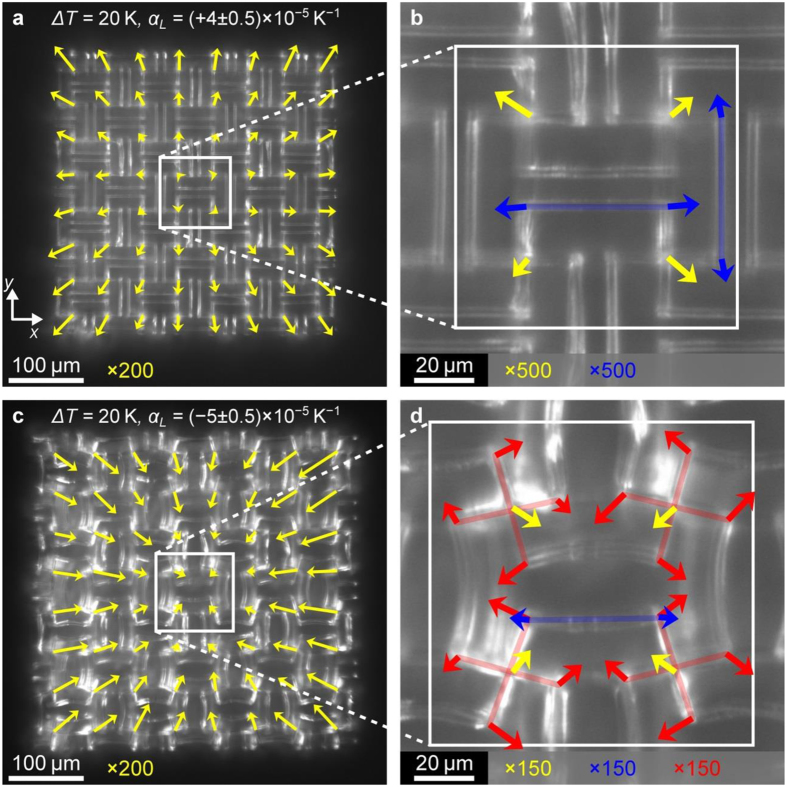
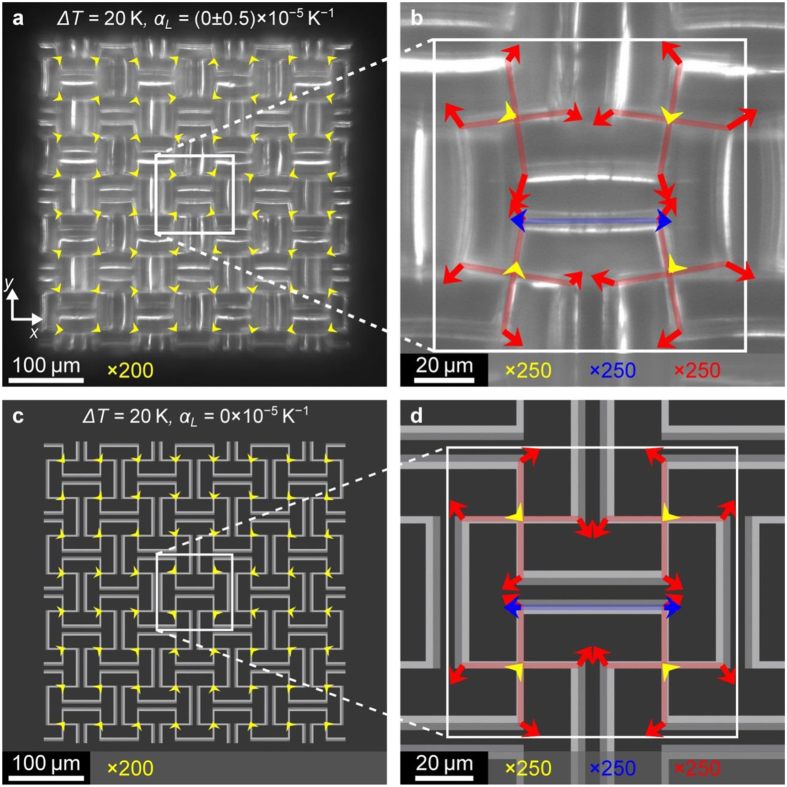
Figure 3 summarizes measurements of the displacement-vector field induced by a temperature rise of ΔT = 20 K with respect to room temperature. The background shows the room-temperature optical image as recorded by the CCD camera, corresponding to plane P1 in Fig. 1c. The yellow arrows are the displacement vectors at the 3D crossing points. To make them visible, the length of the arrows has been multiplied by a common factor with respect to the optical image as indicated. Obviously, all yellow arrows in Fig. 3c roughly point towards the center of the sample. Furthermore, the length of the arrows roughly increases linearly from the center to the sides. These two observations indicate a nearly homogeneous and isotropic behavior of the 3D crossing points. It is thus meaningful to describe the metamaterial by an effective thermal length-expansion coefficient. Upon reducing the temperature difference to ΔT = 10 K, we find essentially the same behavior as for ΔT = 20 K, albeit with worse signal-to-noise ratio (not depicted). From these data at ΔT = 20 K, we extract an average effective metamaterial thermal length-expansion coefficient of αL = (−5 ± 0.5) × 10−5 K−1. The value of αL = (−5 ± 0.5) × 10−5 K−1 for ΔT = 10 K is the same. When imaging the next lower plane of crossing points (compare P2 in Fig. 1c), we again find essentially the same behavior (average αL = (−5 ± 0.5) × 10−5 K−1). In contrast, the single-component control sample depicted in Fig. 3a and b exhibits a positive value of αL = (+4 ± 0.5) × 10−5 K−1. All of the described aspects are reversible, i.e., we have observed them more than three times on a given sample. We have also seen them on more than five sets of samples. Our analysis also allows for quantifying deviations from ideal isotropy. We find that the modulus of the expansion coefficient is 25% larger along the y-direction as compared to the x-direction for the two-component sample. This anisotropy is assigned to remaining fabrication imperfections. In particular, due to drift during writing, some beam connections in the micro-lattice may actually be missing.

Figure 3. Experimental results on negative thermal expansion.

Figure 3

Open in a new tab

Temperature-induced displacement-vector fields as measured directly by image-correlation analysis based on a room-temperature optical-microscope image (background) and images at a temperature increased by ΔT = 20 K as indicated. For clarity, the lengths of the shown displacement vectors are multiplied by factors as indicated. The image plane P1 is illustrated in Fig. 1c. The samples contain 4 × 4 × 2 unit cells. (a) Control sample as shown in Fig. 2a. From the yellow displacement vectors, we derive an average thermal length-expansion coefficient of αL = (+4 ± 0.5) × 10−5 K−1. (b) Magnified view of panel a. The blue vectors herein highlight the expansion of the beams. (c) Two-component sample (compare Figs 1a and 2b). From the yellow displacement vectors, we derive an average thermal length-expansion coefficient of αL = (−5 ± 0.5) × 10−5 K−1. (d) Magnified view of panel c. The blue vectors highlight the expansion of the beams. Their bending leads to the rotations of the crosses as apparent from the red displacement vectors. The sense of rotation changes between clockwise and counter-clockwise in a checkerboard pattern, in agreement with the anticipated operation principle (compare Fig. 1b). The semi-transparent red and blue lines in panels b and d are guides to the eye.

To further investigate whether the samples actually follow the behavior anticipated by the design as discussed above, we also track other points within the unit cell (compare plane P1 in Fig. 1c). The blue arrows in Fig. 3d show that the connecting beams actually expand upon heating. Here, for clarity, their average displacement vector has been subtracted. A similar behavior is found for all other beams as well (not depicted). The red arrows reveal the anticipated rotation of the crosses, with alternating clockwise and counter-clockwise sense of rotation. Again, for clarity, the average displacement vector for each cross has been subtracted from the corresponding set of four arrows. Note that the red rotation vectors are generally longer than the blue ones. As explained above, this behavior leads to the negative thermal length-expansion: The shrinkage induced by the rotations over-compensates the expansions of the individual beams. For the control sample in Fig. 3b, no significant rotations are found within the noise (hence the red arrows are not depicted here) and the thermal expansion is positive – as for the bulk polymer constituents discussed above. The measured behavior in Fig. 3 is in good overall agreement with the calculated one shown in Fig. 4. Finally, Fig. 5 shows results for 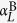Inline graphic closer to 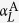Inline graphic, for which the rotations merely compensate the expansion of the beams, leading to effectively near-zero thermal length-expansion coefficient. Again, experiment and theory agree.

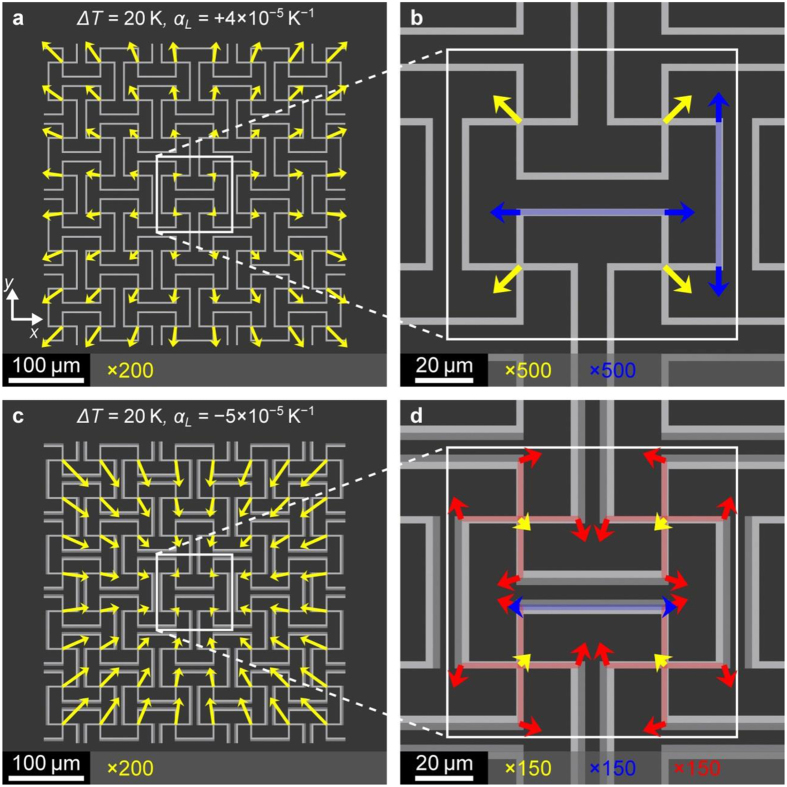
Figure 4. Numerical results on negative thermal expansion.

Figure 4

Open in a new tab

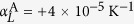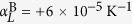Calculations corresponding to the experiments shown in Fig. 3, represented just like these experiments. Geometrical parameters (see Fig. 1a): a = 100 μm, h = 2.5 μm, and L = 40 μm. Material parameters: EA = 4 GPa, EB = 3 GPa, Inline graphic, and Inline graphic.

Figure 5. Near-zero thermal expansion.

Figure 5

Open in a new tab

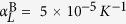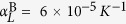(a,b) Experiment as in Fig. 3c and d, but for power scaling factors of 65% and 50% (instead of 65% and 38%) for the constituent materials a and b, respectively. The extracted average thermal length-expansion coefficient is αL = (0 ± 0.5) × 10−5 K−1. (c,d) Theory as in Fig. 4c and d, but for Inline graphic (instead of Inline graphic). All other parameters are the same. To make the rotations of the crosses visible, the indicated arrow stretching factors in panels b and d are ×250 instead of ×150 in Figs 3d and 4d.

In summary, we have fabricated and characterized micrometer-scale two-component polymer-based metamaterials exhibiting an effectively negative thermal length-expansion coefficient from positive constituents. The necessary two components plus voids have been realized by 3D gray-tone laser lithography using only a single photoresist.

Methods

Sample design

For the metamaterial design, a simple cubic unit cell was analyzed by a finite-element approach using the commercially available software package COMSOL Multiphysics and the MUMPS solver within. For the mechanical part of the problem, the thermal expansion was introduced as a volumetric stress. The constituent materials A and B were modeled with a Young’s modulus of EA = 4 GPa and EB = 3 GPa (actually only the ratio EA/EB enters into the effective thermal expansion/contraction, see Supplementary Figs S1 and S3), a Poisson’s ratio of vA = 0.4 (and vB = 0.4), and a thermal length-expansion coefficient of 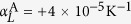Inline graphic throughout this manuscript. For the negative effective thermal length-expansion metamaterials shown in Figs 1 and 4 we used 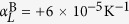Inline graphic. For the near-zero effective thermal length-expansion metamaterial shown in Fig. 5 we used 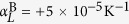Inline graphic. For the calculations shown in Figs 1b and S3, we used periodic boundary conditions29. To assess the influence of finite sample size, we also performed calculations for samples containing 4 × 4 × 2 unit cells fixed to a rigid substrate (Figs 4 and 5). Based on the two mirror planes cutting the structure through the middle, one parallel to the xz- and one parallel to the yz-plane, we reduced the computational domain to 2 × 2 × 2 unit cells fixed to a rigid substrate. The other sides are left free to move (stress-free boundary conditions). The derived effective thermal length-expansion coefficients were different from those for the periodic boundary conditions by only a few percent. Typically, each unit cell (compare Fig. 1a) was discretized into 5 × 105 tetrahedral elements.

Sample fabrication

The structures composed of 4 × 4 × 2 unit cells were written using a liquid photoresist (IP-Dip, Nanoscribe GmbH, Germany) and a commercial three-dimensional laser lithography system (Photonics Professional, Nanoscribe GmbH). The objective lens (63×, NA = 1.4, Carl Zeiss) was dipped directly into the photoresist. An average power of 50 mW measured at the backfocal aperture of the objective lens with a diameter of 7.3 mm was defined as the reference power. The actual power was given by this reference power times the power scaling factor, which was varied locally in the spirit of gray-tone lithography. The component with low (high) thermal length-expansion coefficient was written with a power scaling factor of 65% (38%). The line spacing in the x- and y-direction was 200 mm, that in the z-direction was 500 nm. Small parts were written in the galvo-scanning mode with a scan speed of 2 cm/s and stitched by using micro-positioning stages29. The exposed samples were developed in mr-Dev 600 for 60 minutes, transferred to acetone, followed by supercritical-point-drying in acetone.

Additional Information

How to cite this article: Qu, J. et al. Micro-Structured Two-Component 3D Metamaterials with Negative Thermal-Expansion Coefficient from Positive Constituents. Sci. Rep. 7, 40643; doi: 10.1038/srep40643 (2017).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Material

Supplementary Information
srep40643-s1.pdf (645.2KB, pdf)

Acknowledgments

We acknowledge discussions with Claudio Findeisen and Peter Gumbsch (KIT). We are grateful to Martin Schumann (KIT) for help regarding the electron micrographs. We thank the Hector Fellow Academy, the Helmholtz program Science and Technology of Nanosystems (STN), and the Karlsruhe School of Optics & Photonics (KSOP) for support. We acknowledge support by Deutsche Forschungsgemeinschaft and Open Access Publishing Fund of Karlsruhe Institute of Technology.

Footnotes

Author Contributions J.Q. has fabricated the samples and has performed the measurements and the data processing. M.K. has helped in the simulations and discussions. A.N. has helped in the discussions. M.W. has led the effort and has written the first draft of the paper. All authors have contributed to the final version of the manuscript.

References

  1. Lakes R. S. Cellular solid structures with unbounded thermal expansion. J. Mater. Sci. Lett. 15, 475–477 (1996). [Google Scholar]
  2. Milton G. W. The Theory of Composites, Cambridge Univ. Press, UK (2002). [Google Scholar]
  3. Milton G. W. Extending the Theory of Composites to Other Areas of Science, Milton-Patton Publishing, USA (2016). [Google Scholar]
  4. Giovampaola C. D. & Engheta N. Digital metamaterials. Nature Mater. 13, 1115–1121 (2014). [DOI] [PubMed] [Google Scholar]
  5. Smith D. R., Padilla W. J., Vier D. C., Nemat-Nasser S. C. & Schultz S. Composite Medium with Simultaneously Negative Permeability and Permittivity. Phys. Rev. Lett. 84, 4184–4187 (2000). [DOI] [PubMed] [Google Scholar]
  6. Soukoulis C. M., Linden S. & Wegener M. Negative refractive index at optical wavelengths. Science 315, 47–49 (2007). [DOI] [PubMed] [Google Scholar]
  7. Pendry J. B., Holden A. J., Robbins D. J. & Stewart W. J. Magnetism from conductors and enhanced nonlinear phenomena. IEEE Trans. Microwave Theory Tech. 47, 2075–2084 (1999). [Google Scholar]
  8. Liu Z., Zhang X., Mao Y., Zhu Y. Y., Yang Z., Chan C. T. & Sheng P. Locally resonant sonic materials. Science 289, 1734–1736 (2000). [DOI] [PubMed] [Google Scholar]
  9. Lakes R. S. & Wojciechowski K. W., Negative compressibility, negative Poisson’s ratio, and stability. Phys. Status Solidi B 245, 545–551 (2008). [Google Scholar]
  10. Nicolaou Z. G. & Motter A. E., Mechanical metamaterials with negative compressibility transitions. Nature Mater. 11, 608–613 (2012). [DOI] [PubMed] [Google Scholar]
  11. Huang H. H., Sun C. T. & Huang G. L. On the negative effective mass density in acoustic metamaterials. Int. J. Eng. Sci. 47, 610–617 (2009). [Google Scholar]
  12. Kadic M., Bückmann T., Schittny R. & Wegener M. Metamaterials beyond electromagnetism. Rep. Prog. Phys. 76, 126501 (2013). [DOI] [PubMed] [Google Scholar]
  13. Christensen J., Kadic M., Kraft O. & Wegener M. Vibrant times for mechanical metamaterials. MRS Commun. 5, 453–462 (2015). [Google Scholar]
  14. Lakes R. S. Solids with tunable positive or negative thermal expansion of unbounded magnitude. Appl. Phys. Lett. 90, 221905 (2007). [Google Scholar]
  15. Ha C. S., Hestekin E., Li J., Plesha M. E. & Lakes R. S. Controllable thermal expansion of large magnitude in chiral negative Poisson’s ratio lattices. Phys. Status Solidi B 252, 1431–1434 (2015). [Google Scholar]
  16. Wu L., Li B. & Zhou J. Isotropic Negative Thermal Expansion Metamaterials. ACS Appl. Mater. Interfaces 8, 17721–17727 (2016). [DOI] [PubMed] [Google Scholar]
  17. Grima J. N., Bajada M., Scerri S., Attard D., Dudek K. K. & Gatt R. Maximizing negative thermal expansion via rigid unit modes: a geometry-based approach. Proc. R. Soc. A 471, 20150188 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Hopkins J. B., Song Y., Leeb H., Fang N. X. & Spadaccini C. M. Polytope Sector-Based Synthesis and Analysis of Microstructural Architectures With Tunable Thermal Conductivity and Expansion. J. Mech. Des. 138, 051401 (2016). [Google Scholar]
  19. Briane M. & Milton G. W., Homogenization of the Three-Dimensional Hall Effect and Change of Sign of the Hall Coefficient. Arch. Ration. Mech. Anal. 193, 715–736 (2009). [Google Scholar]
  20. Kadic M., Schittny R., Bückmann T., Kern C. & Wegener M. Hall-effect sign-inversion in a realizable 3D metamaterial. Phys. Rev. X 5, 021030 (2015). [Google Scholar]
  21. Kern C., Kadic M. & Wegener M. Parallel Hall effect from three-dimensional single-component metamaterials. Appl. Phys. Lett. 107, 132103 (2015). [Google Scholar]
  22. Gatt R. & Grima J. N. Negative compressibility. Phys. Status Solidi RRL 2, 236–238 (2008). [Google Scholar]
  23. Miller K. J., Romao C. P., Bieringer M., Marinkovic B. A., Prisco L. & White M. A. Near-Zero Thermal Expansion in In(HfMg)0.5Mo3O12. J. Am. Ceram. Soc. 96, 561–566 (2013). [Google Scholar]
  24. Mary T. A., Evans J. S. O., Vogt T. & Sleight, Negative thermal expansion from 0.3 to 1050 K in ZrW2O8. Science 272, 90–92 (1996). [Google Scholar]
  25. Marinkovic B. A., Aria M., Mendes Jardima P., de Avilleza R. R., Rizzoa F. & Ferreira F. F. In2Mo3O12: A low negative thermal expansion compound. Thermochim. Acta 499, 48–53 (2010). [Google Scholar]
  26. Dietz J. E. & Peppas N. A. Reaction kinetics and chemical changes during polymerization of multifunctional (meth)acrylates for the production of highly crosslinked polymers used in information storage systems. Polymer 38, 3767–3781 (1997). [Google Scholar]
  27. Bückmann T., Stenger N., Kadic M., Kaschke J., Frölich A., Kennerknecht T., Eberl C., Thiel M. & Wegener M. Tailored 3D mechanical metamaterials made by dip-in direct-laser-writing optical lithography. Adv. Mater. 24, 2710–2714 (2012). [DOI] [PubMed] [Google Scholar]
  28. Liu J., Gu T., Shan S., Kang S. H., Weaver J. C. & Bertoldi K. Harnessing buckling to design architected materials that exhibit effective negative swelling. Adv. Mater. 28, 6619–6624 (2016). [DOI] [PubMed] [Google Scholar]
  29. Frenzel T., Findeisen C., Kadic M., Gumbsch P. & Wegener M. Tailored Buckling Microlattices as Reusable Light‐Weight Shock Absorbers. Adv. Mater. 28, 5865–5870 (2016). [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information
srep40643-s1.pdf (645.2KB, pdf)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES