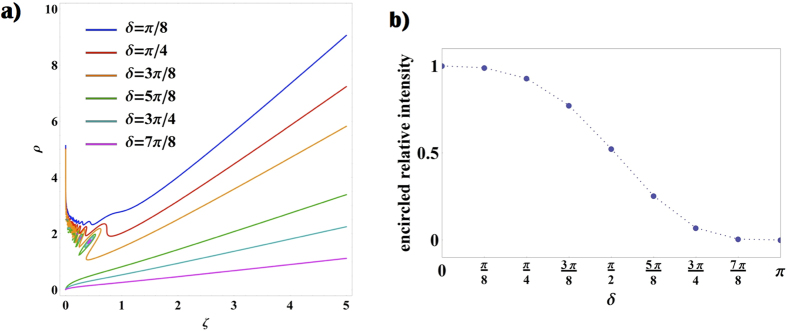
Figure 5. Dynamical evolution of C-points during propagation and associated enclosed energy.

(a) We plot the radial coordinate ρ of the position of left handed C-points vs the longitudinal coordinate ζ, for different values of δ (the latter are encoded in the color of the curve, as displayed in the figure legend). Two regimes can be distinguished: for π/2 < δ < π C-points merge at the beam center (ρ = 0) as ζ → 0, hence making the polarization pattern topology non-trivial. In the same regime, additional closed loops indicate the existence of C-point dipoles appearing and disappearing as the beam propagates, as a consequence of the dynamical evolution of the radial ripples characterizing HyGG modes. For 0 < δ < π/2, instead, the C-point radius increases indefinitely as we approach the beam near field. (b) For the same values of δ as in panel a, we plot here the fraction of the beam intensity contained in a circular region with a radius given by the C-point radial coordinate, in the far field limit. These results, in particular, are obtained when considering ζ = 15, but in the limit of large ζ, they remain essentially constant.