Figure 2.
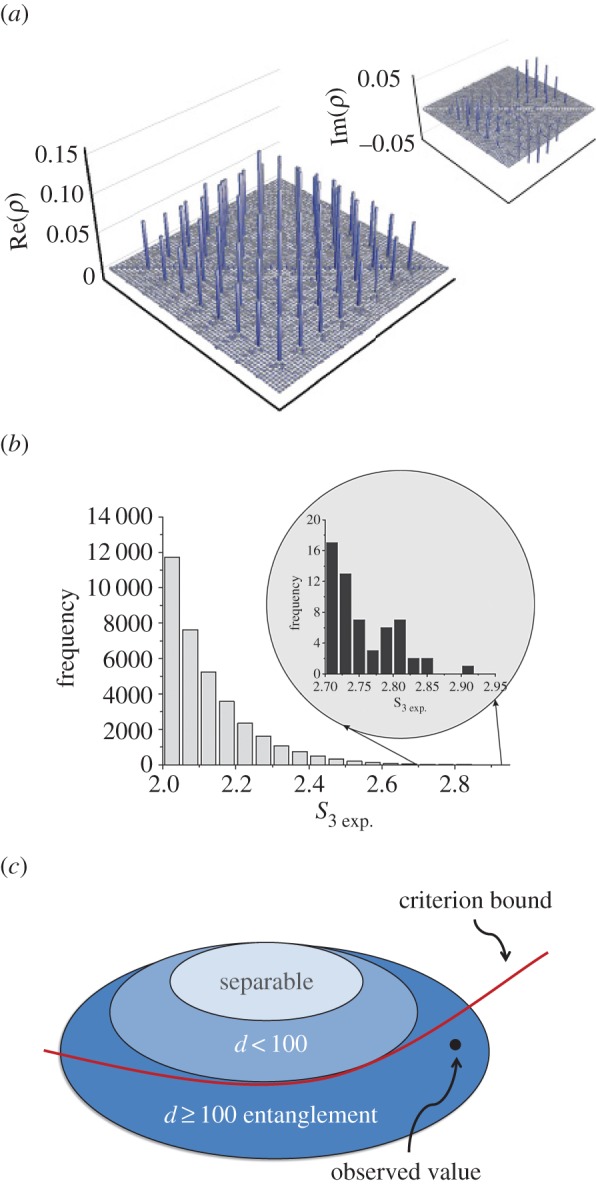
Three different methods to investigate high-dimensional entanglement. (a) Quantum state tomography, (b) violation of high-dimensional Bell inequalities, (c) entanglement dimensionality witnesses. (a) Quantum tomography, while experimentally and computationally expensive, gives the maximal possible information about the quantum state. The highest-dimensional two-photon state for which quantum tomography (without assumption of state properties) was reported is an eight-dimensionally entangled state. The figure shows the reconstructed density matrix (adapted from [24]). (b) Generalized Bell inequalities can also be used to verify high-dimensional entanglement. These values from [27] show the violation of a three-dimensional Bell inequality by exceeding the classical bound of S3 = 2 for various settings in the experiment. (c) An entanglement dimensionality (Schmidt Number) witness gives a set of measurements and bounds that a state with entanglement dimension d can maximally reach. If an experiment exceeds this bound, the state was at least (d + 1)-dimensionally entangled. In this image, a situation is depicted where a measurement leads to an observed value exceeding the bound for d = 100-dimensional entanglement (adapted from [28]). (Online version in colour.)
