Fig. 7.

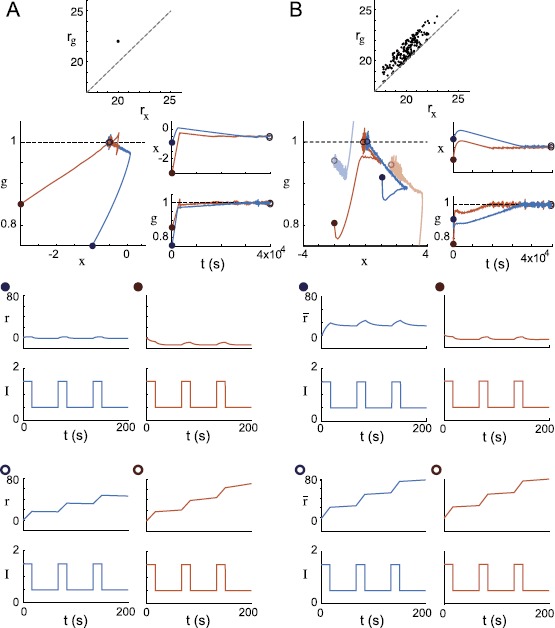
Dual homeostasis creates integrators from a single recurrently excitatory neuron and a heterogeneous excitatory network. (A) Dual homeostasis tunes a single neuron with a recurrent excitatory connection to function as an integrator from two different initial conditions (orange and blue). First row: the target rates $r_x$ and $r_g$ are plotted as a point in $(r_x,r_g)$ space. Second row: the resulting x and g trajectories are plotted in phase space and over time. Third row: before dual homeostasis, the neuron is tested for integrator-like behavior by injecting pulsatile input $I(t)$. The firing rate r returns to a baseline after each pulse. Fourth row: after dual homeostasis, firing rate r increases at each pulse and retains its approximate value from one pulse to the next. This neuron is an integrator: its firing rate at any time represents an integral of the pulse history. (B) Analogous plots for a heterogeneous recurrently excitatory network of 200 neurons initialized from two initial conditions (orange and blue). Top row: target firing rate pairs of all neurons are plotted in $(r_x,r_g)$ space. Note that $r_g$ is always chosen to be greater than $r_x$. Second row: average values of g and x across the network are plotted in phase space and over time. In phase space, a representative trajectory of a single neuron in the network for each of the two simulation runs is also plotted in lighter colors. Note that these trajectories are significantly removed from the average trajectories. Third row: both times the network is initialized, the average network rate r̅ does not act as an integrator for pulsatile input. Fourth row: after dual homeostasis, the average network rate acts as an integrator