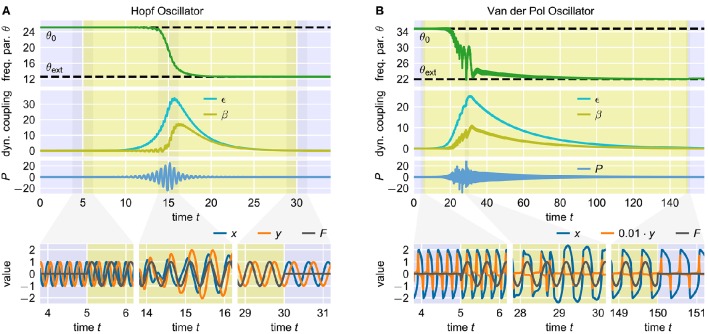
Figure 6.

Adaptation of two oscillators with the AFDC mechanism. The upper-most panels show the time course of the frequency determining parameter θ. The time during which the external signal is applied to the system is indicated by the yellow shaded area. The dashed horizontal lines indicate the initial value θ₀ and the value θ_ext corresponding to the exact frequency of the external signal. The second panels from the top show the time course of the adaptive coupling strengths β and ϵ. The third panels from the top show the time course of the filtered external signal P. The panels on the bottom show the oscillating state variables x and y and the external signal F at different short time windows during the adaptation process. In both cases, the initial intrinsic frequency of the oscillator is f₀ = 4.0 and the external signal is a sine wave with unit amplitude and frequency f_ext = 2.0. (A) Hopf oscillator with AFDC mechanism with μ = 1.0, η = 0.5, κ = 5.0, τ = 2.0, β₀ = 0.0 and ϵ₀ = 0.01. The initial value of the parameter θ is given by θ₀ = 2πf₀ ≈ 25.1, the value corresponding to the frequency of the external signal is θ_ext = 2πf_ext ≈ 12.6. The external signal is applied for 5 ≤ t < 30. (B) Van der Pol oscillator with AFDC mechanism with μ = 100.0, η = 2.0, κ = 5.0, τ = 15.0, β₀ = 0.0 and ϵ₀ = 0.01. The values of the parameter θ corresponding to f₀ and f_ext are determined to be θ₀ ≈ 34.8 and θ_ext ≈ 22.0 (see Methods). The external signal is applied for 5 ≤ t < 150.