Figure 6. Entropy and intervals example.

Let us indicate with the same letters the occurrences, e.g., of lyrics of the same artist in the sequence. Suppose that A has just appeared in the sequence, which ends with G. Thus, A appears 4 times, i.e., k = 4. We divide the subsequence 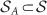in 4 parts and count the occurrences f_i of A in each of them (bottom numbers). The normalized entropy of A will be 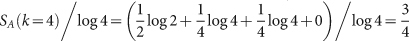. As a value of S(k) we average all entropies of the elements occurring k-times in 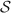. The numbers at the top show the length of the inter-times used in the interval distribution evaluation. The local reshuffling would shuffle only those 15 elements occurring after the first occurrence of A, and compute the normalized entropy and the time intervals distribution on this reduced sequence.