Fig 3. Results of Minimizing Network Energy Loss.

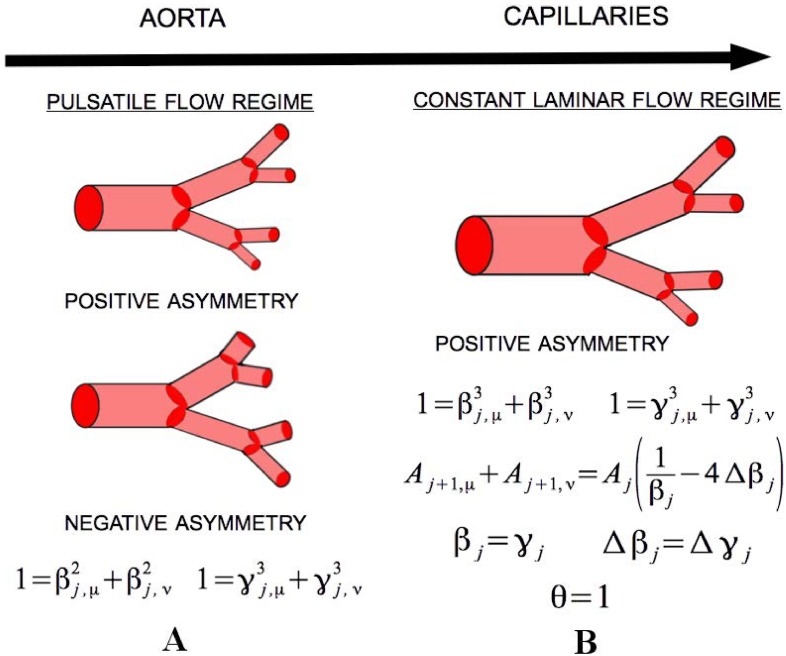
In the pulsatile flow regime (A) either network asymmetry type is allowed, but both must follow the area-preserving and space-filling principles at the nodal level, as per Eqs (16) and (17). In this regime switching between asymmetry types can occur both within and across generations. In the constant laminar flow regime (B) only positive network asymmetry is predicted, and the scale factors must follow the space-filling and Murray’s Law relations, as per Eqs (20) and (21). We also find that the asymmetric version of Murray’s Law allows for an expression of the total cross-sectional area, A, of any two child branches in terms of the parent area and the average and difference scale factors, showing that asymmetric branching allows for a toggling between branching with increasing area and constant area. Lastly, the average radius and length scale factors are predicted to be equal, as well as the difference radius and length scale factors. When combining these two results with the space-filling and Murray’s Law expressions, we can make a strict prediction of the metabolic scaling exponent θ = 1 for the constant laminar flow regime.