Figure - PMC

Skip to main content

An official website of the United States government

Here's how you know

Here's how you know

Official websites use .gov
A .gov website belongs to an official government organization in the United States.

Secure .gov websites use HTTPS
A lock ( ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

View full-text article in PMC

. 2017 May 16;11:56. doi: 10.1186/s12918-017-0430-4

Search in PMC
Search in PubMed
View in NLM Catalog
Add to search

© The Author(s) 2017

Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The Creative Commons Public Domain Dedication waiver (http://creativecommons.org/publicdomain/zero/1.0/) applies to the data made available in this article, unless otherwise stated.

PMC Copyright notice

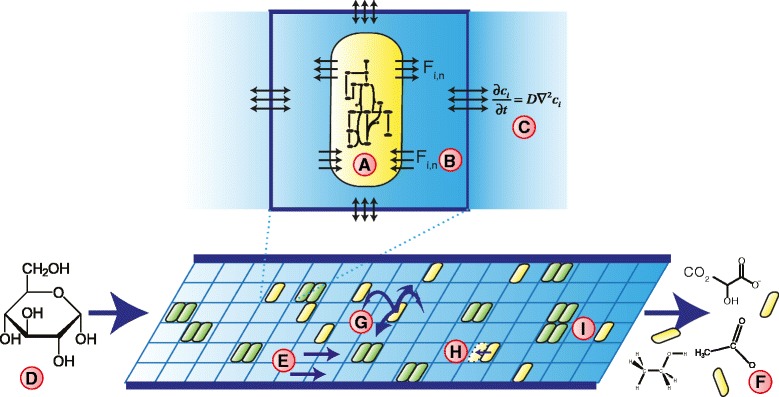
Fig. 3 — Setup of the simulation model of a metabolizing gut microbial community. The model represents a community of growing subpopulations of genetically identical bacteria. a The metabolism of each population is modeled using a unique, modified GEM of L. plantarum[28]; b Based on extracellular metabolite concentrations, the genome scale model predicts the growth rate (r) of the subpopulation and the influx and efflux rates of a subset of 115 metabolites. These are used as derivatives for a partial-differential equation model describing the concentrations of extracellular metabolites, $\partial c_{i} (\vec{x}, t) / ∂t = F_{i} (\vec{x}) + D \nabla^{2} c (\vec{x}, t)$ , where c the metabolites diffuse between adjacent grid sites, $\vec{x}$ . d The population is represented on a two-dimensional, tube-like structure, with periodic inputs of glucose. e To mimic advection of metabolites through the gut, the concentrations are periodically shifted to the right, until they f exit from the end of the tube. g The bacterial populations hop at random to adjacent grid sites; to mimic adherence to the gut wall mucus bacterial populations are not advected, unless indicated otherwise. h Once the subpopulation has grown to twice its original size, it divides into an empty spot in the same lattice size at which time the metabolic network is mutated. i Two subpopulations can live on one grid point; with yellow indicating presence of one subpopulation, and green indicating the presence of two subpopulations. (Structural formulas: Licensed under Public domain via Wikimedia Commons; “Alpha-D-Glucopyranose” by NEUROtiker, also licenced under public domain via Wikipedia Commons)