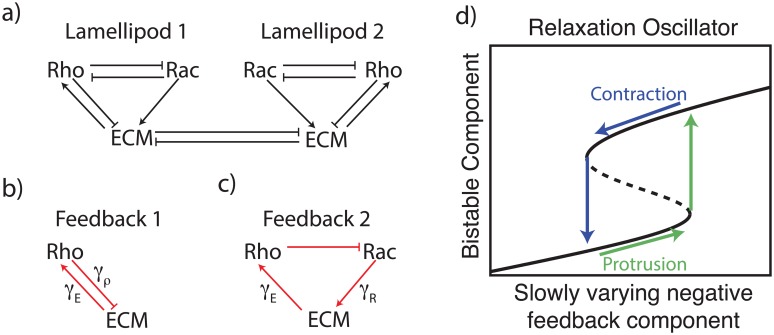
Fig 2. Model schematic.

(a) Schematic depiction of the proposed interactions between the GTPases and cell-ECM contact areas, which are proxies for extracellular matrix (ECM) signalling in each lamellipod (E_j). We assume that Rac (Rho) promotes protrusion (contraction), resulting in opposite effects: Rac (Rho) increases (decreases) the cell-ECM contact area and hence E_j. Mechanical constraints or other limitations couple the two lamellipods, preventing the cell-ECM contact areas from growing independently. This is modelled as a competition term coupling E₁ and E₂. (b,c) We consider two potential negative feedback loops that could lead to oscillations. Each arrow is labeled with the parameter governing the strength of its influence. (d) Basic idea of a relaxation oscillator: bistability is present in a subset of the model (black curve); other component(s) acting on a slower timescale provide negative feedback that sets up oscillations (green, blue curves). In this illustration, bistability results from two fold bifurcations as in the GTPase submodel, but the same idea applies when the bifurcations are both transcritical, as found in the ECM submodel (Fig 3d). Protrusion causes an increase in ECM signaling that leads to increased feedback, contraction has the opposing effect.