Fig. 5.

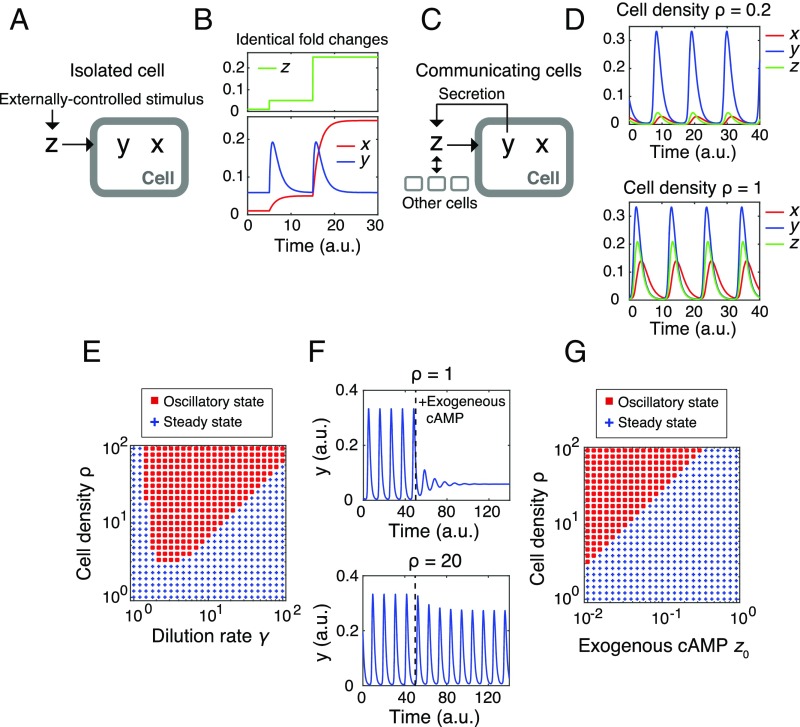
Density robustness is a basic property of a secrete-and-sense system consisting of FCD elements. (A and B) A reaction scheme (A, “Isolated Cell”) and the characteristic behaviors (B) of an FCD system. The time evolution of y and x are described by functions f and g that together constitutes a FCD system (A). Simulated time course of x and y (B, Lower) to incremental increase in z (B, Upper). (C and D) A Schematic diagram of a closed-loop secrete-and-sense system (C, “Communicating cells”) and results from model simulations (Eq. 3) for low (Upper) and high (Lower) cell densities. Here, x and y follow the same reaction scheme as in A except that y is secreted, and thus transformed to z to further stimulate the cells. (E) A phase diagram of the system (Eq. 3) with a lower detection limit. Oscillatory (red squares) and static [blue plus sign (+)] states. (F) Results from numerical simulations of a modified model (Eq. 3 with dz/dt = ρk_ty – γ(z − z₀)). (G) A phase diagram of the system (the model in D) as a function of cell density ρ and the exogenous cAMP level z₀. Red squares and blue plus signs (+) indicate oscillatory and static states, respectively. The parameters were τ = 1.5, n = 2, K = 4, k_t = 2, and γ = 3, unless otherwise indicated.