Figure 5.

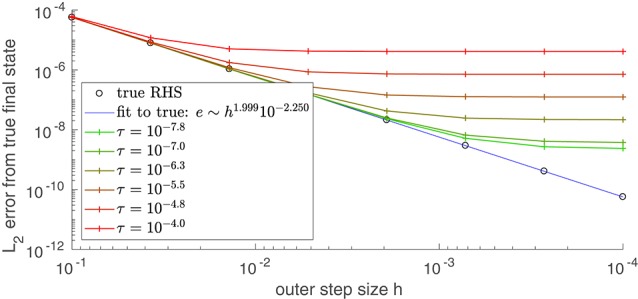
As the inner step size τ decreases, the error (compared to direct integration) of a projective (not coarse-projective) integration becomes bounded by the integrator's intrinsic (outer) step size h. The true solution at t = 0.417 was found by integrating using MATLAB's ode45 with an absolute tolerance of 10^−12.0 and a relative tolerance of 10^−12.0 The series of black circles give the error at t = 0.417 that results from using integration using the true RHS function (Equation 3) in an explicit second-order Runge-Kutta integration scheme of (outer) step size h. The colored curves use the same integrator and outer step size, but approximate the RHS function with the difference map f_τ(θ(t)) = θ(t)−Φ_{τ, F}[θ(t)], analogous to the coarse difference map of Equation (18). Error was evaluated by taking the norm of the vector difference between the projective integration solution θ(0.417) and the true solution. Compare this to Figure A1 in the Appendix, in which a similar analysis is performed on a system of two ODEs modeling a single reversible reaction.